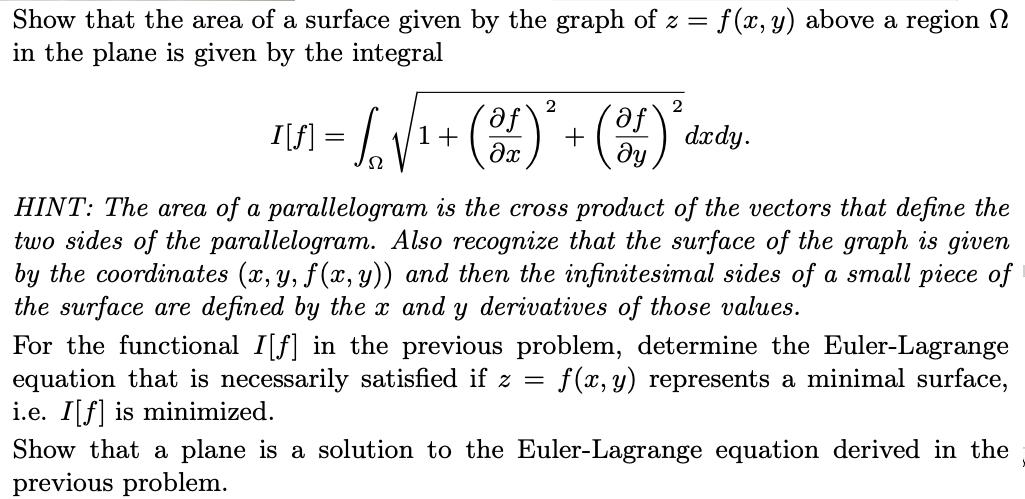
Show that the area of a surface given by the graph of z = f(x, y) above a region in the plane is given by the integral 2 = + (06) S2 I[f] = + 2 ddy. HINT: The area of a parallelogram is the cross product of the vectors that define the two sides of the parallelogram. Also recognize that the surface of the graph is given by the coordinates (x, y, f(x, y)) and then the infinitesimal sides of a small piece of the surface are defined by the x and y derivatives of those values. For the functional I[f] in the previous problem, determine the Euler-Lagrange equation that is necessarily satisfied if z = f(x, y) represents a minimal surface, i.e. I[f] is minimized. Show that a plane is a solution to the Euler-Lagrange equation derived in the previous problem.