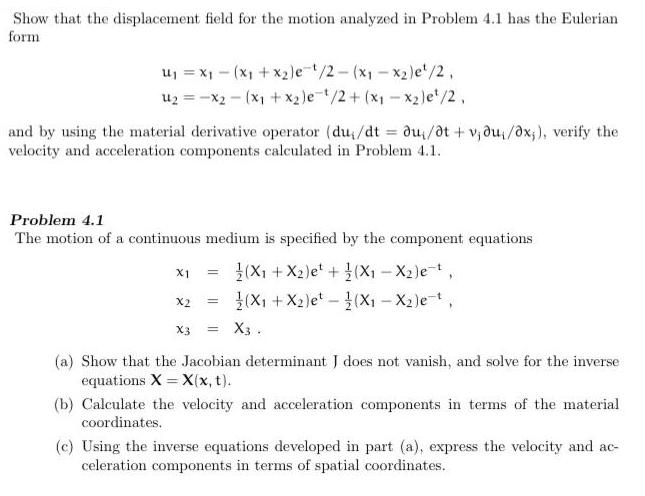
Show that the displacement field for the motion analyzed in Problem 4.1 has the Eulerian form uj = X1 - (X +x2le-/2-(x1-x2)e'/2, u2 = -X2 - (x + x2)et/2+(x1-x2)e'/2, and by using the material derivative operator (du/dt = du/at + v, du;/ax;), verify the velocity and acceleration components calculated in Problem 4.1. Problem 4.1 The motion of a continuous medium is specified by the component equations (X1 + X2)et + (X1-X2)e-t (X1 +X2)e-(X1- X2)e, X1 X2 !! X3 . X3 (a) Show that the Jacobian determinant J does not vanish, and solve for the inverse equations X = X(x, t). (b) Calculate the velocity and acceleration components in terms of the material coordinates. (c) Using the inverse equations developed in part (a), express the velocity and ac- celeration components in terms of spatial coordinates.