Show workings Kindly.

2. Suppose 42% of Ph.D.s in statistics/biostatistics are earned by woman and a random sample 36 statistics/iostatistics Ph.D.s is obtained.(You may want to use technology to compute some of the probabilities.)

a. What's the probability none of the 36 statistics/biostatistics Ph.D.s are women?

b. What's the probability at least 1 of the 36 statistics/biostatistics Lh.D.s is a woman?

c. How many woman do you expect in the sample of the 36 statistics/biostatistics Ph.D.s?

d. What's the probability fewer than half of hte 36 statistics/biostatistics Ph.D.s are women?

e. What's the probability fewer than half of the 36 statistics/biostatistics Ph.D.s are women if at least 12 are not women?

f. What's the probability the first woman is the 5th person in the sample?

g. What's the probability the first woman is the 15th person in the sample?

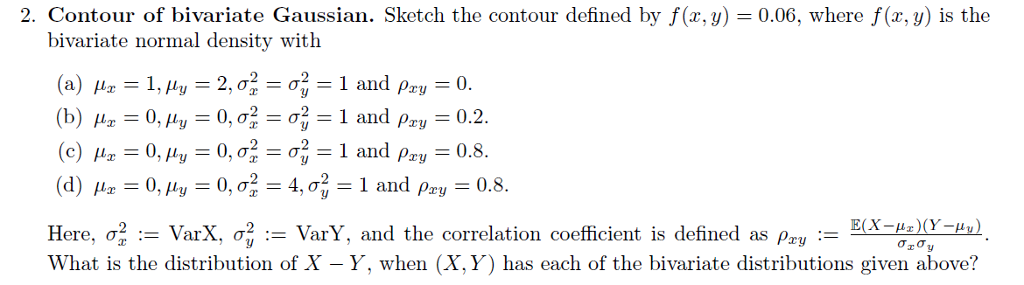
For some constant c > 0, X and Y are bivariate Gaussian random variables with joint PDF exp ( (2 3) /4 (x-3)(y-1)/3+ (y-1)2/4 fx, x (x, y) = C 87 5/9 Let W = 2X + Y and R = X + Y. Choose all the true statements. Keep in mind that the number of true statements is random; it's possible that all 10 statements are true . Note that (z) is the CDF of the Standard Normal Random Variable Z, and Q (z) is the Standard Normal Complementary CDF. P(2X + Y 6) = Q(2/V3). The correlation coefficient px y = 1/3. The correlation coefficient PW , R = 0.782. Y is Gaussian (1, 4). Var W] = 92/3. W and R are bivariate Gaussian random variables. Cov W, R = 20. The constant c = 5/9.and Y are bivariate Gaussian if their joint PDF is given by fx, y (x, y) = 2noxGY (1 - Pxy ) 1/2 exp X HX - HX MY 2(1 - PXY) - 2PXY OY ox OY This PDF is specified by 5 parameters which correspond to the marginal means, variances and correlation, i.e., (X, Y) ~ N(ux, MY , 6%, 67, PXY) Some interesting facts about bivariate Gaussians are listed below: . Marginals are Gaussian: X ~ N(ux, ox ) and X ~ N(ux, ox). . Bivariate Gaussian RVs are uncorrelated iff they are independent: If pxy = 0 then the joint density can be written as fx , y (x, y) = fx ( x ) fy ( v ) = - -e - ( x - ux ) 2 /208 1 -e- ( 1-MY ) 2/20%, V2nox V2TOY thus the RVs are independent. . A linear transformation of bivariate Gaussian is also bivariate Gaussian, but with new parameters. . Bivariate Gaussian and conditioning: For bivariate Gaussian RVs (X, Y) the conditional distributions fry and frix are also Gaussian, and are given by, frix ( y | x ) = JX, Y (x, y) (y - MY x (x))2 - exp fx (x) V2noYx ~ N ( MY X , OYIX ) , 20 Yx where E[Y | X] = Myx (X) = My + Pxy ox (X - ux ) and GYx = of (1 - PxY). Suppose that X ~ N(0, 1) and Y ~ N(1, 2) are two independent Gaussian random variables. Define random variables U and V as U = X +Y, V = X +aY for some a. 1. What is the joint distribution of U, V in terms of a? 2. Find a (if it exists) such that U and V be independent. 3. Find the conditional distribution fulv (u|5).2. Contour of bivariate Gaussian. Sketch the contour defined by f(x, y) = 0.06, where f(x, y) is the bivariate normal density with (a) Hr = 1, My = 2,02 = 03 = 1 and Pry = 0. (b) Hz = 0, My = 0, 02 = 02 = 1 and Pry = 0.2. (c) Hx = 0, My = 0,02 = 03 = 1 and Pxy = 0.8. (d) Ax = 0, My = 0, 02 = 4, 03 = 1 and Pry = 0.8. Here, o2 := VarX, o2 := VarY, and the correlation coefficient is defined as Pry := E(X -HI) ( Y-Hy) What is the distribution of X - Y, when (X, Y) has each of the bivariate distributions given above?How are your grades? In a recent semester at a local university, 3oo students a: enrolled in both Statistics I and Psychology 1. Of these students, as got an A 4') in statistics, is: got an a in psychology, and 44 got an A in both statistics and E: psychology. Round the answers to four decimal places, as needed. Q E1 d]: (a) Find the probability that a randomly chosen student got an A in E statistics or psychology or both. The probability that a randomly chosen student got an A in statistics or psychology or both is D. ms at: (b) Find the probability that a randomly chosen student did not get an A in statistics. The probability that a randomly chosen student did not get an A In statistics is D