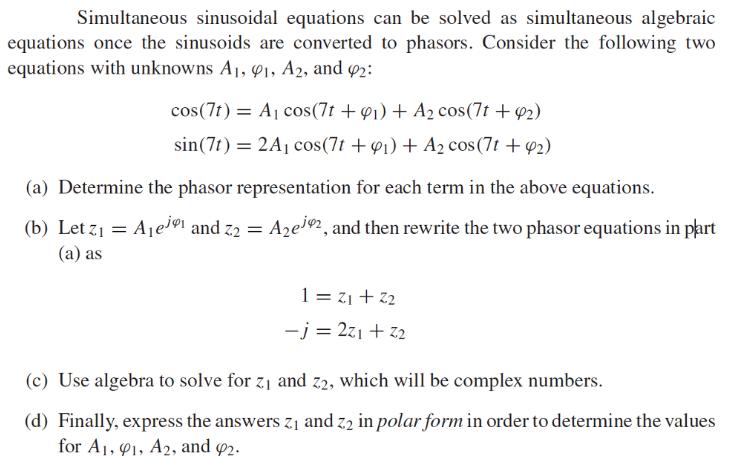
Simultaneous sinusoidal equations can be solved as simultaneous algebraic equations once the sinusoids are converted to phasors. Consider the following two equations with unknowns A1, 1, A2, and 2: cos(7t) = Aj cos(7t +e1) + A2 cos(7t +42) %3D sin(7t) = 2A1 cos(7t +y1) + A2 cos (7t +2) (a) Determine the phasor representation for each term in the above equations. (b) Let z1 = Ael1 and z2 = A2e1, and then rewrite the two phasor equations in part (a) as 1 = z1 + 22 -j = 2z1 + z2 (c) Use algebra to solve for z1 and z2, which will be complex numbers. (d) Finally, express the answers z1 and z2 in polar form in order to determine the values for A1, 1, A2, and 2.