slides that went with question

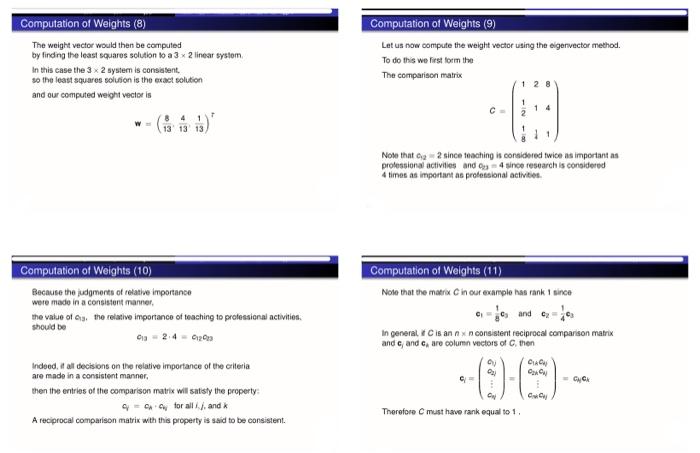
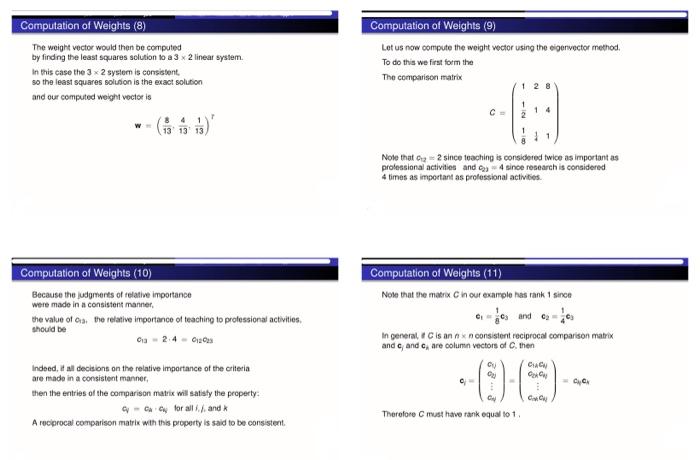
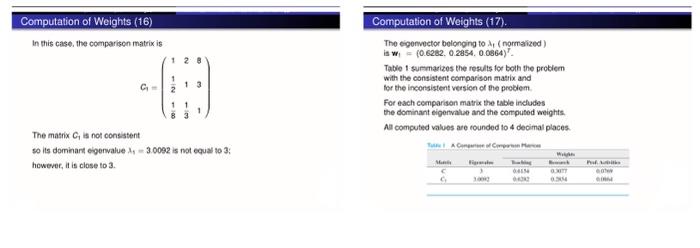
A management student received fellowship offers from four universities and now must choose which one to accept. The student uses the analytic hierarchy process to decide among the universities and bases the decision process on the following four criteria: (i) financial matters-tuition and scholarships (ii) the reputation of the university (iii) social life at the university (iv) geography-how desirable is the location of the university In order to weigh the criteria the student decides that finance and reputation are equally important and both are 4 times as important as social life and 6 times as important as geography. The student also rates social life twice as important as geography. (a) Determine a reciprocal comparison matrix C based on the given judgments of the relative importance of the 4 criteria. (b) Show that the matrix C is not consistent. (c) Make the problem consistent by changing the relative importance of one pair of criteria and determine a new comparison matrix C1 for the consistent problem. (d) Find an eigenvector belonging to the dominant eigenvalue of C1 and use it to determine a weight vector for the decision criteria. we considered an exanple imolving a search process to fill a full professor position at a laroe universty. In order to assign woights to the quasty of the reseacch of the four candidates. the committee did pairwise comparisona of the relative quality of the research publications of the candidates. Atter studying the puldications of all the candidates, the committee agreed upon the following pairwise comporisons of the woights: w1=1.75w2,w2=0.75w2w1=1.5w3,w1=1.25w4w2=0.50w4.w2=0.75w4 Here an equation such as w2=0.50w4 would indcase that the qualty of research from candidate 2 was only hall as strong as the qualty of research from candidate 4. Equivalently one could say that the qualty of research from candidase 4 is twice as strong as the quality of research from candidate 2 . Computation of Weights (2) Computation of Weights (3) the weights must all add up io 1 . Using this condition we were able to express w4 in terme of w1,w2, and w2. We then found the values of w1,w2, and w3 by calculating the loast aquares solution to a 6 : 3 linear syrtem. The calculaled weight vector was w4=(0.3289,0.1739,0.2188,0.2784)T. We now consider an alternative method for computing the weight voctor based on an eigonvectar cakculatien. To do this we first form a comparison matrix C. The (i,j) entry of C indicates how the quality of the research of candidate i compares to the quality of the research of candidate / Thus if, for example, w2=0.5w4. then cic=2 and ce=21. The weight vector would then be computed by finding the least squares solution to a 32 linear systom. In this case the 32 system is consiasent. so the least squares solision is the exact solution and our computed weight vector is w=(138,134,131)T Computation of Weights (10) Because the judgmerts of retative importance were made in a consistent manner. the value of Gis. the relastive importance of teaching to professienal activities. should be c13=24=c12cn Indeed, If al decisions on the retative importance of the criteria are made in a consistent manner, then the entries of the comparison matrix will satisly the property: =cn+cNforalli,andk A reciprocal comparison matrix with this property is said to be consistent. Let us now compute the weight vector using the eigenvector method. To do this we first form the The comparison matrix C=12181211841 Note that C2=2 since teaching is considered fwice as important as protessional activities and c2y=4 since research is considered 4 times as important as protessional activises. Computation of Welghts (11) Nole that the matr c in cur example has rank 1 since c1=81c3andc2=41c3 In generat, fC is an n n consistent reciprocal comparison matrix and cj and c are columen vectors of C,then c=cjc2jcvj=c1kcwc2kcvjcikcij=cjck Therefoe C must have rank equal to 1 . The weight vector would then be computed by finding the least squares solution to a 32 linear system. In this case the 32 system is consistent. so the least squares solusion is the exact solution and our computed weight vecter is w=(138,134,131)r Let us noe compute the weight vector using the eigenvector method. To do this we first form the The cemparison matrix C=121812141841 Nole that c2=2 since teaching is considered twice as important as prolessional activities and c2y=4 sinoe research is considered 4 times as important as prolestional activities. Computation of Weights (11) Nole that the matrx C in cur exarrole has rank 1 since c1=81c3andc2=41c3 In general, fC is an nB consistent reciprocal comparison matrox and cj and ci are columen vectors of C, then c=cvc2jcvj=c1ncvc2ncvcincv=cinck Therefoce C must have rank equal to 1. A reciprocal comparison matrix with this preperty is said to be consistent. It follows that 0 must be an eigervalue of C and the dimension of its eigenspace must be n1, the nullty of C. So 0 musi be an eigenvalue of multiplicily n1. The remaining eigervalue A4 must equal the trace of C. So 1=a is the dominart eigenvalue of C. Furthermore, since C has rark 1 , any column vector C wal be an eigenvector belonging to the dominant eigenvalue. For our example, if folows that the deminant eigenvalue of C is 1=3 and that c3 is an eipenvector belonging io 4. If wo divide c3 by the sum of its entries. we end up with the weigh vecter w=(138,134,131)7. In generat, if the decisiens on the relative importance are made in a consistent manner. then there is only one way to choose the weights and both the least scuares mothod and the eigenvector mothod will produce the same weight vector. Suppose now that the decisions are not made in a cansistent mannect This is not uncommon when decisions are made based on human judgments. For the least squares method, the linear system in the variables w1w2+,we1 will not be consistent. but we can always find a least squares solution. Computation of Weights (14) If the eigenvector methed is used. the comparison matrix C1 will not be consistent. By Perron's theorem C1 will have a positive dominant eigervalue 4 and a positive eigenvector X1. The eigenvedor can be scaled to form a vector w1 whose entries add to 1 . The scalsd vector w1 is used to assign weights to the criteria. If the decisions on the relative importance have not been made in a wildly inconeistent mannec, but in a way that is in some sense close to being consistent. then the eigenvector w, is a reasonable cheice tor a weight vector. Computation of Weights (15) In this case. the matrix C1 should in some sense be close to a consistent reciprocal oonparison matrix and 4 and w1 should be close to the dominam oigenvalue and eigenvecter of a consistent matric. Suppose, for example, fhat the search comminee at the college had depided as beloce that teaching is fwice as important as research and 8 times as important as prolessional activites; however, suppose this time they decided that research should ony be 3 times as important as prolessional activities. In this case, the comparison matrix is C7=121812131831 The masrix C1 is not consistent so its domhart eigenvalue 1=3.0092 is not equal to 3 : however, it is close to 3. The eigenvector belonging to 1 ( normalized) is w1=(0.6282.0.2854,0.0864)?. Table 1 summarizos the results for both the problem with the consiatent comparison matrix and fer the inconsistent versien of the probiem. For each cemparison matrix the table includes the dominant eigervalio and the computed weights. All computed values ave rounded to 4 decimal places