Answered step by step
Verified Expert Solution
Question
1 Approved Answer
So, I'm working with taking n = 1 from the following pdf: f ( y ) = 1 e y , y > 0 for
So, I'm working with taking n = 1 from the following pdf:
f(y)=1ey,y>0
for testing
H0:=1
H1:>1
The null hypothesis will be rejected if y3.20. I'm trying to calculat e the Type I error.
Based on the examples in the book that I have, I don't know how to approach this problem as the examples all had a pdf where y was between two values, inclusive. I'm including a problem that I felt was similar, but I can't quite make the jump to solve the problem above.
Help!!
Below is the similar example (I think):
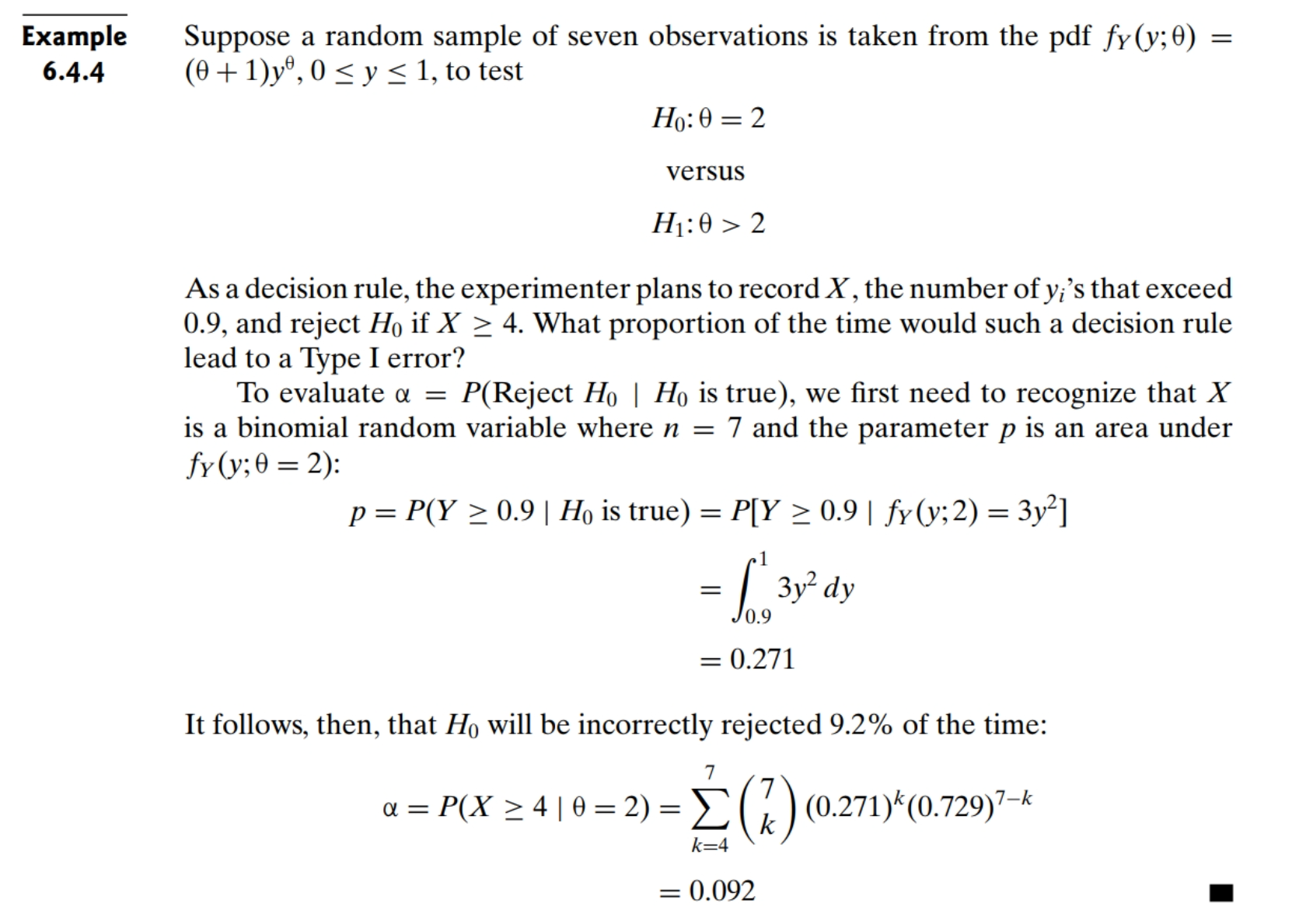
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


