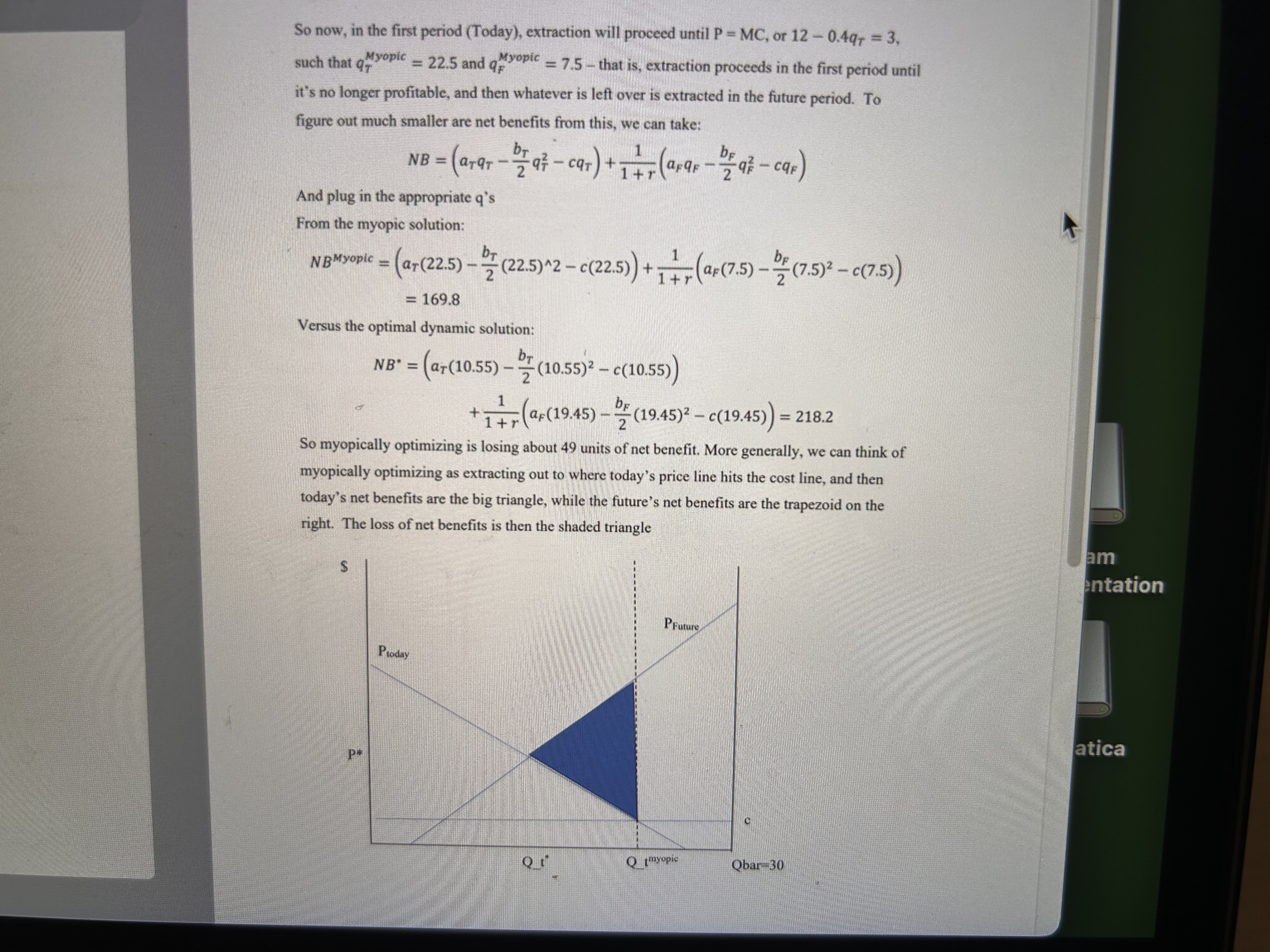
So now, in the first period (Today), extraction will proceed until P = MC, or 12 -0.4qr = 3, opic such that q Myopic = 22.5 and qopic = 7.5- that is, extraction proceeds in the first period until it's no longer profitable, and then whatever is left over is extracted in the future period. To figure out much smaller are net benefits from this, we can take: br = (arar - 17 a - car) + 11+ (arar-a-car) NB= And plug in the appropriate q's From the myopic solution: bf NBMyopic = (ar(22.5)-7 (22.5)^2-c(22.5)) + (ap(7.5) - (7.5)-c(7.5)) = 169.8 Versus the optimal dynamic solution: br 1+r NB = (ar(10.55) - (10.55) - c(10.55)) + bF 11+ (ar (19.45) - DF (19.45) - c(19.45)) = 218.2 2 So myopically optimizing is losing about 49 units of net benefit. More generally, we can think of myopically optimizing as extracting out to where today's price line hits the cost line, and then today's net benefits are the big triangle, while the future's net benefits are the trapezoid on the right. The loss of net benefits is then the shaded triangle S P Ptoday PFuture Q_t* Qtmyopic Qbar-30 am entation atica