Solve all.

1- A paint manufacturer's production process is normally distributed with a mean of 100,000 gallons and a standard deviation of 20,000 gallons. Management wants to incentive bonus for the production crew when the daily production exceeds the 95th percentile of the distribution.

i) At what level of production should management pay the incentive bonus?

ii) What is the probability that the deviation of daily production from mean being greater than 20,000 gallons?

2- A company markets educational software products and is ready to place five new products on the market. Past experience has shown that for this particular software, the chance of "success" is 90%. Assume that the probability of success is independent for each product. (10 points)

i) Find the probability that exactly 2 of the 5 products is successful.

ii) Find the probability that at least 1 product is successful.

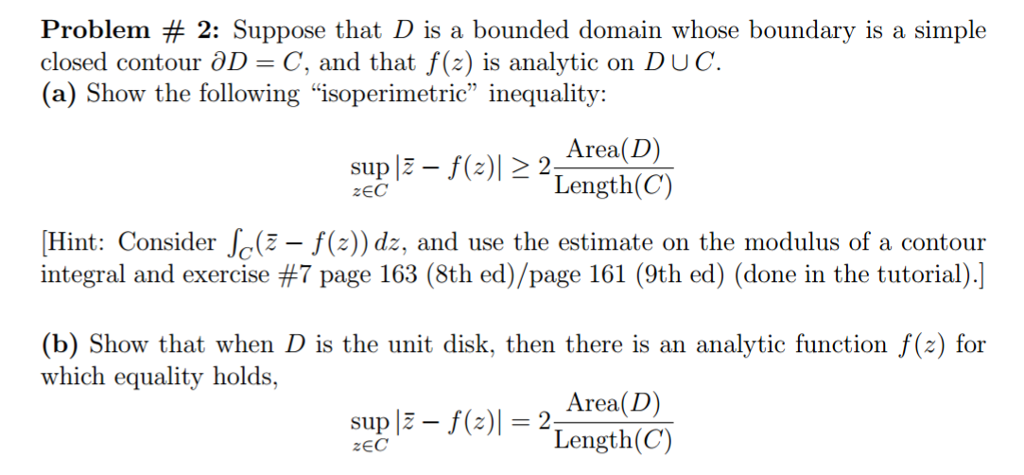
3) Given the following linear programming problem:

Max Z = 5x + 3y

s.t.

3x + y ? 40

2x-y ? 10

What would be the values of x and y that will maximize revenue?

A) x = 13; y = 1 B) x = 13.33; y = 0 C) x = 10; y = 10 D) x = 0; y = 40

6- Sonny Lawler's law office uses EOQ models to manage their office supplies. They've been ordering ink refills for their printers in quantities of 100 units, each $20 (i.e., price of each unit is $20). The firm estimates carrying cost at 25% of its price and that annual demand is about 1000 units per year. The assumptions of the basic EOQ model are thought to apply. For what value of ordering cost would its action be optimal?

7- A bakery uses an average of 50 ounces of organic orange juice daily. Demand is normally distributed with a standard deviation of 12 ounces. The bakery places an order every thirteen days. The lead time for delivery of the juice is three days.

i) Compute the safety stock required to achieve a 90% service level.

ii) If the bakery has 160 ounces at the time an order is placed, how much should be ordered?

s) For the isoperimetric problem done in class, where a string of length L connects the points (0,0) and (1,0) in the xy plane, we found that the equation for a circle (x - 1/2 )2 + (y- b)= = 12 (where ) was the Lagrange multiplier) maximizes the enclosed area. What can you say about the value of b relative a given length L > 1. of lengthProblem # 2: Suppose that D is a bounded domain whose boundary is a simple closed contour 8D = C, and that f (z) is analytic on D U C. (a) Show the following \"isoperimetric\" inequality: _ _ Area(D) :33 '3 \"z\" 2 2Length(0) [Hint Consider fc(2 f(z)) dz, and use the estimate on the modulus of a contour integral and exercise #7 page 163 (8th ed) /page 161 (9th ed) (done in the tutorial).] (b) Show that when D is the unit disk, then there is an analytic function f(z) for which equality holds, _ _ Area(D) 2'23 '3 ' \"z\" ' 2Lengthw) An Isoperimetric Problem The perimeter of a triangle with one unit side is P=atb+1 The area of a triangle is (Heron's Formula) A = TV(a+ b+ 1)(-a+b+1)(a-b+1)(a+b-1) A =IV(-a+20262+ 202-6*+26"-1) We will find the triangle with one unit side with the maximum area for a given perimeter. This is called an "isoperimetric" problem, from the Greek words for "same perimeter". 1. Solve for 4 a. Hint 1: for any differentiable function f(x) 20, 4(f(x))= x) 27(x) b. Hint 2: Don't forget the chain rule! 2. Find for which a does 3. Is this an equilateral triangle (i.e. is a = b = 1)?1.18 (a) The simplest way to derive the Schwarz inequality goes as follows. First, ob- serve (lal + A'(BD -(Ja) + A|8)) 20 for any complex number A; then choose A in such a way that the preceding inequality reduces to the Schwarz inequality. [b) Show that the equality sign in the generalized uncertainty relation holds if the state in question satisfies AA|a) = AAB|a) with A purely imaginary (c) Explicit calculations using the usual rules of wave mechanics show that the wave function for a Gaussian wave packet given by (x'la) = (2xd )- 1/4 exp R(pix' (' -0))] h 412 satisfies the minimum uncertainty relation V((AT)) ((AP))= Prove that the requirement (x'|Axle) = (imaginary number)(x'[Aplx) is indeed satisfied for such a Gaussian wave packet, in agreement with (b)