Solve below maths functions questions. Thanks!

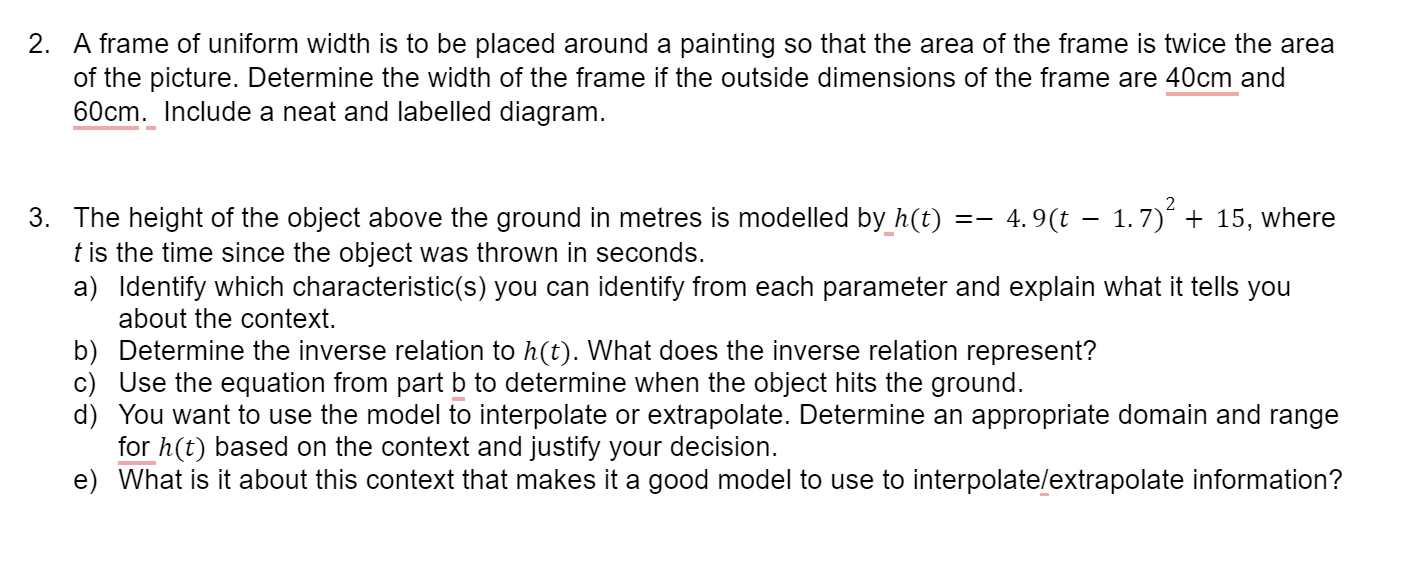
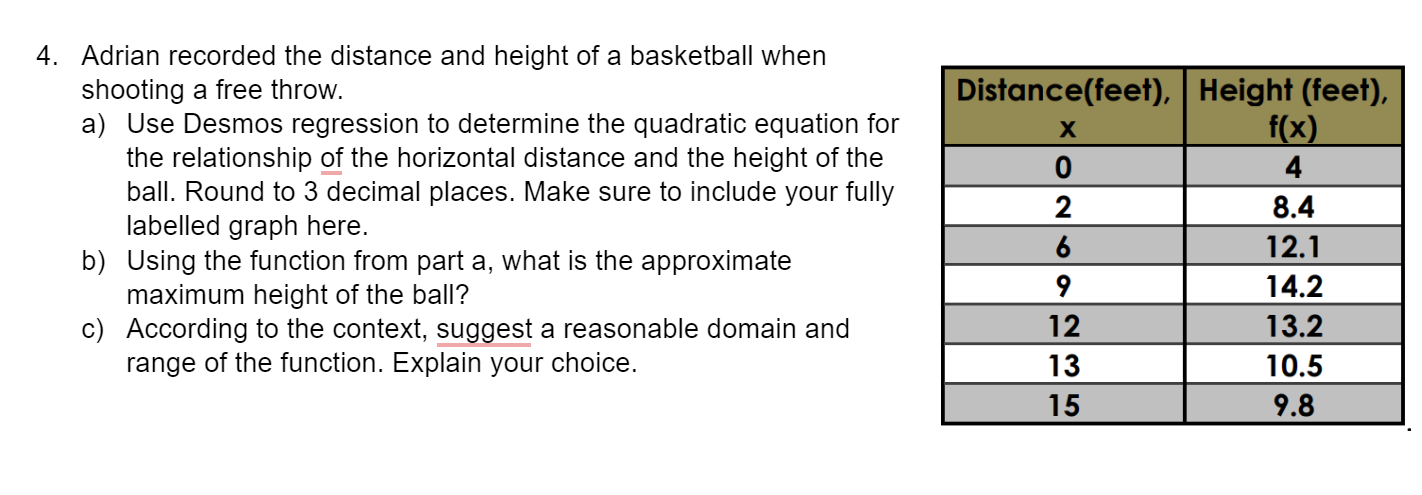
1. You are the manager of a fundraising event. You sell the event tickets at $ ticket and expect [pick a value between 10 and 15] per [pick a value between 500 and 1500 people to attend your event. _ A survey indicates that for every $ [pick a value between 1 and 3, can be a decimal value] increase in price, there will be [pick a value between 40 and 60] less people who will attend the event. a) Determine a function F that models the funds collected as a function of # of price increases. Recall: Revenue = (# of items) * (price per item) b) Determine the maximum funds that the event can raise. What should be the ticket price? c) If their goal is to raise $5000, will they be able to reach their goal?_ If yes, at what ticket price would it be possible? d) The amount per person it costs you to organize this event is $5. Use your expression for the number of people from part (a) to write the cost, C, as a function of # of price increases. Recall: Cost = (# of items) * (cost per item) e) Write a function, P, to determine the profit for this event. Recall: Profit = Revenue - Cost f) Show algebraically that you will break-even at a ticket price of $5. Verify your answer using Desmos. Recall: break-even profit = 0 2. A frame of uniform width is to be placed around a painting so that the area of the frame is twice the area of the picture. Determine the width of the frame if the outside dimensions of the frame are 40cm and 60cm. Include a neat and labelled diagram. - 3. The height of the object above the ground in metres is modelled by_h(t) == 4.9(t 1.7) + 15, where t is the time since the object was thrown in seconds. a) Identify which characteristic(s) you can identify from each parameter and explain what it tells you about the context. b) Determine the inverse relation to h(t). What does the inverse relation represent? c) Use the equation from part b to determine when the object hits the ground. d) You want to use the model to interpolate or extrapolate. Determine an appropriate domain and range for h(t) based on the context and justify your decision. e) What is it about this context that makes it a good model to use to interpolate/extrapolate information? 4. Adrian recorded the distance and height of a basketball when shooting a free throw. a) Use Desmos regression to determine the quadratic equation for the relationship of the horizontal distance and the height of the ball. Round to 3 decimal places. Make sure to include your fully labelled graph here. b) Using the function from part a, what is the approximate maximum height of the ball? c) According to the context, suggest a reasonable domain and range of the function. Explain your choice. Distance(feet), Height (feet), X f(x) 4 8.4 12.1 14.2 13.2 10.5 9.8 0 2 6 9 12 13 15 1. You are the manager of a fundraising event. You sell the event tickets at $ ticket and expect [pick a value between 10 and 15] per [pick a value between 500 and 1500 people to attend your event. _ A survey indicates that for every $ [pick a value between 1 and 3, can be a decimal value] increase in price, there will be [pick a value between 40 and 60] less people who will attend the event. a) Determine a function F that models the funds collected as a function of # of price increases. Recall: Revenue = (# of items) * (price per item) b) Determine the maximum funds that the event can raise. What should be the ticket price? c) If their goal is to raise $5000, will they be able to reach their goal?_ If yes, at what ticket price would it be possible? d) The amount per person it costs you to organize this event is $5. Use your expression for the number of people from part (a) to write the cost, C, as a function of # of price increases. Recall: Cost = (# of items) * (cost per item) e) Write a function, P, to determine the profit for this event. Recall: Profit = Revenue - Cost f) Show algebraically that you will break-even at a ticket price of $5. Verify your answer using Desmos. Recall: break-even profit = 0 2. A frame of uniform width is to be placed around a painting so that the area of the frame is twice the area of the picture. Determine the width of the frame if the outside dimensions of the frame are 40cm and 60cm. Include a neat and labelled diagram. - 3. The height of the object above the ground in metres is modelled by_h(t) == 4.9(t 1.7) + 15, where t is the time since the object was thrown in seconds. a) Identify which characteristic(s) you can identify from each parameter and explain what it tells you about the context. b) Determine the inverse relation to h(t). What does the inverse relation represent? c) Use the equation from part b to determine when the object hits the ground. d) You want to use the model to interpolate or extrapolate. Determine an appropriate domain and range for h(t) based on the context and justify your decision. e) What is it about this context that makes it a good model to use to interpolate/extrapolate information? 4. Adrian recorded the distance and height of a basketball when shooting a free throw. a) Use Desmos regression to determine the quadratic equation for the relationship of the horizontal distance and the height of the ball. Round to 3 decimal places. Make sure to include your fully labelled graph here. b) Using the function from part a, what is the approximate maximum height of the ball? c) According to the context, suggest a reasonable domain and range of the function. Explain your choice. Distance(feet), Height (feet), X f(x) 4 8.4 12.1 14.2 13.2 10.5 9.8 0 2 6 9 12 13 15









