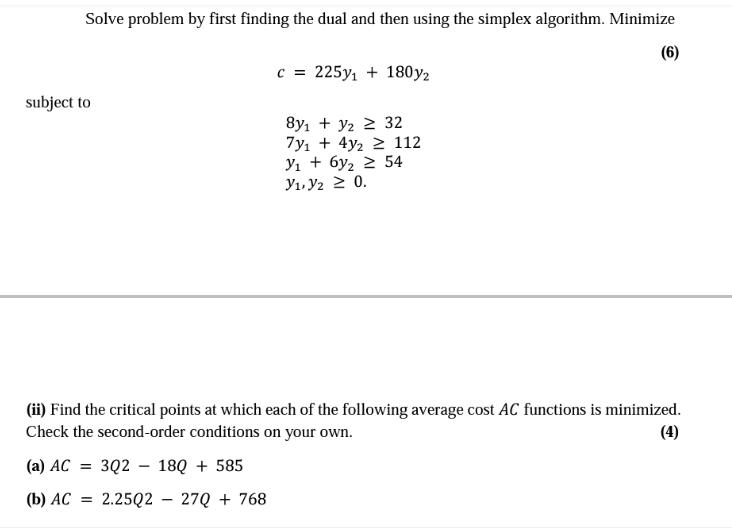
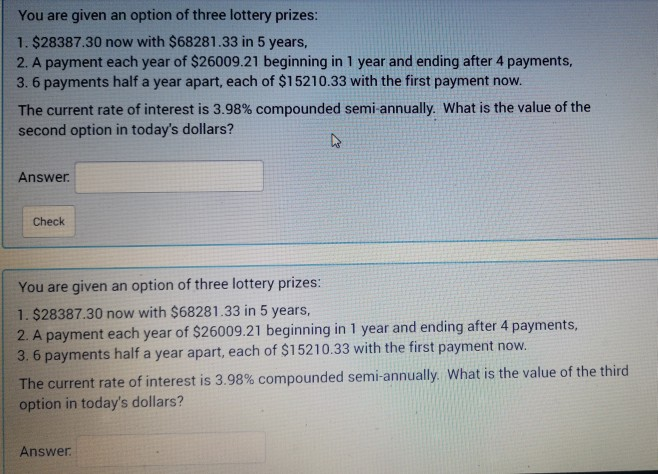
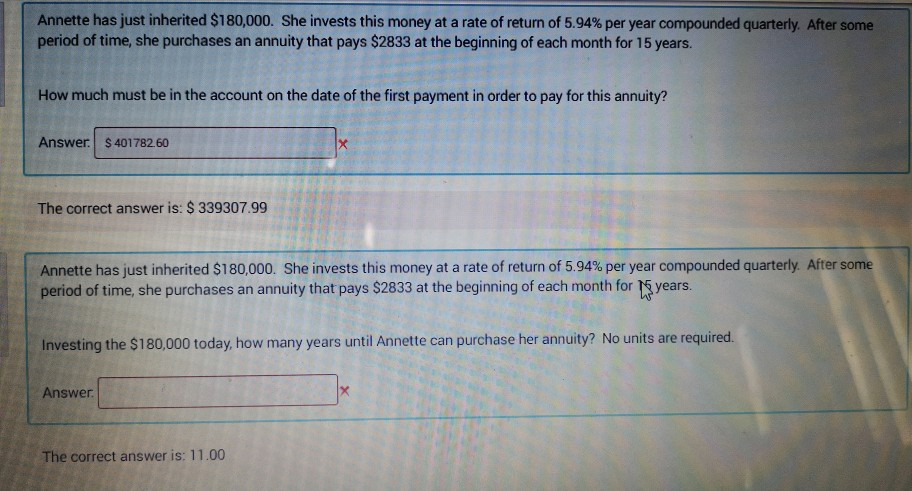
Solve problem by first finding the dual and then using the simplex algorithm. Minimize (6) subject to c = 225y + 180y2 - 8y + y2 32 7y1+ 4y22 112 1 + 54 y, y 0. (ii) Find the critical points at which each of the following average cost AC functions is minimized. Check the second-order conditions on your own. (4) (a) AC = 302 180 + 585 (b) AC = 2.2502 27Q + 768 You are given an option of three lottery prizes: 1. $28387.30 now with $68281.33 in 5 years, 2. A payment each year of $26009.21 beginning in 1 year and ending after 4 payments, 3.6 payments half a year apart, each of $15210.33 with the first payment now. The current rate of interest is 3.98% compounded semi-annually. What is the value of the second option in today's dollars? Answer. Check 4 You are given an option of three lottery prizes: 1. $28387.30 now with $68281.33 in 5 years, 2. A payment each year of $26009.21 beginning in 1 year and ending after 4 payments, 3.6 payments half a year apart, each of $15210.33 with the first payment now. The current rate of interest is 3.98% compounded semi-annually. What is the value of the third option in today's dollars? Answer. A firm is developing a TV advertising campaign. Development costs (fixed costs) are $ 150,000 and the firm must pay $ 15,000 per minute for TV spots. The firm estimates that for each minute of advertising additional sales of $ 70,000 results. Out of this $ 70,000, $ 47,500 is absorbed to cover the variable cost of producing the items and $ 15,000 must be used to pay for the minutes of advertising. Any remainder is the contribution to fixed cost & profit. a) How many minutes of advertising are necessary to recover the development costs of the advertising campaign? b) If the firm uses 15-one minutes spots of advertising on the TV, determine the total revenue, total costs (production & advertising), and total profit (or loss) resulting from this campaign. Annette has just inherited $180,000. She invests this money at a rate of return of 5.94% per year compounded quarterly. After some period of time, she purchases an annuity that pays $2833 at the beginning of each month for 15 years. How much must be in the account on the date of the first payment in order to pay for this annuity? Answer $401782.60 The correct answer is: $ 339307.99 Annette has just inherited $180,000. She invests this money at a rate of return of 5.94% per year compounded quarterly. After some period of time, she purchases an annuity that pays $2833 at the beginning of each month for years. W Investing the $180,000 today, how many years until Annette can purchase her annuity? No units are required. Answer. The correct answer is: 11.00