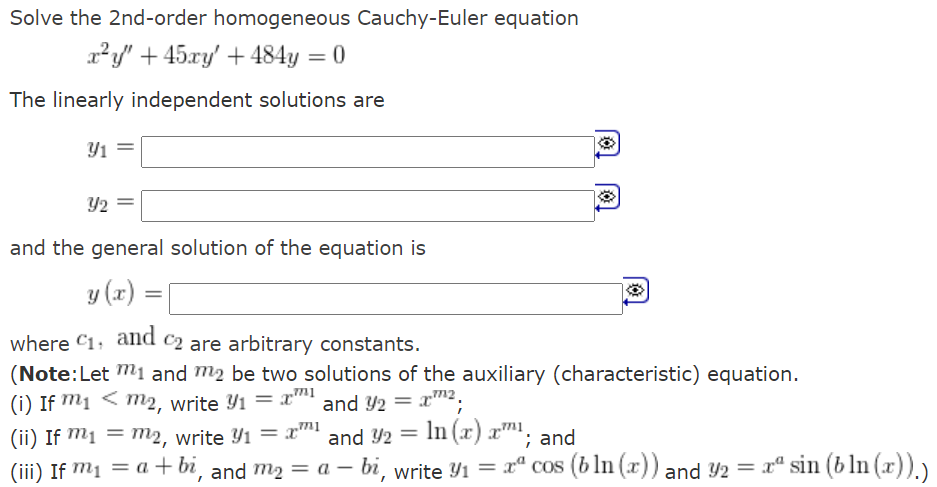
Solve the 2nd-order homogeneous Cauchy-Euler equation xy" +45xy' +484y = 0 The linearly independent solutions are Y1 Y2 and the general solution of the equation is y (r) = where C1, and c2 are arbitrary constants. 0 (Note: Let mi and m be two solutions of the auxiliary (characteristic) equation. (i) If m1 m2, write 31 = 1 and 2 = 22. x y2 x (ii) If m = m2, write 31 = 1 and 2 = ln(x) x; ; and (iii) If m = a + bi, and m = a - bi, write 31 = x cos (bln (x)) and 32 = x sin (bln (x)).)