Solve. the following attachments.

Joyce's utility function is as follows:

U= 10XY2

Where, X, is the quantity of good X consumed, Y, is the quantity of good Y consumed and, U, is Joyce's utility function.

If Joyce spends all of his income, B, on goods X and Y what is the general form of his budget constraint? (Hint: the budget constraint should be specified such that budget is the sum of the expenditures on good X and Y.) Also note that you should use notation such that, PX, is the unit price of good X, and PY, is the unit price of good Y. Please show all of your work to receive full credit (5 points)

What are Joyce's marginal utilities given the information on his total utility curve presented above. That is, derive MUX and MUY Please show all of your work to receive full credit.(10 points)

What is Joyce's marginal rate of substitution? Please show all of your work to receive full credit.(5 points)

Now suppose that Joyce's income is $500, and that the price of good X is 1 and the price of good Y is 2 what is the optimal amount of good X and good Y that he should purchase? Use the information from above to help answer this question.Please show all of your work to receive full credit. ( 10 points)

Suppose the price of good X has increased to 2 and Joyce's income and the price of good Y has remained the same. Now, what is the optimal amount of good X and good Y that he should purchase? Please show all of your work to receive full credit. (10 points)

Plot Joyce's demand curve for good X using your answers from questions 1D and 1E. (5 points).

Given the results you found for questions 1D and 1E, are goods Y and X complements, substitutes or neither? Please explain (5 points).

Section II: Another application of consumer utility - (25 points)

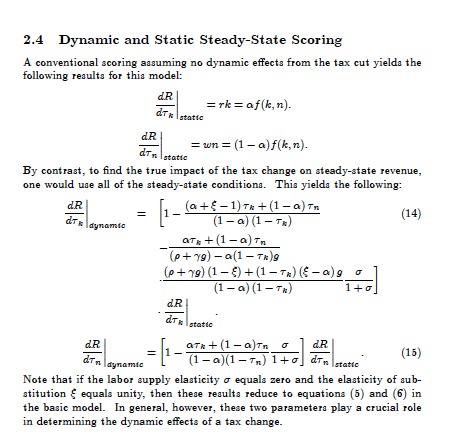
Suppose that John Gray spends his entire income on food and clothing. The total utility that he derives from each good (shown below) is independent of the amount consumed of the other good. The price of food is $100 and the price of clothing is $500.

Table 2.2

John Gray's

Number of unitstotal utility

of goods consumedFood (lbs)Clothing (yds)

000

12050

23895

354135

467170

577200

683225

787245

889260

990270

1090275

a.) If John Gray has an income of $1,000 per month, how many units of each should he purchase to maximize his total utility? Please show all of your work to receive full credit.(5 points)

b.) What happens to his total utility if he substitutes a unit of clothing for an additional five units of food? Please show all of your work to receive full credit.(5 points)

c.) What is the new total utility maximizing market basket for John's new utility maximizing market basket, given his new income level of $2,000. Please show all of your work to receive full credit.(5 points)

d.) If the price of food rises to $250 per unit, what is John's new utility maximizing market basket, given his new income level of $2,000. Please show all of your work to receive full credit.(5 points)

e.) Using the information provided from this exercise determine whether food and clothing are complementary goods, substitute goods or neither for John. Please show all of your work to receive full credit.(5 points)

2.4 Dynamic and Static Steady-State Scoring A conventional scoring assuming no dynamic effects from the tax cut yields the following results for this model: d.R =rk =of (k, n). dix static d.R =wn = (1 - a)f(k,n). an static By contrast, to find the true impact of the tax change on steady-state revenue, one would use all of the steady-atate conditions. This yields the following: = 1_ (a+(-1) Tat (1 -Q) Tn (14) AT & dynamic (1 -a) (1-72) are+(1-a)in (p+ 79) - a(1 - TA)9 (p+ 79) (1 - 5) + (1 - JA) (6 -0)9 0 (1 -a) (1-7*) 1to d.R Ta static AR at+ (1 - a)in d.R. (15) din dynamic (1-a)(1- 7) 1+] din static Note that if the labor supply elasticity o equals zero and the elasticity of sub- stitution { equals unity, then these results reduce to equations (5) and (6) in the basic model. In general, however, these two parameters play a crucial role in determining the dynamic effects of a tax change.6. Consider an investor who, on January 1, 2016, purchases a TIPS bond with an original principal of $100,000, an 8 percent annual (or 4 percent semiannual) coupon rate, and 10 years to maturity. (LG 6-2) a. If the semiannual inflation rate during the first six months is 0.3 percent, calculate the principal amount used to determine the first coupon payment and the first coupon payment (paid on June 30, 2016). b. From your answer to part a, calculate the inflation-adjusted principal at the beginning of the second six months. c. Suppose that the semiannual inflation rate for the second six-month period is 1 percent. Calculate the inflation-adjusted principal at the end of the second six months (on December 31, 2016) and the coupon pay- ment to the investor for the second six-month period. What is the inflation-adjusted principal on this coupon payment date