Question: Solve the initial value problem dx dt with x(0) = -2. x(t): = - 4x = cos(2t) (1/10)(sin2t-2cos2t)-(9/5)(e^(4t)) (1 point) Find the general solution,

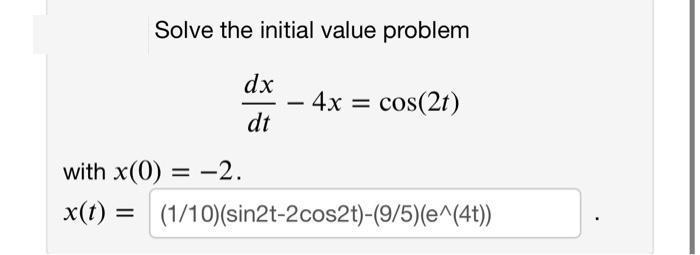
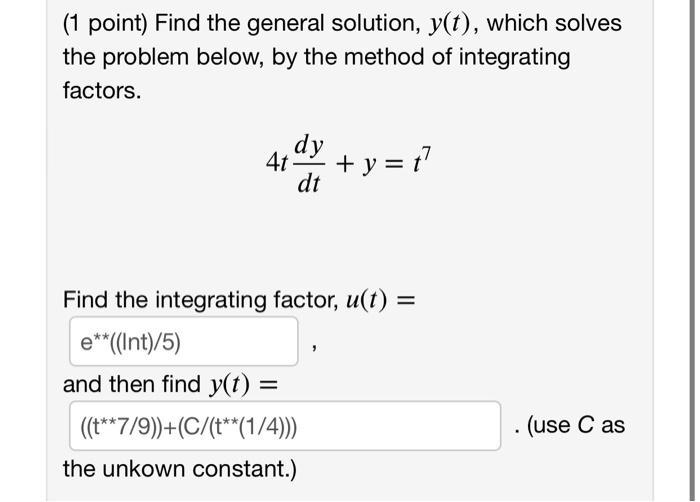
$( 1 point) Find the general solution, $ y(t) $, which solves the problem below, by the method of integrating factors.\[4$

Solve the initial value problem dx dt with x(0) = -2. x(t): = - 4x = cos(2t) (1/10)(sin2t-2cos2t)-(9/5)(e^(4t)) (1 point) Find the general solution, y(t), which solves the problem below, by the method of integrating factors. dy 4t +y=t dt Find the integrating factor, u(t): = e**((Int)/5) and then find y(t): ((t**7/9))+(C/(t**(1/4))) the unkown constant.) . (use C as

Step by Step Solution

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Accounting Questions!

Solve the initial value problem Explain how orthogonality can help. LI 1-6)u, u(0) =(1

Initial Values Specified for Problems 1-3 solve the initial value problems? 1. y" - 25y = 0, y(0) = 1, y' (0) = 0 2. y" - y'- 2y = 0, y(0) = 1, y' (0) = 0 3. y" + 2y' + y, y(0) = 0, y' (0) = 0

Solve the initial value problem by a power series. Graph the partial sums of the powers up to and including x 5 . Find the value of the sum s (5 digits) at x 1 . (1 - x 2 )y" - 2xy' + 30y = 0, y(0) =...

Solve the initial value problem dx dt Ax with x 0 xo 4 2 0 A 3 i 1 x t

Solve the Initial Value Problem. dy dt te", y(1) = 0 = = y

Proposition 3 Let x J y J be two solutions of the initial value problem dx f t x x t x dt then x t y t for all 1 JOJ Proof Recall that both x and y being solutions of the initial value problem...

April 26, 2017 CODE 111 Page 9 B5. Solve the initial value problem: dy + = 2e(x2) with y(1) = 2e. marks dx

In Exercises 3-6, solve the initial value problem x'(t) = Ax(t) for t > 0, with x(0) = (3, 2). Classify the nature of the origin as an attractor, repeller, or saddle point of the dynamical system...

The reaction of chemical compounds can often be modeled by differential equations. Let y(t) be the concentration of a substance in reaction for t 0 (typical units of y are moles/L). The change in...

Use Eulers method to solve the initial value problem graphically, starting at x 0 = 0 with a. dx = 0.1. b. dx = -0.1. dy dx x + y ey + xy(0) = 0

Neci and Olwen want to form and do business as a corporationPastries & Pies Inc. a corporation is a legal entity created and recognized by A.A central federal administrative agency. B.A city or...

Mortgage Payoff: You take out a 30-year fixed rate mortgage for a house. The amount borrowed is $300,000. The interest rate is 4% per year compounded monthly. You decide to sell the house immediately...

A community hospital system realized that approximately 2,000 patients were leaving its emergency department (ED) each year without receiving medical attention. This hospital system realized that...

Describe what a quality control activity might look like at a t-shirt manufacturing factory. (LO3.3)

Save & Exit Brandon, an individual, began business four years ago and has sold 1231 assets with $5,900 of losses within the last five years. Brandon owned each of the assets for several years. In the...

The Lim Company has a plant that manufacturers transistor radios. The production time is only a few minutes per unit. The company uses a just-in- time production system and a backflush costing system...

1. A company uses options to hedge its merchandise inventory. If the options qualify as a fair value hedge of the inventory, and analysis of hedge effectiveness excludes option time value, which...

Baxter, Inc., owns 90 percent of Wisconsin, Inc., and 20 percent of Cleveland Company. Wisconsin, in turn, holds 60 percent of Clevelands outstanding stock. No excess amortization resulted from these...

State the divergence theorem from memory. Give applications.

Showing the details, develop 63x 5 - 90x 3 + 35x

Find the center and the radius of convergence.

2. What kinds of things or events in your life do you remember? How do those memories differ from those around you? Of people from different cultures? How do you think those differences in memories...

2. Describe examples of how cross-cultural research findings in attention, categorization, attributions, reasoning, or memory support the framework.

3. Ever notice how people see the world differently? How do people come to have their views of the world? Their perceptions? Take any of the figures in the section on optical illusions and conduct...