solve the question

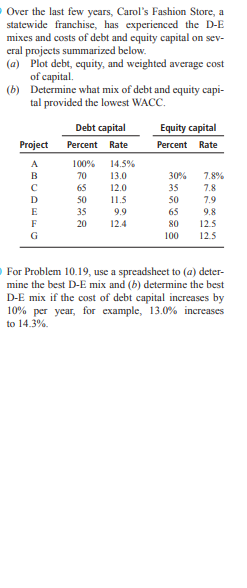
If an alternative has a salvage value, how is it han- dled in the calculation of a B/C ratio relative to benefits, disbenefits, costs, or savings? The cost of grading and spreading gravel on a short rural road is expected to be $300,000. The road will have to be maintained at a cost of $25,000 per year. Even though the new road is not very smooth, it allows access to an area that previously could only be reached with off-road vehicles. The improved accessibility has led to a 150% increase in the property values along the road. If the previ- ous market value of a property was $900,000, calculate the B/C ratio using an interest rate of 6% per year and a 20-year study period. Arsenic enters drinking water supplies from natu- ral deposits in the earth or from agricultural and industrial practices. Since it has been linked to cancer of the bladder, kidney, and other internal organs, the EPA has lowered the arsenic standard for drinking water from 0.050 parts per million to 0.010 parts per million (10 parts per billion). The annual cost to public water utilities to meet the new standard is estimated to be $200 per house- hold. If it is estimated that there are 90 million households in the United States and that the lower standard can save 50 lives per year valued at $4,000,000 per life, what is the benefit/cost ratio of the regulation?Over the last few years, Carol's Fashion Store, a statewide franchise, has experienced the D-E mixes and costs of debt and equity capital on sev- eral projects summarized below. (a) Plot debt, equity, and weighted average cost of capital. (b) Determine what mix of debt and equity capi- tal provided the lowest WACC. Debt capital Equity capital Project Percent Rate Percent Rate A 100% 14.5% B 70 13.0 30% 7.8% C 65 12.0 35 7.8 50 11.5 7.9 35 9.9 65 9.8 20 12.4 20 125 100 12 5 For Problem 10.19, use a spreadsheet to (a) deter- mine the best D-E mix and (b) determine the best D-E mix if the cost of debt capital increases by 10% per year, for example, 13.0% increases to 14.3%Fairmont Industries primarily relies on 100% eq- uity financing to fund projects. A good opportunity is available that will require $250,000 in capital. The Fairmont owner can supply the money from personal investments that currently earn an aver- age of 7.5% per year. The annual net cash flow from the project is estimated at $30,000 for the next 15 years. Alternatively, 60% of the required amount can be borrowed for 15 years at 7% per year. If the MARR is the WACC, determine which plan, if either, should be undertaken. This is a before-tax analysis. Omega Engineering Inc. has an opportunity to in- vest $10,000,000 in a new engineering remote control system for offshore drilling platforms. Fi- nancing will be split between common stock sales ($5,000,000) and a loan with an 8% per year inter- est rate. Omega's share of the annual net cash flow is estimated to be $1.35 million for each of the next 5 years. Omega is about to initiate CAPM as its common stock evaluation model. Recent analy- sis shows that it has a volatility rating of 1.22 and is paying a premium of 5% on its common stock dividend. The U.S. Treasury bills are currently paying 4% per year. Is the venture financially at- tractive if the MARR equals (a) the cost of equity capital and (b) the WACC?Chebyshev's inequality, (see Exercise 44, Chapter 3), is valid for continuous as well as discrete distributions. It states that for any number k satisfying k 2 1, P(IX - p 2ko) =1/k (see Exercise 44 in Chapter 3 for an interpretation). Obtain this probability in the case of a normal distribution for , 2, and 3, and compare to the upper bound. Reference exercise 44 A result called Chebyshev's inequality states that for any probability distribution of an rv X and any number k that is at least 1, P( | X - p | k o) $ 1/k2 . In words, the probability that the value of X lies at least k standard deviations from its mean is at most 1/12. a. What is the value of the upper bound for k = 2? K + 3? K = 4? K= 5? K = 10? b. Compute p and o for the distribution of Exercise 13. Then evaluate P()X - p| 2 ko) for the values of k given in part (a). What does this suggest about the upper bound relative to the corresponding probability? c. Let X have possible values -1, 0, and 1, with probabilities 1/18, 8/9 and 1/8 , respectively. What is P(1X - p/2 30), and how does it compare to the corresponding bound? d. Give a distribution for which P(IX - p|2 50) = .04.For the data shown, calculate the conventional B/C ratio at i = 6% per year. Benefits: $20,000 in year () and $30,000 in year 5 Disbenefits: $7000 in year 3 Savings (to government): $25,000 in years 1-4 Cost: $100,000 in year 0 Project life: 5 years Explain a fundamental difference between the modified B/C ratio and the profitability index. Gerald Corporation entered a public-private part- nership using a DBOM contract with the state of Massachusetts 10 years ago for railroad system upgrades. Determine the profitability index for the financial results listed below using a MARK of 8% per year. Investments: Year 0 $-4.2 million Year 5 $-3.5 million Net savings: Years 1-5 $1.2 million per year Years 6-10 $2.5 million per year A project had a staged investment distributed over the 6-year contract period. For the cash flows shown (next page) and an interest rate of 10% per year, determine the profitability index and deter- mine if the project was economically justified.The two-parameter gamma distribution can be general- ized by introducing a third parameter y, called a thresh- old or location parameter: replace x in (4.8) by x - y and x 2 0 by x = y. This amounts to shifting the density curves in Figure 4.27 so that they begin their ascent or descent at y rather than 0. The article "Bivariate Flood Frequency Analysis with Historical Information Based on Copulas" (J. of Hydrologic Engr., 2013: 1018-1030) employs this distribution to model X = 3-day flood volume (10 m'). Suppose that values of the parameters are a = 12, 8 = 7, y = 40 (very close to estimates in the cited article based on past data). a. What are the mean value and standard deviation of X? b. What is the probability that flood volume is between 100 and 150? c. What is the probability that flood volume exceeds its mean value by more than one standard deviation? d. What is the 95th percentile of the flood volume distribution?An ecologist wishes to select a point inside a circular sampling region according to a uniform distribution (in practice this could be done by first selecting a direction and then a distance from the center in that direction). Let X = the x coordinate of the point selected and Y = the y coordinate of the point selected. If the circle is centered at (0, 0) and has radius R, then the joint pdf of X and Yis TT R2 x + y's R f(x, y) = 0 otherwise a. What is the probability that the selected point is within R/2 of the center of the circular region? [Hint: Draw a picture of the region of positive density D. Because f(x, y) is constant on D, computing a probability reduces to computing an area.] b. What is the probability that both X and Y differ from 0 by at most R/2? C. Answer part (b) for replacing RIV2. d. What is the marginal pdf of X? Of Y? Are X and Y independent