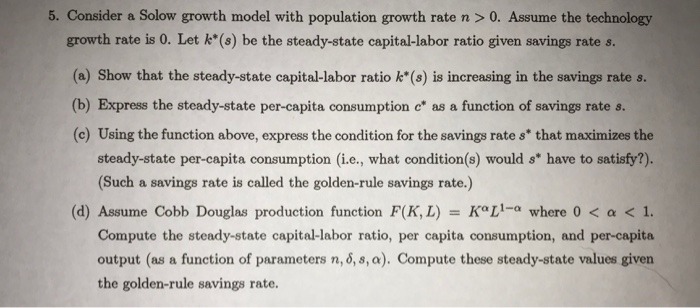
.Solve these questions correctly.

Be sure that your responses model clear economic reasoning and addresses each of the following:

Question

Part 1.) Consider the discrete Bertrand game described in the Oligopoly lecture notes/video. According to the rules of this game each student selects a number from the set {0,1,2, 3, 4, 5, 6, 7, 8, 9, 10} and is randomly matched with another student. Whoever has the lowest number wins that amount in dollars and whoever has the high number wins zero. In the event of ties, each student receives half their number in dollars. What number would you select if you played this game in our online class? Explain your reasoning.

Part 2.) Continue to consider this discrete Bertrand model, but now assume that each student has a constant cost of 5 that is deducted from all payoffs. So whoever has the low number wins their number, minus 5. Whoever has the high number loses 5 total. In the event of a tie, each student wins an amount equal to their number divided by two, then minus five. Find any Nash equilibria in this game. Explain your reasoning.

3: We will think about oligopoly in a few forms: basic cournot model, the discrete

Bertrand model, the undifferentiated Bertrand model, and a comment on the differentiated

Bertrand model.

4: In 1838 Augustin Cournot set the foundation for modern mathematical

microeconomics. He's thinking about a situation where producers choose a quantity level in

order to maximize their profits. This makes sense in the context he was observing which was

producers of mineral water?each seller chose how many jugs to fill knowing the market price

would adjust when they all returned to town to sell.

5: In the Cournot model we say firms' strategies are strategic substitutes meaning the

marginal payoff of a firm's action is decreasing in the action of their rival. It's better for each

individual when the rivals bring a smaller quantity to market. The smaller the quantity, the

higher the market price shared by all. If we assume we have just two firms, we can see this with

a generic linear demand curve, P=a - bQ where Q = q1 + q2, so then P=a - bq1 - bq2. Clearly

the quantity choice of firm two lowers the market price for both and therefore affects the quantity

that firm one optimally chooses.

6: There's a negative externality here?each firm considers only the effect of the lower

market price on its own output, not the effect on the aggregate output. So each firm chooses to

overproduce. Optimally the firms want to jointly supply something along the lines of the

monopoly quantity!

7: Now let's think of a different model, one due to Josef Bertrand who wrote in 1838.

Cournot thought of firms as strategically selecting quantities, Bertrand thinks its more natural to

consider price competition. I'll spend some time on Bertrand's model as a strategic form game.

8: First, here's a picture of the two fellows.

9: So a game is just a mathematical object that involves a set of players a set of strategies

and a set of payoffs.

10: Our players are just the firms, since we're thinking of the Bertrand model, strategy

will just be prices. Payoffs will be the profits. I'll assume a discrete game where firms can

choose as their price an integer from the set 0 to 10. A firm earns 0 if it has the high price, it

earns it's own price, pi, if it has the low price, and it earns half it's price if it splits the market

with its rival.

11: We can think of this as a market with one prospective buyer, there's no costs and one

unit is sold, so profit is just the selling price.

12: What outcome do we expect? Let's apply the Nash equilibrium, which is defined as a

strategy profile wherein no player has a profitable unilateral deviation. NE requires that no

player can improve their payoff by switching to another strategy while the co-player keeps

playing the original strategy. So what are the NE in this game?

13: Let's start at the bottom. How about (0,0) meaning player 1 selects 0 and player 2

selects 0. Here both receive payoffs of zero. And no one can improve by selecting a higher

number. So this is Nash.

14: What about (1,1), meaning player 1 selects 1 and player 2 also sets a price equal to 1.

Then each receives a payoff of . Well, suppose they try something different. Player 1 can

capture the market by undercutting with P=0, but that results in profits of 0. There's no benefit to

increasing price, clearly. So this is Nash also.

15: What about (2,2) meaning player 1 selects 2 and player 2 also sets a price equal to 2.

Here both receive payoffs of 1. Raising the price leads to losing the market and profits of zero.

Lowering the price, for instance to P=1, leads to capturing the market but still making payoff

equal to 1?not a profitable deviation. So this is Nash. There are 3 Nash!

16: Are there more? Well let's try (3,3). Here the payoff is 1.5 to each. But either can

deviate to a price of 2, and capture the market to receive a payoff of 2. So (3,3) is not Nash.

Neither are any other pairs of higher prices. So we found a total of 3 Nash Equlibria!

17: Now let's consider a different game, suppose strategies are drawn from the smaller

set that omits 0 and 1, So players pick integers from 2 to 10. Now find the Nash equlibria!

18: There's just one, it's (2,2). Here the payoffs to each are 1 and there's no way to

improve.

19: Again (3,3) is not a Nash equilibrium in the previous version of the game or the

present one.

20: We can think about the Bertrand game more generally. Here's the formal

representation of the standard Bertrand model with undifferentiated products. Now instead of

selecting integer prices, I'll assume that players can pick prices based on a demand curve Q=api.

So then payoffs are just profits.

21: In this version, the prediction is that players should set price equal to marginal cost.

I've sketched a proof to demonstrate that there's no possible unilateral deviation from the P=MC

Nash equilibrium. Basically what you need to know is that if all firms are currently setting price

equal to marginal cost, no individual can gain by pricing higher or lower.

22: Interestingly, this leads to the implication of the competitive market equilibrium with

only two firms. We might think the two firms would coordinate or collude to keep prices high?

but they don't.

23: If we allow any number of firms, the NE are the same. There's always a NE where at

least two firms play P=MC?in fact there's many, many of these depending on how many other

firms there are and what everyone else does. This seems odd?we typically expect that an

increase in the number of firms will increase competition?but this result tells us that in this

model, two firms are enough, and additional firms don't make the market any more competitive!

24: Note: this is a prediction unique to the undifferentiated Bertrand model. When we

allow product differentiation we get a wildly different prediction. When goods are not perfectly

substitutable, we get a solution where each firm sets a price higher than marginal cost.

25: So in the differentiated Bertrand, we say that firms' strategies are strategic

complements. The marginal payoff of one firm's action is increasing in the action of others.

Firms here like it when their rivals set higher prices, because then they can too. But note,

ordinary Bertrand behaviors can look like collusion to raise prices?but this is not the case

necessarily?firms might be jointly raising prices not because of anything illegal but because of

natural oligopoly dynamics.

5. Consider a Solow growth model with population growth rate n > 0. Assume the technology growth rate is 0. Let k*(s) be the steady-state capital-labor ratio given savings rate s. (a) Show that the steady-state capital-labor ratio k* (s) is increasing in the savings rate s. (b) Express the steady-state per-capita consumption c* as a function of savings rate s. (c) Using the function above, express the condition for the savings rate s* that maximizes the steady-state per-capita consumption (i.e., what condition(s) would s* have to satisfy?). (Such a savings rate is called the golden-rule savings rate.) (d) Assume Cobb Douglas production function F(K, L) = KoLl-a where 0