Some examples of how the multiplication & division tabular approaches should look. Would really appreciate the help with these questions please.

Just learning binary conversion, so some help would be appreciated please.

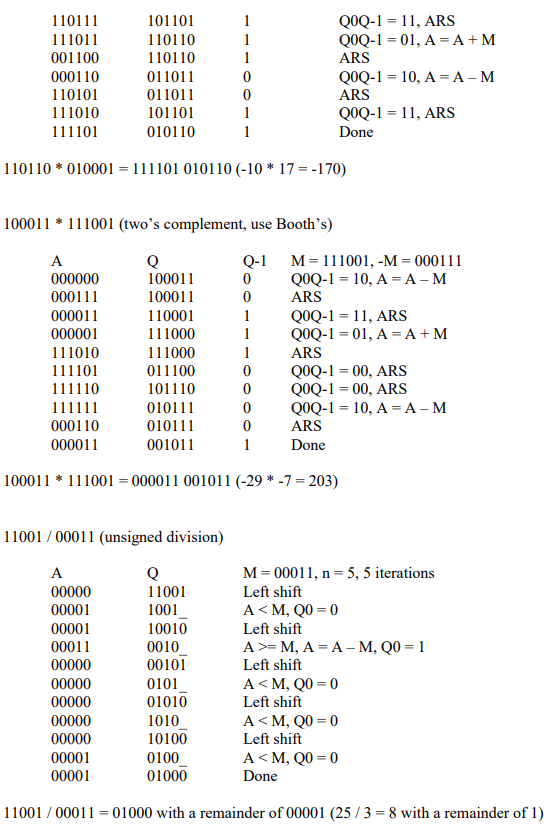
Word Processor all answers (figures or answers that are too awkward to type can be hand-written or hand-drawn). Each problem (e.g,. la, lb, 2a, 3b, etc) is worth 5 points. Show your work for a chance at partial credit - if you don't show your work and you get an answer wrong, you get it all wrong! NOTE: remember to include minus signs for any negative numbers. 1. Convert 11011000 to decimal assuming the number is stored in each of the following representations: a. Unsigned magnitude b. Signed magnitude c. One's complement d. Two's complement 2. Convert 1000111101101000 to decimal assuming that the number is stored in a. Unsigned magnitude b. Signed magnitude c. One's complement d. Two's complement 3. Convert -13033 from decimal to each of the following a. 16-bit signed magnitude b. 16-bit one's complement c. 16-bit two's complement 4. Using the 14-bit floating point representation from chapter 2 (figure 2.2) where exponents are represented using excess-16, convert the following a. b. c. d. 11011110110011 to decimal 19.375 to binary 00111011010000 to decimal -5.6 to binary 5. Do the following binary multiplication and division problems. Use the tabular approach (as covered in class, see the sample problems on the web site and power point notes). If you do not show the work via the tabular approach, you will get no credit. For a, d & e, the numbers are unsigned magnitude. For b & c, the numbers are two's complement. The multiplication problems use 5-bit numbers and the division problems use 6-bit numbers a. b. c. d. e. 11101 * 10010 (use the unsigned multiplication algorithm) 11010 *01011 (use Booth's algorithm) 10010 * 10100 (use Booth's algorithm) 111000/000101 (use the unsigned division algorithm) 100110/000011 (use the unsigned division algorithm) Perform the following multiplication and division problems 101110 * 001100 (unsigned magnitude) M-001100, n-6, iterate 6 times 001100 000110 010010 001001 010101 001010 000101 010001 001000 101110Q0 0, right shift 010111 010111 001011 001011 000101 000101 100010Q0 0, right shift 010001 010001 101000 Q0 1, add M to A Right shift Q0-1, add M to A Right shift Q0, add M to A Right shift Q0 1, add M to A Right shift Done 101110* 001100 001000 101000 (46 * 12-552) 010111 * 111000 (unsigned magnitude) 111000 011100 010100 101010 100010 110001 01 1000 010000 010111 010111 001011 001011 000101 000101 000010 100001 100001 M=111000, n = 6 Q0-1, add M to A Right shift Q0, add M to A Right shift Q0-1, add M to A Right shift Q0 0, right shift Q0, add M to A Right shift 0 101000 010000 Q0-0, right shift 010100 001000 Done 010111 * 111000 010100 001000 (23 56- 1288) 110110 * 010001 (two's complement, use Booth's algorithm) M-010001,-M-101 1 1 1 Q0Q-1 00, arithmetic right shift (ARS) Q0Q-1-10, A-A- M Q-1 110110 011011 011011 101111 110111 111011 001100 000110 110101 111010 111101 101101 110110 110110 011011 011011 101101 010110 Q0Q-1-11, ARS Q0Q-1-10, A-A- M Q0Q-1-11, ARS Done 110110* 010001-111101 010110 (-10 * 17--170) 100011 * 111001 (two's complement, use Booth's) Q- M 111001, -M-000111 0 Q0Q-1-10, A A- M 100011 100011 110001 111000 111000 011100 101110 010111 010111 001011 000111 000011 000001 111010 111101 1 Q0Q-1, ARS 0 Q0Q-1-00, ARS 0 Q0Q-1-00, ARS 0 Q0Q-1-10, A A- M 000110 000011 ARS ne 100011 * 1001-000011 001011 (-29 * -7-203) 11001 /00011 (unsigned division) M-00011, n-5, 5 iterations 11001 1001 10010 0010 00101 0101 01010 1010 10100 0100 01000 Left shift Left shift Left shift Left shift Left shift Done 00001 00001 00011 00001 00001 11001 /00011 01000 with a remainder of 00001 (25 /3-8 with a remainder of 1) 111101 /011001 (unsigned division) M-011001, n-6 (6 iterations) Left shift 000001 000001 000011 000011 000111 000111 001111 001111 011110 000101 001011 001011 111101 11101 111010 11010 110100 10100 101000 01000 010000 10000 100001 00001 000010 Left shift Left shift Left shift A