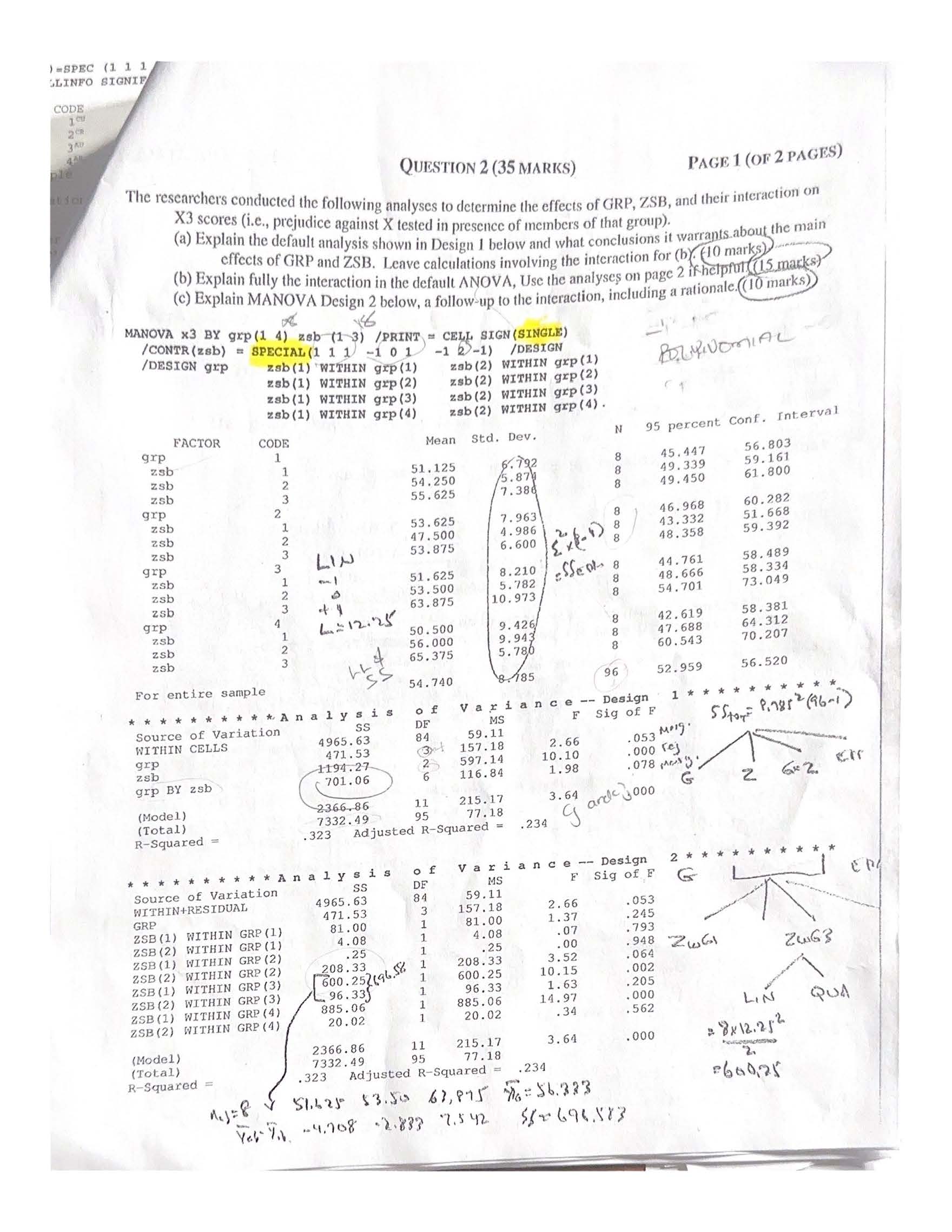
)-SPEC (1 1 1 SLINFO SIGNIF CODE 1 cu 2 CR ple 3AD 4A atdor QUESTION 2 (35 MARKS) PAGE 1 (OF 2 PAGES) The researchers conducted the following analyses to determine the effects of GRP, ZSB, and their interaction on X3 scores (i.e., prejudice against X tested in presence of members of that group). (a) Explain the default analysis shown in Design I below and what conclusions it warrants about the main effects of GRP and ZSB. Leave calculations involving the interaction for (b) (10 marks) (b) Explain fully the interaction in the default ANOVA, Use the analyses on page 2 if helpful ((15 marks) (c) Explain MANOVA Design 2 below, a follow-up to the interaction, including a rationale.((10 marks) /DESIGN grp MANOVA x3 BY grp (14) zeb (13) /PRINT /CONTR (zsb) = SPECIAL (1 1 1 -1 0 1 zsb (1) WITHIN grp (1) zsb (1) WITHIN grp (2) zsb (1) WITHIN grp (3) zsb (1) WITHIN grp (4) = CELL SIGN (SINGLE) -1 2-1) /DESIGN zab (2) WITHIN grp (1) zsb (2) WITHIN grp (2) zsb (2) WITHIN grp (3) zsb (2) WITHIN grp (4). Std. Dev. 6.792 888 BOLYNOMIAL FACTOR CODE grp Mean N 95 percent Conf. Interval 1 zsb 1 zsb 51.125 45.447 56.803 2 54.250 5.879 49.339 59.161 zsb 3 55.625 7.386 49.450 61.800 grp 2 zsb 1 53.625 7.963 zsb 2 47.500 4.986 zsb 3 grp 3 LIN 53.875 6.600 zsb 1 51.625 8.210 zsb Essent 2 9 53.500 5.782 zsb 3 63.875 10.973 888 888 46.968 60.282 43.332 51.668 48.358 59.392 44.761 58.489 48.666 58.334 54.701 73.049 grp 4 zsb 1 b=12.25 50.500 9.426 8 42.619 58.381 zsb 2 56.000 9.943 8 47.688 64.312 zsb 3 65.375 5.780 8 60.543 70.207 For entire sample Analysis 54.740 8.185 96 52.959 56.520 of Variance -- Design Source of Variation WITHIN CELLS grp zsb SS DF MS F Sig of F 4965.63 471.53 84 59.11 1194.27 2 grp BY zsb 701.06 326 3x+ 157.18 2.66 597.14 10.10 116.84 1.98 (Model) (Total) R-Squared 2366.86 11 215.17 7332.49 95 77.18 .323 Adjusted R-Squared = .234 Analysis of Design 3.64 .053 M19. .000 rej .078 and 3000 9 Variance -- G **** 5Stay = 8.7852 (96-1) * N Cir 6x2. 2*** **** Source of Variation WITHIN+RESIDUAL SS DF 4965.63 84 MS 59.11 F Sig of F G GRP ZSB (1) WITHIN GRP (1) ZSB (2) WITHIN GRP (1) ZSB (1) WITHIN GRP (2) 2SB (2) WITHIN GRP (2) ZSB (1) WITHIN GRP (3) ZSB (2) WITHIN GRP (3) ZSB (1) WITHIN GRP (4) ZSB (2) WITHIN GRP (4) (Model) (Total) R-Squared 1+1=8 .25 208.33 600.257196 1 96.33 885.06 Yet-74 -4.708 471.53 3 157.18 2.66 .053 81.00 1 81.00 1.37 .245 4.08 1 4.08 .07 .793 1 .25 .00 .948 26 208.33 3.52 ZWG3 .064 1 600.25 10.15 .002 1 96.33 1.63 .205 1 885.06 14.97 .000 20.02 1 20.02 .34 .562 2366.86 11 7332.49 95 215.17 77.18 3.64 .000 LIN 8x12.252 HUGECS570) .323 Adjusted R-Squared "Z =2 .234 51.425 53.Sis 63,875 76=56.333 860035 +2.833 7.542 552696.583 represents the simple effect of des for abstract words (parameter 3 or 2ND Parameter), and -1 -1 11 represent the PAGE 2 (OF 2 PAGE 501646 " 2 3 RECODE grp (12 = -1) (3 4 RECODE grp (1 = -1) (2 = QUESTION 2 = 1) INTO g1. 1) (34 = 0) INTO 92. RECODE grp (12 = 0) (3 = -1) (4 = 1) INTO g3. RECODE zsb (1 = RECODE zsb (13 -1) (2 = 0) (3 = 1) INTO z1. -1) (2 = 2) INTO Z2. COMPUTE gizl = g1*21. COMPUTE g1z2 = g1*22. Yol (15-16)-(12-96) COMPUTE g2z1 = g2*z1. COMPUTE g2z2 = g2*z2. 2 \Yeb - Yo =P DIF L 3 COMPUTE g3z1 = g3*z1. 155217 COMPUTE g3z2 = YG- You-16=PPIF 234 1 2. 34 14,479 g3*z2. 2 ? 14.479 2 154.740 3 REGRE /DEP = x3/ENTER g1 92 93 z1 z2 g1z1 TO g3z2 /SAVE PRED (prd1) REGRE/DEP = x3 /ENTER g1 g2 g3 z1 z2 /SAVE PRED (prd2). COMPUTE pdif = prdl - prd2. DESCR pdif. pdif 55AVB 2.71654(96-1) 1701.06 N Minimum Maximum Mean Std. Deviation 96-3.77 4.98 .0000 2.71654. *NOTE: listing of ONE case for each group. LIST o grp zsb g1 TO g3z2 x3 prd1 prd2 pdif /CASES FROM o grp zsb gl g2 g3 z1 z2 glzl glz2 g2z1 g2z2 g3z1 g322 x3. Main conly, eff, parallel TO 96 BY 8. prd2 pdif ssm ed not be o Yaz prdi 1 1 1 -1 1 0-1-1 1 1 1 1 0 0 41 51.12500 50.64583 ,4792 9 1 2 -1 -1 0 0 2 0 -2 0 -2 0 0 62 54.25000 17 1 3 -1 -1 0 1-1 -1 1 -1 1 0 0 60 55.62500 51.73958 58.61458 2.5104 -2.9896 40 2. 25 2 1 -1 1 0-1 -1 1 1 -1 -1 0 0 39 53.62500 48.64583 4.9792 S 33 2 2 -1 1 0 0 2 0 -2 0 2 0 0 53 47,50000 49.73958 -2.2396 -SSAR 41 2 3 -1 1 0 1-1 -1 1 1 -1 0 0 57 53.87500 56.61458 -2.7396 49 3 1 1 0-1-1-1 -1 -1 0 0 1 1 49 57 3 2 1 0-1 0 2 0 2 0 0 0 -2 59 13:2 65 3 3 1 0-1 1-1 1 -1 0 0 -1 1 43 73 4 1 1 0 1 -1 -1 -1 -1 0 0 -1 -1 59 81 A 2 1 0 1 0 2 0 2 0 0 0 2 47 89 4 3 1 0 1 1-1 1 -1 0 0 1 -1 55 51.62500 53.31250 53.50000 54.40625 63.87500 61.28125 50.50000 54.27083 56.00000 55.36458 65.37500 62.23958 -1.6875 -.9063 2.5938 -3.7708 .6354 3.1354 Grp 1 4 232 Az." 72-7 1 51.125 5362551625 50,5 51.319 -31021 2. -268 2. 5425 47.5 13.5 ngi 3 5555385 63,875 53.687 51.667 56.333 57.292 -1.073 -3.073 +1.893 42.352. 52.813 56 51927 65.775 59.688 +4.948 32 (-3.0211) Y( 1194.32 40.260082 1619538 552 (-1.73): 31.180727 predictor. It is there. comparisons to