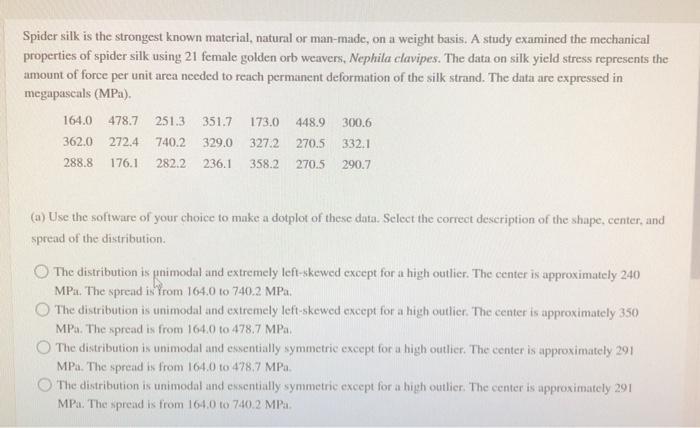
Spider silk is the strongest known material, natural or man-made, on a weight basis. A study examined the mechanical properties of spider silk using 21 female golden orb weavers, Nephila clavipes. The data on silk yield stress represents the amount of force per unit area needed to reach permanent deformation of the silk strand. The data are expressed in megapascals (MPa). 164.0 478.7 251.3 351.7 173.0 448.9 300.6 362.0 272.4 740.2 329.0 327.2 270.5 332.1 288.8 176.1 282.2 236.1 358.2 270.5 290.7 (a) Use the software of your choice to make a dotplot of these data. Select the correct description of the shape, center, and spread of the distribution. The distribution is unimodal and extremely left-skewed except for a high outlier. The center is approximately 240 MPa. The spread is from 164.0 to 740.2 MPa. The distribution is unimodal and extremely left-skewed except for a high outlier. The center is approximately 350 MPa. The spread is from 164.0 to 478.7 MPa. The distribution is unimodal and essentially symmetric except for a high outlier. The center is approximately 291 MPa. The spread is from 164.0 to 478.7 MPa. The distribution is unimodal and essentially symmetric except for a high outlier. The center is approximately 291 MPa. The spread is from 164.0 to 740.2 MPa. (b) Find the mean and median yield stress. (Enter the mean to two decimal places and the median to one decimal place.) Note: If you are using Crunchlt, you may adjust the default precision under Preferences. See the instructional video on how to adjust precision settings. x== M = A Compare these two values. Referring to your plot, what general fact does your comparison illustrate? The large outlier, as seen in the dotplot, is causing the mean to be greater than the median. The large outlier, as seen in the dotplot, is causing the mean to be less than the median. The approximately symmetric distribution, as seen in the dotplot, is causing the mean to be about the same as the median. O The approximately symmetric distribution, as seen in the dotplot, is causing the mean to be greater than the median.