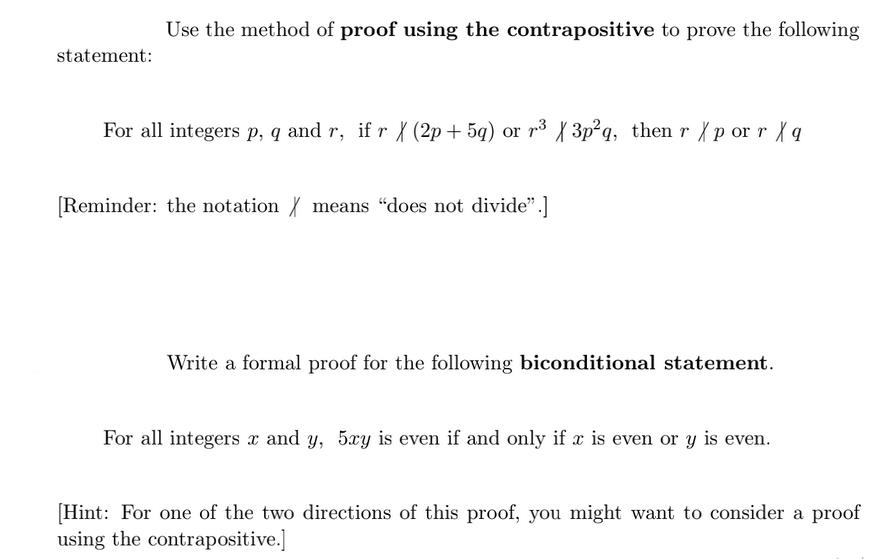
statement: Use the method of proof using the contrapositive to prove the following For all integers p, q and r, if r X (2p+5q) or r X 3pq, then r Xp or r Xq [Reminder: the notation means "does not divide".] Write a formal proof for the following biconditional statement. For all integers x and y, 5xy is even if and only if x is even or y is even. [Hint: For one of the two directions of this proof, you might want to consider a proof using the contrapositive.]