Statistics 7.2
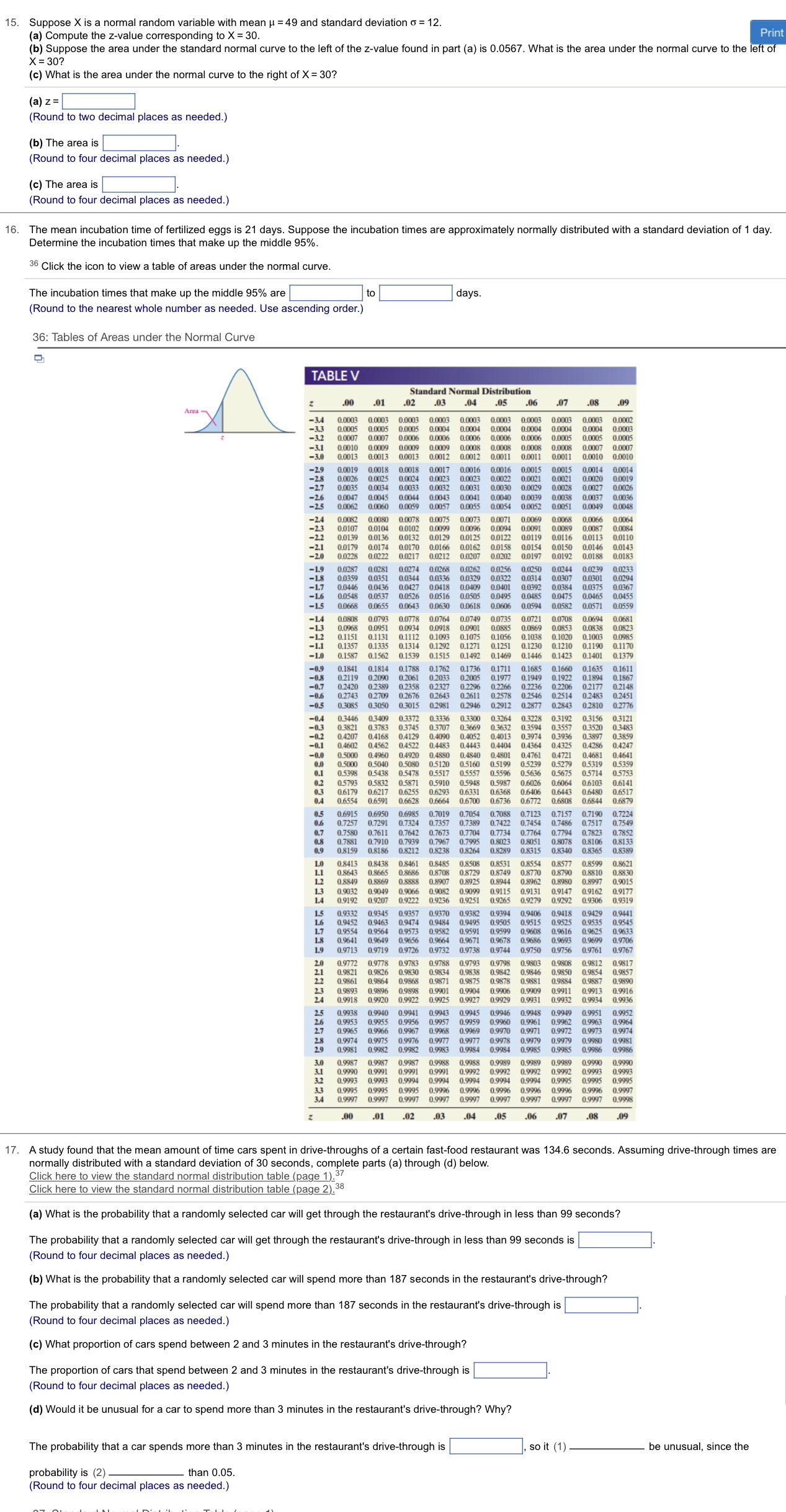
15. Suppose X is a normal random variable with mean u = 49 and standard deviation o = 12. a) Compute the z-value corresponding to X = 30. Print b) Suppose the area under the standard normal curve to the left of the z-value found in part (a) is 0.0567. What is the area under the normal curve to the left of X = 30? (c) What is the area under the normal curve to the right of X = 30? (a) z= (Round to two decimal places as needed.) (b) The area is Round to four decimal places as needed.) c) The area is Round to four decimal places as needed.) 16. The mean incubation time of fertilized eggs is 21 days. Suppose the incubation times are approximately normally distributed with a standard deviation of 1 day. Determine the incubation times that make up the middle 95% 36 Click the icon to view a table of areas under the normal curve. The incubation times that make up the middle 95% are to days (Round to the nearest whole number as needed. Use ascending order.) 36: Tables of Areas under the Normal Curve TABLE V Standard Normal Distribution 00 .01 .03 .04 .05 .07 .08 -3.4 0.0003 0 0905 0.0003 0.0003 0.0003 0.0003 0.0003 0.0003 0.0003 0. 0.0003 0.0002 -3.3 15 0.0005 0.0005 -3.2 0.0004 0.0004 0.0004 0.0004 0.0004 0.0004 0.0005 0.0007 0.0007 0.0006 0.0006 0.0006 0.0006 0.0006 0.0005 0.0005 0.0005 0.0010 0.0009 0.0009 9 0.0009 0.0008 0.0008 0.0008 0.0007 0.0007 -3.0 0.0013 0.0013 0.0013 0.0012 0.0012 0.0011 0.0011 0.0011 0.0010 0.0010 -2.9 0.0019 0.0018 0.0018 0.0017 0.0016 0.0015 0.0015 0.0014 0.0014 -2.8 0.0026 0.0025 0 0.0024 0.0023 0.0023 -27 0.0022 0.0021 0.0021 0.0020 0.0019 0.0035 0.0034 0.0033 0.0032 0.0031 0.0030 0.0029 0.0028 0.0027 0.0026 -2.6 0.0047 0.0045 0.0044 0.0043 0.0041 0.0040 0.0039 0.0038 0.0037 0.0036 -2.5 0.0062 0.0060 0.0059 0.0055 0.0054 0.0052 0.0051 0.0049 0.0048 -2.4 0.0082 0.0080 0 0.0078 0.0075 0.0073 0.0071 0.0069 0.0068 0.0066 0.0064 0.0107 0.0104 0.0102 0.0099 0.0096 0.0094 0.0091 0 0.0087 0.0139 0.0136 0.0129 0.0122 0.0119 0.0116 0.0113 0.0110 -2.1 0.0179 0.0174 0.0170 0.0166 0.0162 0.0158 0.0154 0.0150 0.0146 0.0143 -2.0 0.0228 0.0222 0.0217 0.0207 0.0202 0.0197 7 0.0192 0.0188 0.0183 -1.9 0.0287 0.0281 0.0274 0.0268 0.0262 0.0256 0.0250 0.0244 0.0239 0.0233 -1.8 0.0359 0.0351 0.0336 0.0329 0.0322 0.0314 0 0.0301 0.0294 -1.7 0.0446 0.0436 0.0418 0.0401 0.0392 0.0384 0.0367 -1.6 0.0548 0.0537 0.0526 0.0516 0.0505 0.0495 0.0485 0.0475 0.0465 0.0455 -1.5 0.0668 0.0655 0.0643 0.0630 0.0618 0.0606 0.0594 0.0582 0.0571 0.0559 -1.4 0.0808 0.0793 0.0778 0.0764 0.0749 0.0735 0.0721 0.0708 0.0694 0.0681 -1.3 0.0968 0.0951 0.0853 0.0838 -1.2 0.0823 0.1151 0.1131 0.1112 0.1093 0.1075 0.1056 0.1038 0 0.1357 0.1020 0.1003 0.0985 -1.1 0.1335 0.1314 0.1292 0.1271 0.1251 0.1230 0.1210 0.1190 0.1170 -1.0 0.1587 0.1562 0.1539 0.1515 0.1492 0.1469 0.1446 0.1423 0.1401 0.1379 0.1841 0.1814 0.1788 0.1762 0.1736 0.1711 0.1685 0.1660 0.1635 0.1611 0.2119 0.2090 0.2061 0.2033 0.1977 0.1949 0.1894 0.1867 0.2420 0.2389 0.2358 0.2327 0.2296 0.2236 0.2206 0.2177 0.2148 -0.6 0.2743 0.2709 0.2676 0.2643 0.2611 0.2578 0.2546 0.2514 0 0.2483 0.2451 0.3085 0.3050 0 0.3015 0.2981 0.2912 0.2877 0.2843 0.2810 0.2776 0.3446 0.3409 0.3372 0.3336 0.3264 0.3228 8 0.3192 0.3156 0.3121 0.3821 0.3783 0 0.3745 0.3707 0.3669 0.3632 0.3594 0.3557 0.3483 -0.2 0.4207 0.4168 0.4129 0.4090 0.4052 0.4013 0.3974 0.3936 0.3897 0.3859 -0.1 0.4602 0.4562 0.4522 0.4483 0.4443 0.4404 0.4364 0.4325 0.4286 0.4247 -0.0 0.5000 0.4960 0.4880 0.4641 0.0 0.5000 0.5040 0.5120 0.5160 0.5199 0.5239 0.5279 0.5319 0.5359 0.1 0.5398 0.5478 0.5517 0 0.5557 0.5596 0.2 0.5636 0.5675 0.5714 0.5753 0.5793 0.5832 0.5871 0.5910 0.5948 0.5987 60.6064 0.6103 0.6141 0.3 0.6179 0.6217 0.6293 0.6331 0.6368 0.6443 0.4 0.6517 0.6554 0.6591 0.6628 0.6664 0.6700 0.6736 0.6772 0.6808 0.6844 0.6879 0.5 0.6915 0.6950 0.6985 0.7019 0.7088 0.7157 0.7190 0.7224 0.6 0.7257 0.7291 0 0.7324 0.7357 0.7389 0.7 0.7422 0.7454 0.7486 0.7517 0.7549 0.7580 0.7611 0.7642 0.7673 0.7704 0.7734 0.7764 0.7794 0.7823 0.7852 0.8 0.7881 0.7910 0.8023 0.8051 0.8106 0.8133 0.9 0.8159 0.8186 0.8212 0.8238 0.8264 0.8289 0.8315 0.8340 0.8365 0.8389 1.0 0.8413 0.8485 0.8508 0.8531 0.8554 0.8577 0.8599 0.8621 0.8643 0.8665 0.8708 0.8729 0.8749 0.8770 1.2 0.8790 0.8810 0.8849 0.8869 0.8907 0.8925 0.8944 0.8962 0.8997 0.9015 1.3 0.9032 0.9049 0.9082 0.9099 0.9115 0.9131 0.9147 0.9162 0.9177 1.4 0.9192 0.9207 0.9222 0.9236 0.9251 0.9265 0.9279 0.9292 0.9306 0.9319 1.5 0.9332 0.9345 0.9357 0.9370 0.9382 0.9394 0.9406 0.9418 0.9429 0.9441 1.6 0.9452 0.9463 0.9474 0.9484 0.9495 0.9505 0.9515 0.9525 0.9535 0.9545 1.7 0.9554 0.9582 0.9591 0.9608 0.9616 0.9633 1.8 0.9641 0.9649 0.9656 0.9664 0.9671 0.9678 0.9686 0.9693 0.9699 0.9706 1.9 0.9713 0.9719 0.9726 0.9732 0. 0.9738 0.9744 0.9750 0.9756 0.9761 0.9767 20 0.9772 0.9778 0.9783 0.9788 0.9793 0.9798 21 0.9803 0.9808 0.9812 0.9817 0.9821 0.9826 0.9834 22 0.9842 0.9846 0.9850 0.9854 0.9857 0.9871 0.9878 0.9881 0.9890 23 0.9893 0.9896 0.9898 0.9901 0.9904 0.9906 0.9909 0.9911 0.9913 0.9916 24 0.9918 0.9920 0.9922 0.9925 0.9927 0.9929 0.9931 0.9934 0.9936 0.9938 0.9940 0.9941 0.9943 0.9945 0.9946 0.9948 0.9949 0.9951 0.9952 26 0.9953 0.9955 0.9956 27 0.9957 0.9959 0.9960 0.9961 0.9962 0.9963 0.9964 0.9965 0.9966 0.9967 0.9968 0.9970 0.9971 0.9972 0.9974 2.8 0.9974 0.9975 0.9976 0.9977 0.9977 0.9978 0.9979 0.9979 0.9980 0.9981 2.9 0.9981 0.9982 0.9982 0.9983 0.9984 0.9984 0.9985 0.9985 0.9986 0.9986 3.0 0.9987 0.9987 0.9987 0.9988 0.9988 3.1 0.9989 0.9989 0.9989 0.9990 0.9990 0.9990 0.9991 0.9991 0.9991 0.9992 0.9992 0.9992 0.9993 3.2 0.9993 0.9993 0.9994 0.9994 0.9994 0.9994 0.9995 0.9995 0.9995 3.3 34 0.9995 0.9995 0.9995 0.9996 0.9996 0.9996 0.9996 0.9996 0.9996 0.9997 0.9997 0.9997 0.9997 0.9997 0.9997 0.9997 0.9997 0.9997 0.9997 0.9998 .01 .02 .03 .04 .05 .06 .07 17. A study found that the mean amount of time cars spent in drive-throughs of a certain fast-food restaurant was 134.6 seconds. Assuming drive-through times are normally distributed with a standard deviation of 30 seconds, complete parts (a) through (d) below. Click here to view the standard normal distribution table (page 1). 37 Click here to view the standard normal distribution table (page 2),38 (a) What is the probability that a randomly selected car will get through the restaurant's drive-through in less than 99 seconds? The probability that a randomly selected car will get through the restaurant's drive-through in less than 99 seconds is (Round to four decimal places as needed.) (b) What is the probability that a randomly selected car will spend more than 187 seconds in the restaurant's drive-through? The probability that a randomly selected car will spend more than 187 seconds in the restaurant's drive-through is (Round to four decimal places as needed. (c) What proportion of cars spend between 2 and 3 minutes in the restaurant's drive-through? The proportion of cars that spend between 2 and 3 minutes in the restaurant's drive-through is (Round to four decimal places as needed.) (d) Would it be unusual for a car to spend more than 3 minutes in the restaurant's drive-through? Why? The probability that a car spends more than 3 minutes in the restaurant's drive-through is so it (1) be unusual, since the probability is (2) than 0.05. (Round to four decimal places as needed.)








