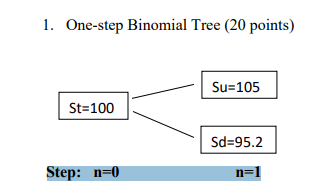
Step: n=0 n=1

Consider the above one-step binomial tree, with each step being three months, =0.25. The stock price is shown on the tree. The annual risk-free rate is 5%. There is no dividend from the stock.

1) Consider an at-the-money European call option (S=K) with a three-month maturity

(a) What are the possible stock prices at the call option expiration under the binomial model?

(b) What is the payoff to this call option at the expiration date at each possible stock price?

(c) Calculate the value of the call option today

(d) Calculate the delta of this call option

(e) Suppose a market maker makes the call option at $3.5, with zero bid-ask spread. You can trade the stock at $100 without transaction cost, and the bond rate is 5%. Can you do arbitrage trading to lock in money under the binomial assumption? How?

2) Consider a European put option with a strike price of 105 and with a three-month maturity.

(a) What is the payoff of the put option at the expiration of each possible stock price?

(b) Calculate the put option fair value today.

(c) Calculate the delta of this put option

(d) Construct a delta-neutral portfolio based on the stock and put option.

1. One-step Binomial Tree ( 20 points) Step: n=0 n=1