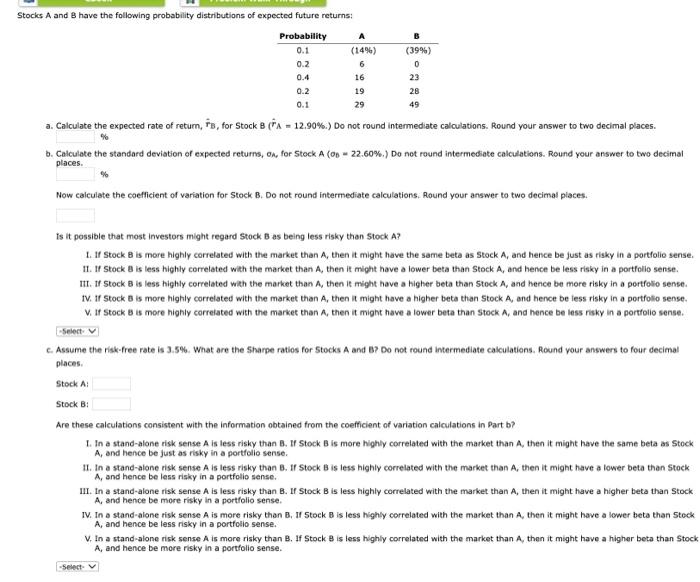
Stocks A and B have the following probsbility distributions of expected future returns: a. Calculate the expected rate of retum, rB, for Stock B(rA=12.90%.) Do not round intermediate calculations. Round your answer to two decimal places. \% b. Calculate the standard deviation of expected returns, A for 5 tock A(OB=22.60%.) Do not round intermediate calculations. Round your answer to two decimal Dtaces. \% Now calculate the coefficient of variation for Stock B. Do not round intermediate calculations. Round your answer to two decimal places. Is it possible that most investors might regard stock B as being less risky then $ tock A ? 1. If Stock B is more highly correlated with the market than A, then it might have the same beta as stock A, and hence be just as risky in a portfolie sense. 1I. If Stock B is less highly correlated with the market than A, then it might have a lower beta than Stock A, and hence be less risky in a portfolio sense. III. If Stock B is less highly correlated with the market than A, then it might have a higher beta than Stock A, and hence bee more risky in a portfolio sense. TV. If stock B is more highly correlated with the market than A, then it might have a higher beta than Stock A, and hence be less risky in a portfolio sense. V. If Stock B is more highly correiated with the market than A, then it might have a lower beta than stock A, and hence be less risky in a portfolio sense. c. Assume the risk-free rate is 3.5%. What are the Sharpe ratios for 5 tocks A and B? Do not round intermediate calculations. Round your answers to four decimal places. Stock A: Stock B: Are these calculations consistent with the information obtained from the coefficient of variation calculations in Part b? 1. In a stand-alone risk sense A is less risky than B. If Stock B is more highiy correlated with the market than A, then it might have the same beta as Stock A, and hence be just as risky in a portfolio sense. 1I. In a stand-alone risk sense A is less risky than B. If Stock B is less highly correlated with the market than A, then it might have a lower beta than stock A, and hence be less risky in a portfolio sense. III. In a stand-alone risk sense A is less risky than B. If Stock B is less highly correlated with the market than A, then it might have a higher beta than Stock A, and hence be more risky in a portfolio sense. IV. In a stand-alone risk sense A is more risky than B. If 5 tock B is less highily correlated with the market than A, then it might have a lower beta than Stock A, and hence be less risky in a portfolio sense. V. In a stand-alone risk sense A is more risky than B. If Stock B is less highly correlated with the market than A, then it might have a higher beta than Stock A, and hence be more risky in a portfolio sense. You have been managing a $5 million portfolio that has a beta of 1.35 and a required rate of return of 13.775%. The current risk-free rate is 5%. Assume that another $500,000. If you invest the money in a stock with a beta of 1.65, what will be the required return on your $5.5 million portfolio? Do not round interm calculations. Round your answer to two decimal places. %