Subject: Stochastic Process

Chapter: Markov Chain

A Markov chain is said to be a tree process if

(i) ?Pij?>0? whenever ?Pji?>0? ,

(ii) for every pair of states ?i? and ?j?, ?i??=j? , there is a unique sequence of distinct states ?i=i0?,i1?,...,in?1?,in?=j? such that

?Pik?,ik+1??>0,k=0,1,...,n?1?

In other words, a Markov chain is a tree process if for every pair of distinct states i and j there is a unique way for the process to go from i to j without reentering a state (and this path is the reverse of the unique path from j to i).

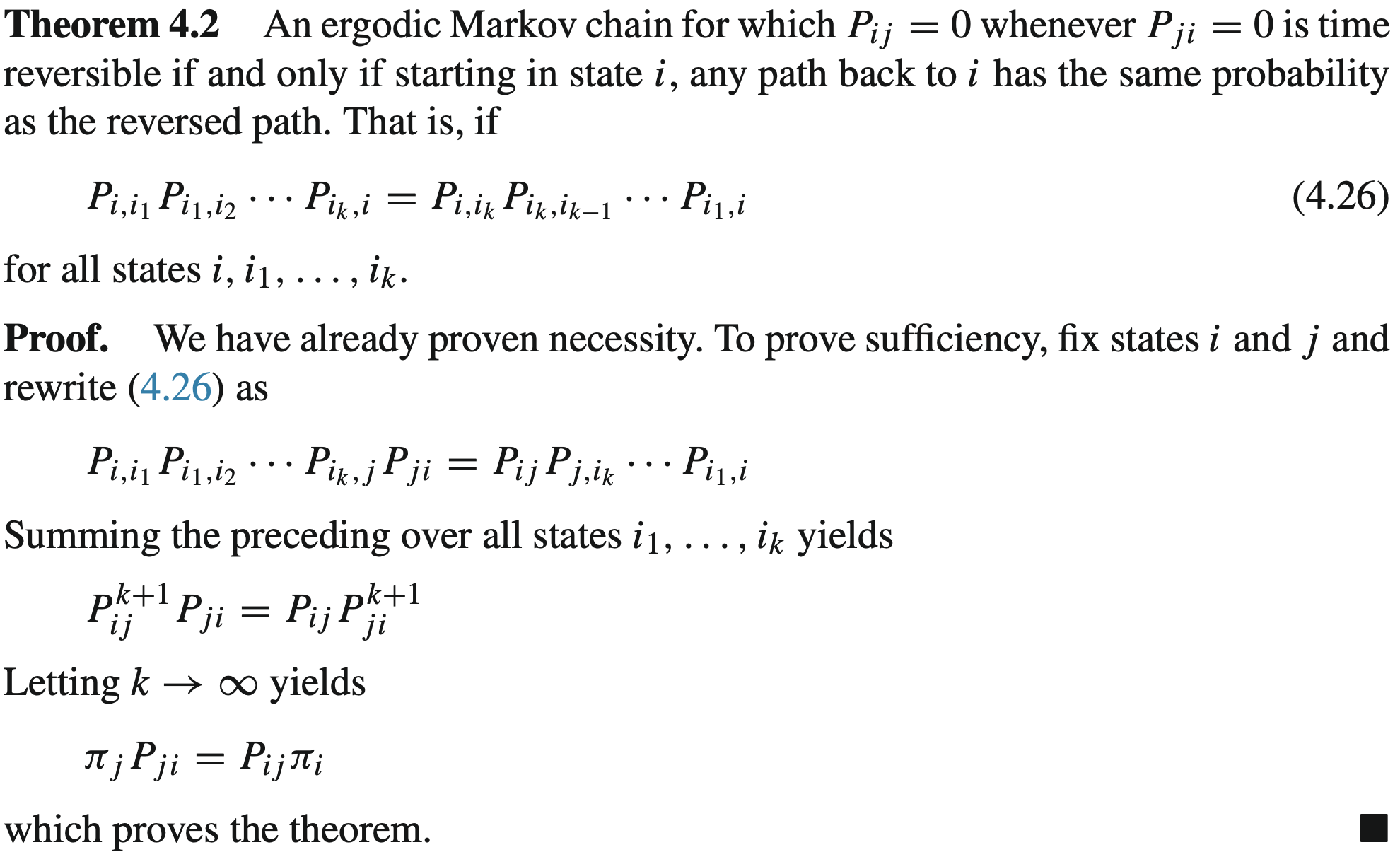
Question: Argue that an ergodic tree process is time reversible.

(According to my teaching assistant, the hint is below: (image attached))

Theorem 4.2 An ergodic Markov chain for which Pij = 0 whenever Pji = 0 is time reversible if and only if starting in state i, any path back to i has the same probability as the reversed path. That is, if Pi,i Pin,iz . . . Pik,i = Pi,ik Pik,ik-1 . . . Pi,i (4.26) for all states i, i1, . . ., ik. Proof. We have already proven necessity. To prove sufficiency, fix states i and j and rewrite (4.26) as Pi,i Pi,iz . . . Pix,j Pji = Pij Pj,ik . . . Pin,i Summing the preceding over all states i 1, . .., ik yields phel Pji = Pijpji Letting k -> co yields nj Pji = PijTi which proves the theorem