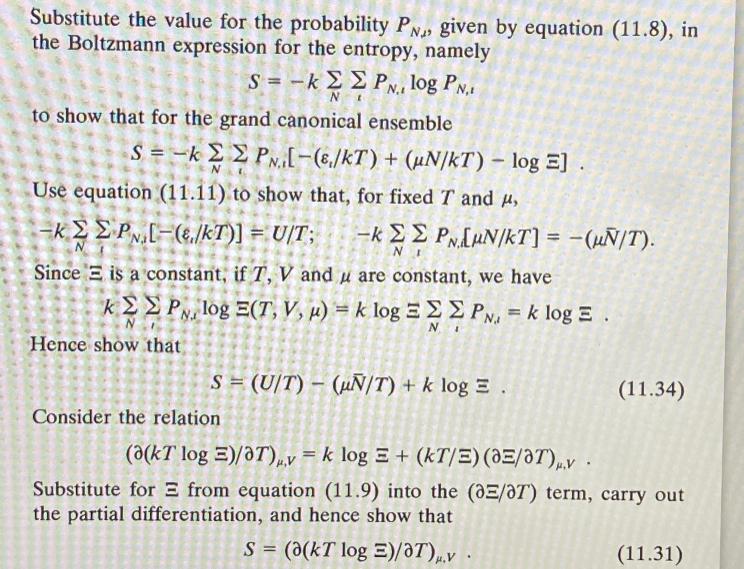
Substitute the value for the probability PN given by equation (11.8), in the Boltzmann expression for the entropy, namely S=-kP., log PN.1 N to show that for the grand canonical ensemble S=-kPN-(e/kT) + (uN/KT) - log E]. Use equation (11.11) to show that, for fixed T and , -k PN [-(e./kT)] = U/T; -k PNLUN/KT] = -(/T). N Since E is a constant, if T, V and u are constant, we have kP, log E(T, V, ) = k log = PN. = k log E. NI Hence show that S = (U/T) (N/T) + k log E. Consider the relation 201 (11.34) (a(kT log E)/OT),v = k log E+ (kT/E) (OE/OT),v. Substitute for from equation (11.9) into the (0E/OT) term, carry out the partial differentiation, and hence show that S = (a(kT log E)/OT).v. (11.31)