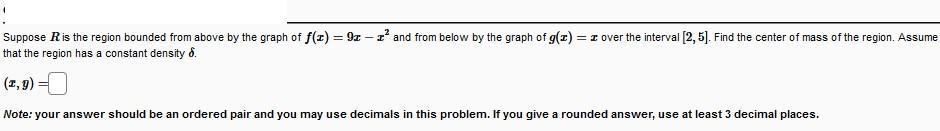
Suppose R is the region bounded from above by the graph of f(x) = 9x - z and from below by the graph of g(z) = z over the interval [2, 5]. Find the center of mass of the region. Assume that the region has a constant density 6. (z,y) Note: your answer should be an ordered pair and you may use decimals in this problem. If you give a rounded answer, use at least 3 decimal places.