Question
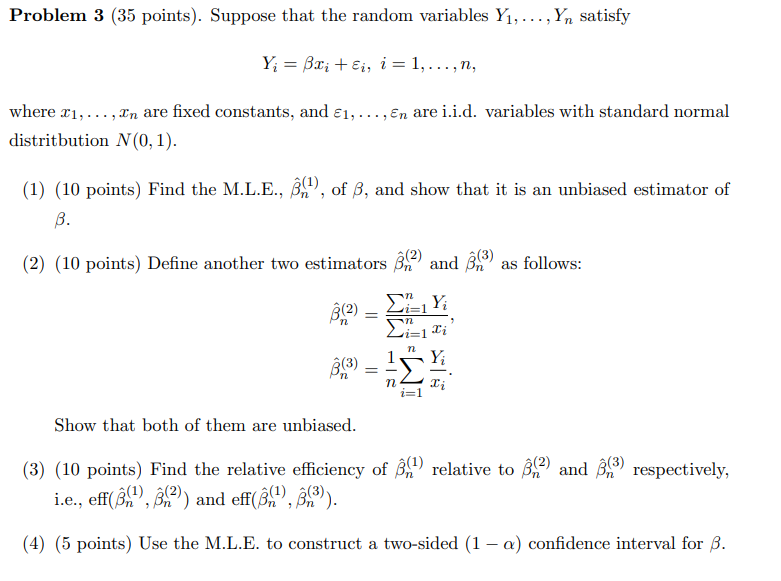
. Suppose that the random variables Y1, . . . , Yn satisfy Yi = xi + i , i = 1, . . .
. Suppose that the random variables Y1, . . . , Yn satisfy
Yi = xi + i , i = 1, . . . , n,
where x1, . . . , xn are fixed constants, and 1, . . . , n are i.i.d. variables with standard normal distritbution N(0, 1).
(1) (10 points) Find the M.L.E., n (1) , of , and show that it is an unbiased estimator of .
(2) (10 points) Define another two estimators (2) n and (3) n as follows:
(2) n = Pn i=1 P /Yi n i=1 xi , (3) n = 1 *Xn i=1 Yi/ xi .
Show that both of them are unbiased.
(3) (10 points) Find the relative efficiency of (1) n relative to (2) n and (3) n respectively, i.e., eff( (1) n , (2) n ) and eff( (1) n , (3) n ).
(4) (5 points) Use the M.L.E. to construct a two-sided (1 ) confidence interval for .
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started