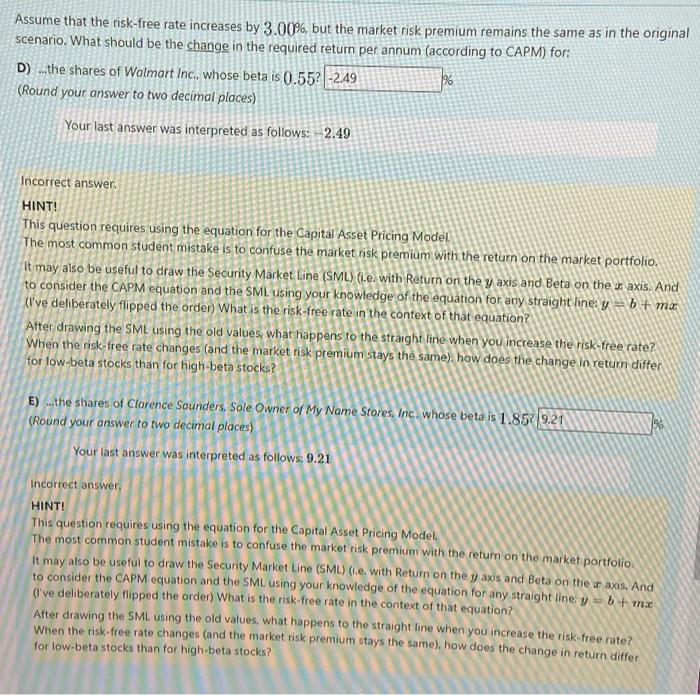
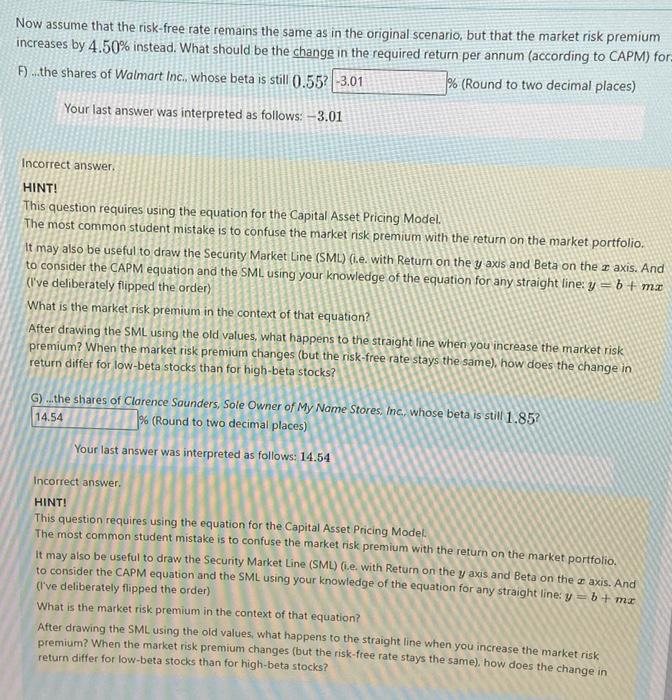
Suppose that the risk-free rate is 5.10% per annum and that the market risk premium is 9.00% per annum. Assume that you are considering buying shares of Piggly Wiggly LLC. Each share of Piggly Wiggly is currently priced at $109.85 and is expected to pay $14.21 as its next dividend to shareholders one year from today. These dividends grow by 2.60% per annum. A) Based off the current market price of shares of Piggly Wiggly, what return do investors expect to earn per annum by investing in the firm's stock? 15.54 % (Round to two decimal places for this answer, but use unrounded value in subsequent questions if needed) Your last answer was interpreted as follows: 15.54 Correct answer, well done. B) What would the beta of this firm's stock need to be in order for current market expectations to be consistent with CAPM? 1.16 (Round to two decimal places for this answer) Your last answer was interpreted as follows: 1.16 Correct answer, well done. 1% C) If the beta of Piggly Wiggly LLC should actually be 1.51. then according to the Capital Asset Pricing Model by what percentage are shares of the firm currently overpriced or underpriced? 44.27 (Round to two decimal places for this answer. A positive answer implies overpriced and a negative answer implies underpriced) Your last answer was interpreted as follows: 44.27 Incorrect answer. HINT! This question requires using the equation for the Capital Asset Pricing Model The most common student mistake is to confuse the market risk premium with the return on the market portfolio. In order to calculate how overpriced or underpriced something is as a percentage, you compare the actual price of that thing to what its price should be. For example, if an apple costs $0.80/apple but it should cost $1.00/apple ...whatever should means in this context), then that apple is priced -20% compared to what it should be i.e. it is 20% underpriced). Assume that the risk-free rate increases by 3.00%, but the market risk premium remains the same as in the original scenario. What should be the change in the required return per annum (according to CAPM) for: D) .the shares of Walmart Inc., whose beta is 0.552 -2.49 % (Round your answer to two decimal places) Your last answer was interpreted as follows: 2.49 Incorrect answer. HINT! This question requires using the equation for the Capital Asset Pricing Model The most common student mistake is to confuse the market nisk premium with the return on the market portfolio. It may also be useful to draw the Security Market Line (SML) (1.e. with Return on the y axis and Beta on the axis. And to consider the CAPM equation and the SML using your knowledge of the equation for any straight line: y = b + max (I've deliberately flipped the order) What is the risk-free rate in the context of that equation? After drawing the SML using the old values, what happens to the straight line when you increase the risk-free rate? When the risk-free rate changes (and the market risk premium stays the same), how does the change in return differ for low-beta stocks than for high-beta stocks? E) the shares of Clorence Saunders. Sole Owner of My Name Stores, Inc., whose beta is 1.852 9.21 (Round your answer to two decimal places) Your last answer was interpreted as follows: 9.21 196 Incorrect answer, HINT! This question requires using the equation for the Capital Asset Pricing Model The most common student mistake is to confuse the market risk premium with the return on the market portfolio It may also be useful to draw the Security Market Line (SML) (e. with Return on the y axis and Beta on the ar axis. And to consider the CAPM equation and the SML using your knowledge of the equation for any straight line y - 6+ mx (I've deliberately flipped the order) What is the risk-free rate in the context of that equation? After drawing the SML using the old values, what happens to the straight line when you increase the risk-free rate? When the risk-free rate changes (and the market risk premium stays the same), how does the change in return differ for low-beta stocks than for high-beta stocks? Now assume that the risk-free rate remains the same as in the original scenario, but that the market risk premium increases by 4.50% instead. What should be the change in the required return per annum (according to CAPM) for- F) ..the shares of Walmart Inc., whose beta is still 0.557 -3.01 % (Round to two decimal places) Your last answer was interpreted as follows: 3.01 Incorrect answer. HINT! This question requires using the equation for the Capital Asset Pricing Model The most common student mistake is confuse the market risk premium with the return on the market portfolio. It may also be useful to draw the Security Market Line (SML) (1.e. with Return on the y axis and Beta on the 3 axis. And to consider the CAPM equation and the SML using your knowledge of the equation for any straight line: y = m. (I've deliberately flipped the order) What is the market risk premium in the context of that equation? After drawing the SML using the old values, what happens to the straight line when you increase the market risk premium? When the market risk premium changes (but the risk-free rate stays the same), how does the change in return differ for low-beta stocks than for high-beta stocks? G) ...the shares of Clarence Sounders, Sole Owner of My Name Stores, Inc., whose beta is still 1.85? % (Round to two decimal places) 14.54 Your last answer was interpreted as follows: 14.54 Incorrect answer HINT! This question requires using the equation for the Capital Asset Pricing Model The most common student mistake is to confuse the market risk premium with the return on the market portfolio it may also be useful to draw the Security Market Line (SML) (e. with Return on the y axis and Beta on the or axis. And to consider the CAPM equation and the SML using your knowledge of the equation for any straight line: y = b + mx (I've deliberately flipped the order) What is the market risk premium in the context of that equation? After drawing the SML using the old values, what happens to the straight line when you increase the market risk premium? When the market risk premium changes (but the risk-free rate stays the same), how does the change in return differ for low-beta stocks than for high-beta stocks