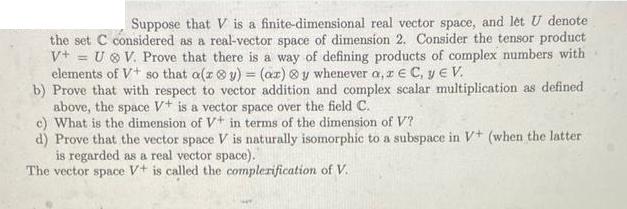
Suppose that V is a finite-dimensional real vector space, and let U denote the set C considered as a real-vector space of dimension 2. Consider the tensor product V+ = UV. Prove that there is a way of defining products of complex numbers with elements of V+ so that a(z y) = (az) y whenever a, z EC, y V. b) Prove that with respect to vector addition and complex scalar multiplication as defined above, the space V+ is a vector space over the field C. c) What is the dimension of V+ in terms of the dimension of V? d) Prove that the vector space V is naturally isomorphic to a subspace in V+ (when the latter is regarded as a real vector space). The vector space V+ is called the complerification of V.