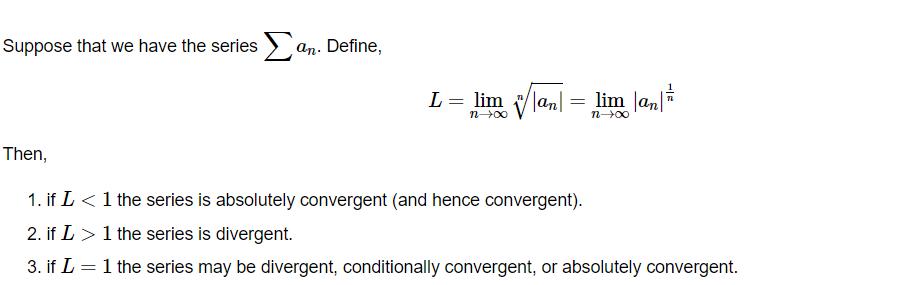
Suppose that we have the series an. Define, Then, L = lim an = lim an n0 1. if I < 1 the series is absolutely convergent (and hence convergent). 2. if L> 1 the series is divergent. 3. if L = 1 the series may be divergent, conditionally convergent, or absolutely convergent. For the following function, find the full power series centered at x = 0 and then give the first 5 nonzero terms of the power series and the open interval of convergence. (Hint: Use the Law of Logs: In(uv) = ln(u) + In(v).) f(x) = + O n=1 =0+0+0+0+0+ f(x): The open interval of convergence is: f(x) = ln(6 + 5x) (Give your answer in interval notation.)