Question: Suppose that X, ..., Xn are independent and identically distributed Poisson(X) random variables with probability function Define X and S as f(x; ) =

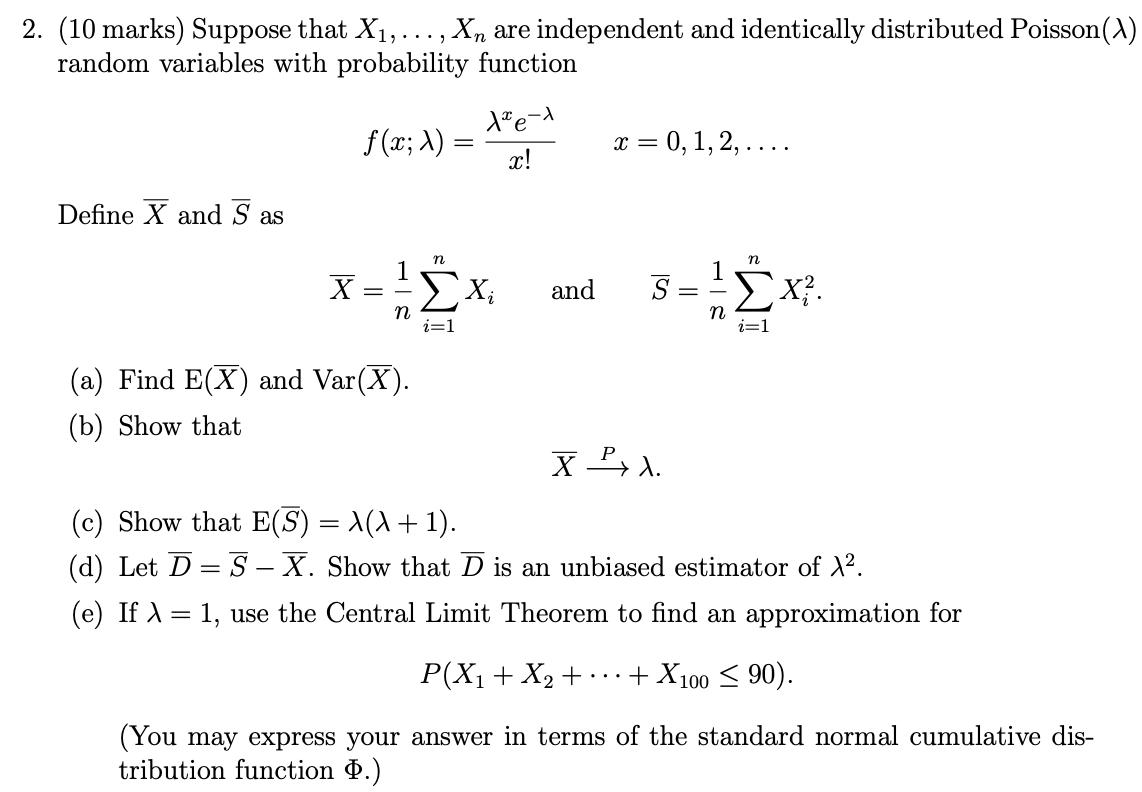
Suppose that X, ..., Xn are independent and identically distributed Poisson(X) random

Suppose that X, ..., Xn are independent and identically distributed Poisson(X) random variables with probability function Define X and S as f(x; ) = = n (a) Find E(X) and Var(X). (b) Show that Xe-1 x! X and x = 0, 1, 2, .... XX. = X. (c) Show that E(S) = X(X + 1). (d) Let D = S-X. Show that D is an unbiased estimator of X. (e) If = 1, use the Central Limit Theorem to find an approximation for P(X + X + + X100 90). (You may express your answer in terms of the standard normal cumulative dis- tribution function .)

Step by Step Solution

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

The detailed ... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

Suppose your grade in a probability course depends on 10 weekly quizzes. Each quiz has ten yes/no questions, each worth 1 point. The scoring has no partial credit. Your performance is a model of...

The ping time, in milliseconds, of a new transmission system, described in Problem 11.1.4 is the exponential (60) random variable N. The ping time of an old system is the exponential (120) random...

Suppose you want to take a sample of students in a university but your sampling frame is a list of all classes offered by the university. A student may be in more than one class, so a probability...

14. In Springfield, Massachusetts, people drive their cars to a state inspection center for annual safety and emission certification at a Poisson rate of . For n 0, if there are n cars at the center...

The daily closing price for a certain stock issue on the NYSE can be represented as the outcome of \(Y_{t}=Y_{t-1}+V_{t}\), where \(y_{t}\) is the value of the stock price on day \(t\), and \(V_{t}...

If X1, X2, . . . ,Xn are independent and identically distributed random variables having uniform distributions over (0, 1), find (a) E[max(X1, . . . ,Xn)]; (b) E[min(X1, . . . ,Xn)].

Let X1, . . . ,Xn be independent and identically distributed random variables. Find E[X1|X1 + + Xn = x]

31. Let X 1 , . . ., X n be independent and identically distributed random variables having distribution function F and density f. The quantity M = [X (1) + X (n) ]/2, defined to be the average of...

Let X1, X2,..., Xn denote independent and identically distributed random variables and define the order statistics X(1),..., X(n) by X(i) ith smallest of X1,..., Xn Show that if the distribution of...

66. Use Chebyshevs inequality to prove the weak law of large numbers. Namely, if X1, X2, . . . are independent and identically distributed with mean and variance 2 then, for any > 0, | X + X2 + +...

CMPU Hospitals, Inc. is increasingly worried about ransomware attacks. The response to ransomware attacks vary. Some companies have no choice but to pay the ransom, while others with good business...

Lead(IV) oxide forms the cathode of lead storage batteries. How is this substance produced for these batteries?

AP2.4 Consider a thermal heating system given by where the output 1s2 is the temperature difference due to the thermal process, the input q1s2 is the rate of heat flow of the heating element. The...

Why are black hat SEO techniques considered unethical? Who is harmed by use of these techniques? Are there consequences?

Fruit traders in Indramayu (place) sell mangoes from their gardens in 6 fruit shops in their area. Traders want to know the price elasticity of mango demand. The six fruit shops are in middle-income...

A monopolist faces a demand for Q = 18-2P where Q is the number/quantity of goods demanded (units). Monopolists have a constant average cost (AC) = 3 per unit. Question: a. From the information...

Let t n, t n + 1 , t n + 2 (all positive) be any 3 consecutive terms of an arbitrary geometric progression. Prove that t n + 1 is the Geometric Mean of t n and t n + 2.

The illustrated structure is affected by a known couple, and try to figure out the restraining reaction force of the hinge A and hinge E. a m D. A a

my 10 year old brother bought plane tickets on accident. I canceled the flight and did not get my money back. I reported it as fraud with the bank to get my money back. Is this bad?>

Suppose that X1, ...; Xn are independent and identically distributed Poisson(A) ran- dom variables with probability function f (I; A) = r = 0, 1, 2, ... Define X and S as X =. _EX; and 5= 15X?. i=1...

1. Suppose that X1,.... Xn are independent and identically distributed Poisson(A) ran- dom variables with probability function f(z; A) = r = 0, 1,2. . .. Define X and S as X =_) X; and =_ i=1 i=1 (a)...

2. Simulation study Suppose that X1, . . ., Xn are independent and identically distributed (iid) binomial random variables such that P(Xi = = | k, P) = (x) D (1 _ p)* z, x =0,1, ..., k for all i = 1,...

2. Simulation study Suppose that X1, . ..; Xn are independent and identically distributed (iid) binomial random variables such that P(Xi = 1 | k, P) = (x)p (1 -p)* z, x =0,1, ..., k for all i = 1,...