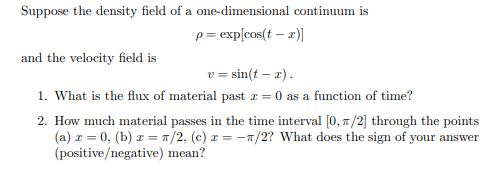
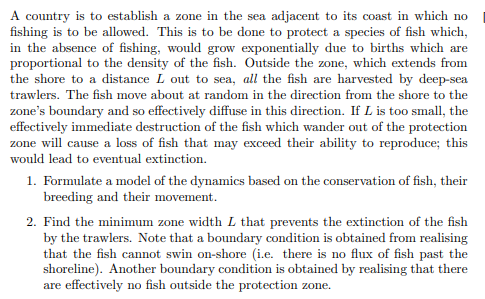
Suppose the density field of a one-dimensional continuum is p = exp[cos(t-x)] and the velocity field is v = sin(t - x). 1. What is the flux of material past z = 0 as a function of time? 2. How much material passes in the time interval [0,1/2] through the points I= (a) x = 0, (b) x = /2, (c) x = -1/2? What does the sign of your answer (positive/negative) mean? A country is to establish a zone in the sea adjacent to its coast in which no fishing is to be allowed. This is to be done to protect a species of fish which, in the absence of fishing, would grow exponentially due to births which are proportional to the density of the fish. Outside the zone, which extends from the shore to a distance L out to sea, all the fish are harvested by deep-sea trawlers. The fish move about at random in the direction from the shore to the zone's boundary and so effectively diffuse in this direction. If L is too small, the effectively immediate destruction of the fish which wander out of the protection zone will cause a loss of fish that may exceed their ability to reproduce; this would lead to eventual extinction. 1. Formulate a model of the dynamics based on the conservation of fish, their breeding and their movement. 2. Find the minimum zone width L that prevents the extinction of the fish by the trawlers. Note that a boundary condition is obtained from realising that the fish cannot swin on-shore (i.e. there is no flux of fish past the shoreline). Another boundary condition is obtained by realising that there are effectively no fish outside the protection zone.