
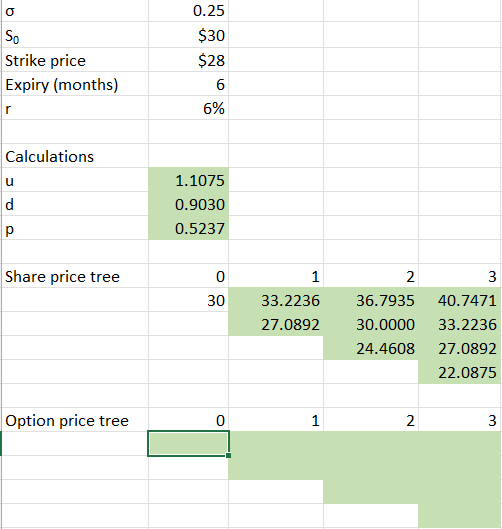
Suppose the price today for a share in XYZ is $30. It is known that the price for XYZ shares has a volatility of =0.25 per year. We would like to price a European put option with a strike price of $28 expiring in 6 months' time. The continuous rate of return is 6% per annum. These inputs have been included in your template spreadsheet. We will build a binomial price tree using 3 time steps ( 1 step=2 months). From the given data, first calculate: - The ratios u and d for an upward and downward movement in the share price respectively implied by the information. - The corresponding probability p of an upward movement in the share price. Enter your results in cells B8 to B10. Using these results, fill in the binomial tree for the share price in cells C13 to E16. Finally, in cells B19-E22, fill in the option value tree using appropriate formulas. The entry in cell B19 should be the value of the put option today. \begin{tabular}{|l|r|} \hline & 0.25 \\ \hline S0 & $30 \\ \hline Strike price & $28 \\ \hline Expiry (months) & 6 \\ \hline r & 6% \\ \hline \end{tabular} \begin{tabular}{|l|r|r|r|r|} \hline Calculations & & & & \\ \hlineu & 1.1075 & & & \\ \hlined & 0.9030 & & & \\ \hlinep & 0.5237 & & & \\ \hline d & & & & \\ \hline & 0 & 1 & 2 & \\ \hline & 30 & 33.2236 & 36.7935 & 40.7471 \\ \hline & & 27.0892 & 30.0000 & 33.2236 \\ \hline & & & 24.4608 & 27.0892 \\ \hline & & & & 22.0875 \\ \hline & & & \\ \hline \end{tabular} Option price tree Suppose the price today for a share in XYZ is $30. It is known that the price for XYZ shares has a volatility of =0.25 per year. We would like to price a European put option with a strike price of $28 expiring in 6 months' time. The continuous rate of return is 6% per annum. These inputs have been included in your template spreadsheet. We will build a binomial price tree using 3 time steps ( 1 step=2 months). From the given data, first calculate: - The ratios u and d for an upward and downward movement in the share price respectively implied by the information. - The corresponding probability p of an upward movement in the share price. Enter your results in cells B8 to B10. Using these results, fill in the binomial tree for the share price in cells C13 to E16. Finally, in cells B19-E22, fill in the option value tree using appropriate formulas. The entry in cell B19 should be the value of the put option today. \begin{tabular}{|l|r|} \hline & 0.25 \\ \hline S0 & $30 \\ \hline Strike price & $28 \\ \hline Expiry (months) & 6 \\ \hline r & 6% \\ \hline \end{tabular} \begin{tabular}{|l|r|r|r|r|} \hline Calculations & & & & \\ \hlineu & 1.1075 & & & \\ \hlined & 0.9030 & & & \\ \hlinep & 0.5237 & & & \\ \hline d & & & & \\ \hline & 0 & 1 & 2 & \\ \hline & 30 & 33.2236 & 36.7935 & 40.7471 \\ \hline & & 27.0892 & 30.0000 & 33.2236 \\ \hline & & & 24.4608 & 27.0892 \\ \hline & & & & 22.0875 \\ \hline & & & \\ \hline \end{tabular} Option price tree








