Suppose there are three possible states of the market, j=1 (good), j=2 (average), j = 3 (bad). Each R_j^B and R_j^C, are given in the following table:

State (j)	Pj	RjA	RjB	RjC
1	1/3	0.34	0.20	0.10
1	1/3	0.10	0.10	0.10
1	1/3	-0.10	0.00	0.10

From the table we see that asset C is a riskless asset with a sure return of 10%. Also, let the utility

function for the investor be U(R) = ln(1+R) where ln represents the natural log and R is the realized

return of an asset or portfolio.

a. Calculate the expected return and standard deviation associated with each asset.

b. Rank the three portfolios from least to most risky using an appropriate measure.

c. Show that for assets A and B, an investor always prefers a sure payment equal to the expected

return of that asset rather than holding the asset itself. For asset C, show that the investor would

be indifferent. Recall that as discussed in lecture, the expected utility associated with holding a

particular asset k = A, B, C is given by

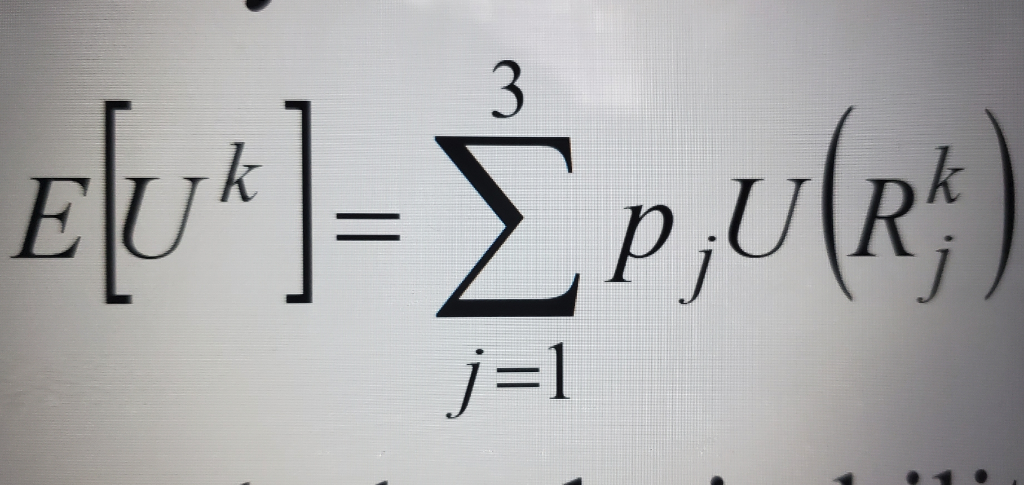
d. Given your calculations in part c, rank the desirability of each asset according to the preferences

of the investor.

e. Suppose that a fourth asset, Asset D, is available and is a portfolio consisting of equal shares of

Assets A, B and C. Calculate the expected return and standard deviation of Asset D. Is it

preferred to Assets A, B and C? Explain your answer.

3 Eu*]-(*) i=1