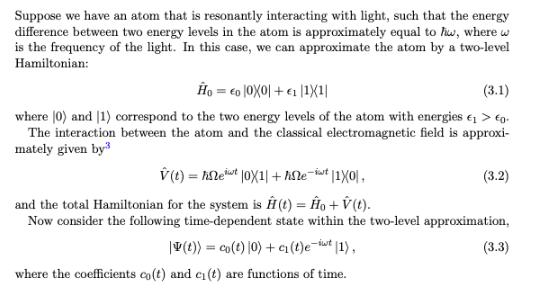
Suppose we have an atom that is resonantly interacting with light, such that the energy difference between two energy levels in the atom is approximately equal to liw, where w is the frequency of the light. In this case, we can approximate the atom by a two-level Hamiltonian: H = co (0X0| +e1 |1x1| (3.1) where (0) and |1) correspond to the two energy levels of the atom with energies e > o. The interaction between the atom and the classical electromagnetic field is approxi- mately given by (3.2) and the total Hamiltonian for the system is H(t) = lo + V (t). Now consider the following time-dependent state within the two-level approximation, |(1)) = co(t) |0) + c(t)e-it 1), -wt (3.3) where the coefficients co(t) and ca (t) are functions of time. (a) Using the Schrdinger equation, ih (t)) = (t) |4()) (3.4) show that the coefficients satisfy the matrix equation a (co(t) "at (1(t), (3.5) An 1 - hw, i.e., they evolve under a Hamiltonian that is time independent. (b) For the resonance condition hw = e1 - co, show that the solution to Eq. (3.5) is =e-icot/h( cos(SNt) -i sin(t) (co(0) -i sin(2) cos(2t) ) (o) co(t) (3.6)