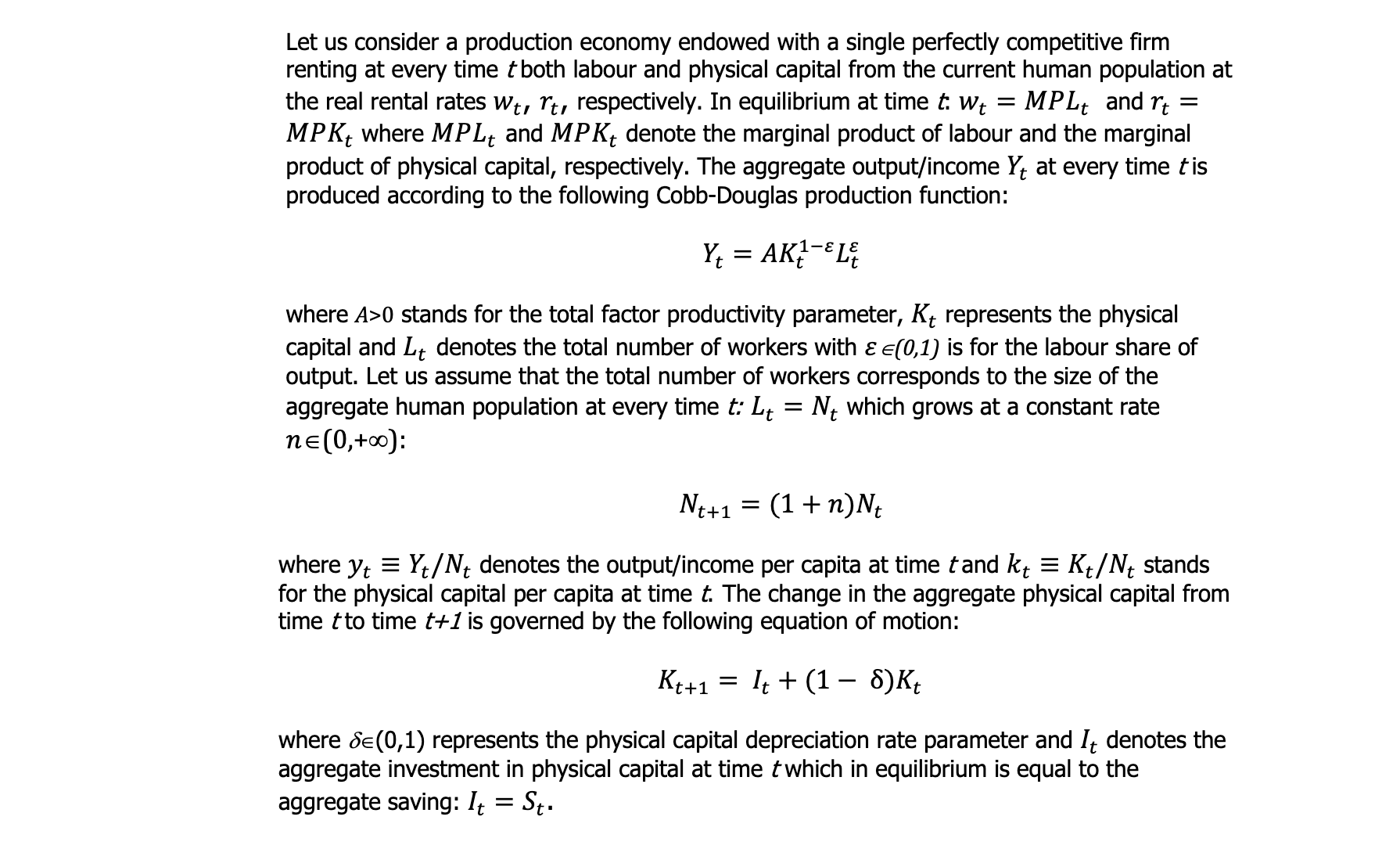
t Let us consider a production economy endowed with a single perfectly competitive firm renting at every time t both labour and physical capital from the current human population at the real rental rates wt, rt, respectively. In equilibrium at time t. W = MPL and rt = MPKt where MPL and MPK denote the marginal product of labour and the marginal product of physical capital, respectively. The aggregate output/income Y at every time t is produced according to the following Cobb-Douglas production function: Y = AK-LE where A>0 stands for the total factor productivity parameter, Kt represents the physical capital and Lt denotes the total number of workers with (0,1) is for the labour share of output. Let us assume that the total number of workers corresponds to the size of the aggregate human population at every time t: Lt = Nt which grows at a constant rate n=(0,+): Nt+1 = (1+n) Nt where yt = Yt/Nt denotes the output/income per capita at time tand k = K/Nt stands for the physical capital per capita at time t. The change in the aggregate physical capital from time to time t+1 is governed by the following equation of motion: Kt+1 = It + (1 8)Kt - where Se(0,1) represents the physical capital depreciation rate parameter and It denotes the aggregate investment in physical capital at time t which in equilibrium is equal to the aggregate saving: It = St. = d. If all the labour income is saved: St w+Lt at time t, then write-down the equilibrium equation of motion for the physical capital per capita from time to time t+1. If all the labour income is saved at every period, then derive the steady-state physical capital per capita and the steady-state output/income per capita. (15 points) e. If all the physical capital income is saved: St = rK at time t, then write-down the equilibrium equation of motion for the physical capital per capita from time to time t+1. If all the capital income is saved at every period, then derive the steady-state physical capital per capita and the steady-state output/income per capita. (15 points) f. Derive the steady-state consumption per capita associated with the steady-state solutions found in d. and e. Which of the two steady-state solutions has the highest steady-state consumption per capita? (10 points)