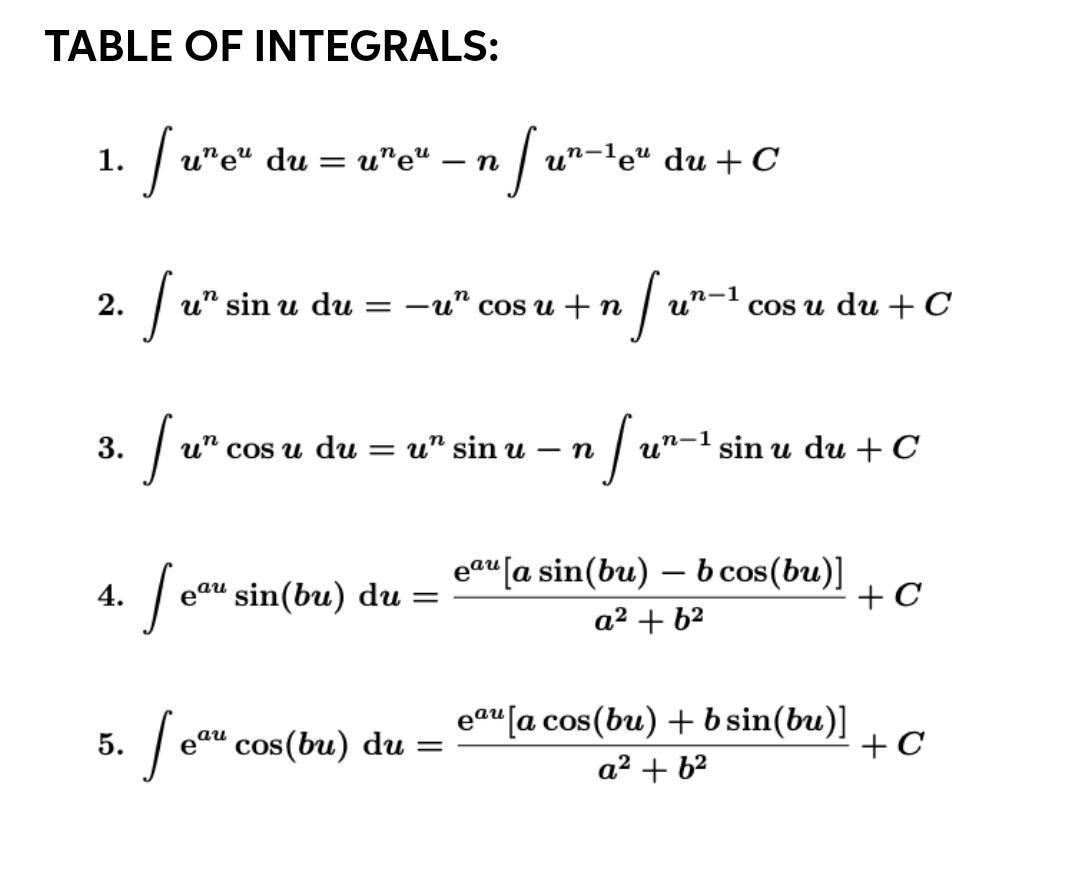
TABLE OF INTEGRALS: 1. 2. 3. [u 4. une" du une" 5. [u - n n [un-1 [eu sin(bu) du = au un sin u du =-un cos u+n un-leu du + C [eau cos(bu) du = un cos u du = u" sinu - n Sun-1 un-1 cos u du + C 2 / 1-1 un-1 sin u du + C eu[a sin(bu) b cos(bu)] +C a + b eau [a cos(bu) + b sin(bu)] a +6 + C Show clearly the steps you have taken and state the table entry number you use. Also, please show the open and closed intervals for part b when graphing. Thank you. 4. (a) Find f(t) = L-{(1+0=40) } . {. (b) Let f(t) = (2 t)h(t 2) (3 t)h(t 3) + t h(t 4). Graph f(t) for 0 t 5. Clearly explain how you obtained your graph. Common Laplace transforms (the ones we will use from Table 5.1) n! gn+1 2. L{t"} = 4. L{sin (wt)}: 6. C{sinh(wt)} 1. L{h(t)}: = 1 S n = 0, 1,... s + w 82 3. L{eot}: (4) Antiderivative: {[(7) dr} = F(8) 8 = 1 s-a 5. L{cos(wt)} 7. L{cosh (wt)} (5) n-th derivative: L{f(n) (t)} = 8"F(s) 8- f (0) 2-1 - 14. L{h(ta)}: S s +6 Five operational properties for Laplace transforms F(s) = L{f(t)} (1) Linearity: L{cf(t) + cf2(t)} = cL{f(t)} + CL{f2(t)} (2) First shift theorem: L{eat f(t)} = F(sa) (3) Second shift theorem: L{f(ta)h(ta)} = e-as F(s) 2 82 8 e-as 8 f(n-1) (0) n = 1, 2,... n = 1: L{f'(t)} = sF(s) sf(0) = sF(s) - f (0) n = 2: L{f"(t)} = sF(s) sf (0) - sf'(0) = sF(s) - sf (0) - f'(0)