Task: With the help of regression analysis, we want to find out if several indicators can explain how long it will take to repay credit as a firm. In the file found under

https://www.dropbox.com/s/rmdaaghc6lv44yl/Final.XLSX?dl=0

there are five variables, and each line represents a firm. "Payment Due" signifies how long it took the firm to pay back the debt the firm owes. "AG-Class" is a variable that says whether the firm is on the stock exchange or not. "RE-Score" shows how much debt was accumulated. "Company Size" gives the number of employees of the firm, and "Rating", quite simply, is a firm's rating (higher is better).

We want to find out whether Payment Due can be explained by the other four variables.

a) What are the dependent and independent variables? Write down on what scales each variable is measured (numeric, discrete, etc.). Then open the dataset and load it into Excel.

b)Run a full regression (with all variables). The output gives you a table with the header "ANOVA". What does this table tell us in this case? Give a statement mentioning the p-value.

c) Which of the coefficients are more meaningful, and which are less meaningful? Make statements how the dependent variable seems to be influenced by the independent variables according to the analysis so far (i.e. interpret the coefficients correctly).

d) Write down the regression equation Y= ? + ?₁X₁ + ... + ?_k X_k.

e) Now drop RE-Score from the regression. Which model is better, and how can you tell?

2) Is company size higher for firms that are trading on the stock exchange?

a) Formulate appropriate Null and Alternative Hypotheses.

b) State which test you will use, test this, and give a p-value.

c) Make a statement about the interpretation of the test.

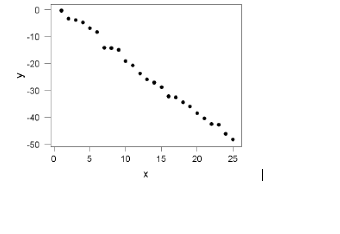
3) Below is a scatterplot of data for x and y.

a) If you would run a simple regression, which value for R squared would be the most likely?

i) 0,05 ii) 0,4 iii) 0,6 iv) 0,95

b) The resulting regression line can be used for many purposes, but not for:

i) estimating the average value of y at a specified value of x

ii) predicting the value of Y for an individual give that individual's x

iii) estimating the change in y for a one-unit change in x

iv) determining whether a change in x causes a change in y