THanks in advance.

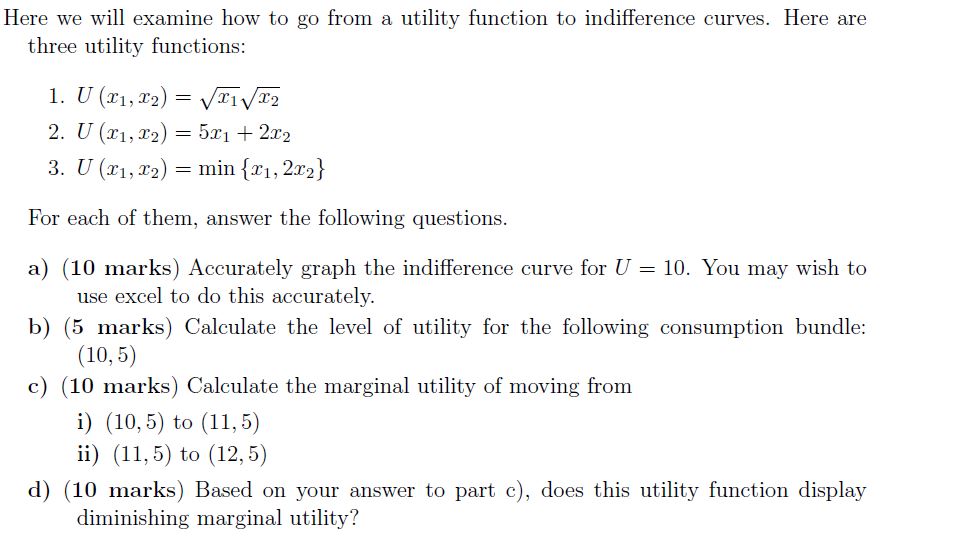
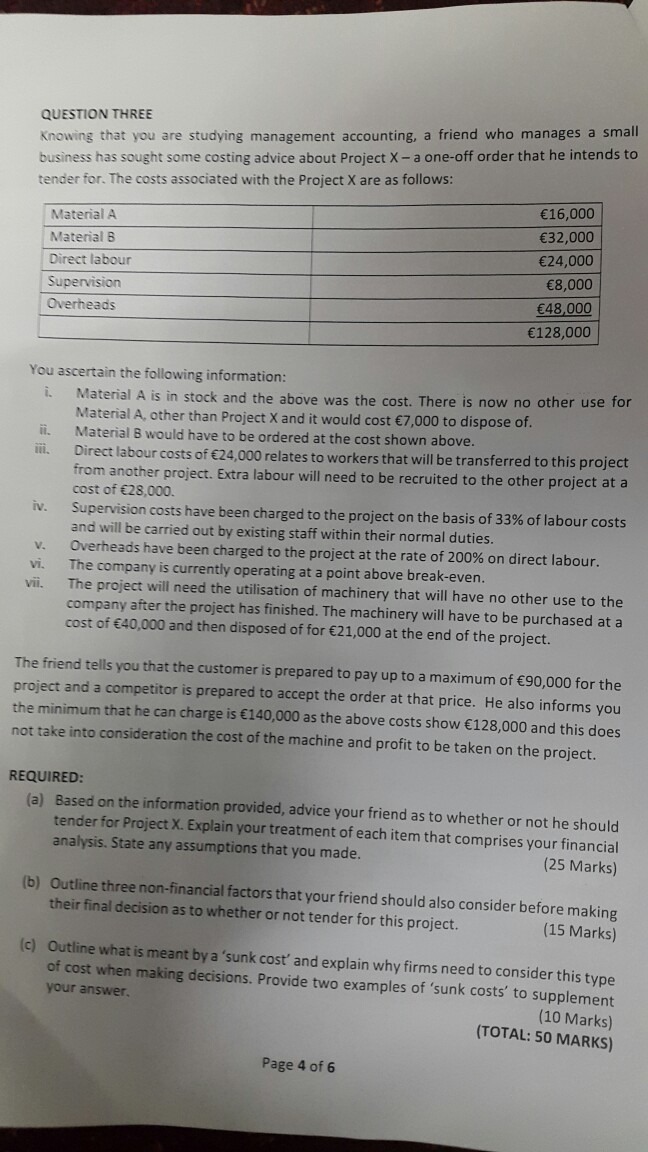
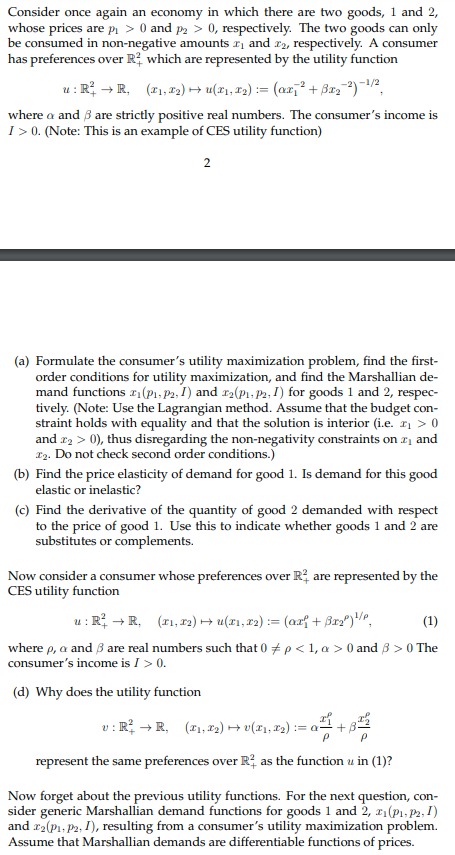
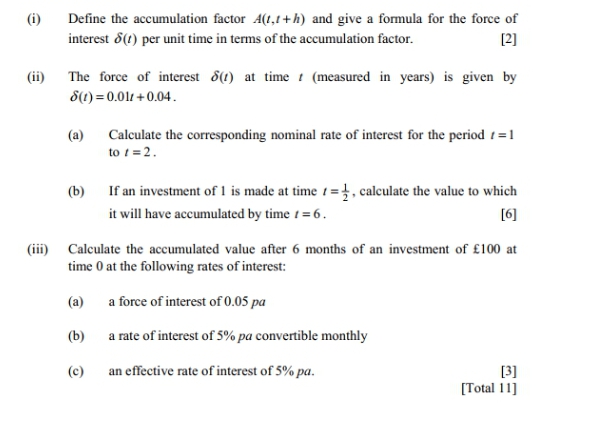
Here we will examine how to go from a utility function to indifference curves. Here are three utility functions: 1- Ulirlaml = x/Ilx/I 2. U (931, 1'2] = 5131 + 2172 3. U(:r1,$2] = min {$1,2I2} For each of them1 answer the following questions. a) ( 10 marks) Accurately graph the indifference curve for U = 10. You may wish to use excel to do this accurately. b) (5 marks] Calculate the level of utility for the following consumption bundle: (10,5) c) (10 marks) Calculate the marginal utility of moving from i) (10,5) to (11,5) ii) (11,5) to (12,5) d) (10 marks) Based on your answer to part c), does this utility function display diminishing marginal utility? QUESTION THREE Knowing that you are studying management accounting, a friend who manages a small business has sought some costing advice about Project X - a one-off order that he intends to tender for. The costs associated with the Project X are as follows: E16,000 Material A E32,000 Material B E24,000 Direct labour 68,000 Supervision E48,000 Overheads (128,000 You ascertain the following information: i. Material A is in stock and the above was the cost. There is now no other use for Material A, other than Project X and it would cost $7,000 to dispose of. ii. Material B would have to be ordered at the cost shown above. iti. Direct labour costs of $24,000 relates to workers that will be transferred to this project from another project. Extra labour will need to be recruited to the other project at a cost of $28,000. iv. Supervision costs have been charged to the project on the basis of 33% of labour costs and will be carried out by existing staff within their normal duties. V. Overheads have been charged to the project at the rate of 200% on direct labour. vi. The company is currently operating at a point above break-even. vii. The project will need the utilisation of machinery that will have no other use to the company after the project has finished. The machinery will have to be purchased at a cost of (40,000 and then disposed of for E21,000 at the end of the project. The friend tells you that the customer is prepared to pay up to a maximum of $90,000 for the project and a competitor is prepared to accept the order at that price. He also informs you the minimum that he can charge is (140,000 as the above costs show E128,000 and this does not take into consideration the cost of the machine and profit to be taken on the project. REQUIRED: (a) Based on the information provided, advice your friend as to whether or not he should tender for Project X. Explain your treatment of each item that comprises your financial analysis. State any assumptions that you made. (25 Marks) (b) Outline three non-financial factors that your friend should also consider before making their final decision as to whether or not tender for this project. (15 Marks) (c) Outline what is meant by a 'sunk cost' and explain why firms need to consider this type of cost when making decisions. Provide two examples of 'sunk costs' to supplement your answer. (10 Marks) (TOTAL: 50 MARKS) Page 4 of 6Consider once again an economy in which there are two goods, 1 and 2, whose prices are p > 0 and py > 0, respectively. The two goods can only be consumed in non-negative amounts r, and r2, respectively. A consumer has preferences over R, which are represented by the utility function 1 : R- - R, (21, 12) - 4(21, 12) := (or, ' + 812 ?) where a and 8 are strictly positive real numbers. The consumer's income is I > 0. (Note: This is an example of CES utility function) 2 (a) Formulate the consumer's utility maximization problem, find the first- order conditions for utility maximization, and find the Marshallian de- mand functions ,(p1, p2, /) and ra(p1, p2, /) for goods 1 and 2, respec- tively. (Note: Use the Lagrangian method. Assume that the budget con- straint holds with equality and that the solution is interior (i.e. r] > 0 and x2 > 0), thus disregarding the non-negativity constraints on a and 12. Do not check second order conditions.) (b) Find the price elasticity of demand for good 1. Is demand for this good elastic or inelastic? (c) Find the derivative of the quantity of good 2 demanded with respect to the price of good 1. Use this to indicate whether goods 1 and 2 are substitutes or complements. Now consider a consumer whose preferences over R4 are represented by the CES utility function 1 : R. - R. (11, 12) - 4(21, x2) := (arf + Brzo)/e, (1) where p, a and S are real numbers such that 0 # p 0 and # > 0) The consumer's income is / > 0. (d) Why does the utility function U: R -R. (21, 12) 4 0(21.12) :=0 represent the same preferences over R. as the function u in (1)? Now forget about the previous utility functions. For the next question, con- sider generic Marshallian demand functions for goods 1 and 2, z,(p1, p2, !) and r2(pi, p2, I), resulting from a consumer's utility maximization problem. Assume that Marshallian demands are differentiable functions of prices.2. A production function is said to be homogeneous of degree gif f(IK, AL) = > f(K, L) . where x is a positive constant. That is, the production function has the same returns to scale for every combination of inputs. For such a production function, show that the marginal product of labour and marginal product of capital functions are homogeneous of degree g - 1.(i) Define the accumulation factor A(t,/+h) and give a formula for the force of interest 6(t) per unit time in terms of the accumulation factor. [2] (ii) The force of interest 6() at time r (measured in years) is given by 5(1) =0.01/ +0.04. (a) Calculate the corresponding nominal rate of interest for the period / = 1 to 1 = 2. (b) If an investment of 1 is made at time /=, calculate the value to which it will have accumulated by time r =6. [6] (iii) Calculate the accumulated value after 6 months of an investment of $100 at time 0 at the following rates of interest: (a) a force of interest of 0.05 pa (b) a rate of interest of 5% pa convertible monthly (c) an effective rate of interest of 5% pa. [3] [Total 1 1](i) Define the accumulation factor A(t,/+h) and give a formula for the force of interest 6(t) per unit time in terms of the accumulation factor. [2] (ii) The force of interest 6() at time r (measured in years) is given by 5(1) =0.01/ +0.04. (a) Calculate the corresponding nominal rate of interest for the period / = 1 to 1 = 2. (b) If an investment of 1 is made at time /=, calculate the value to which it will have accumulated by time r =6. [6] (iii) Calculate the accumulated value after 6 months of an investment of $100 at time 0 at the following rates of interest: (a) a force of interest of 0.05 pa (b) a rate of interest of 5% pa convertible monthly (c) an effective rate of interest of 5% pa. [3] [Total 1 1]