Question: Consider the quadratic form f(x) = x + x3 + x3 + 4x1X2 + 4x2x3 + 4x3x1. (a) Find the real symmetric matrix A

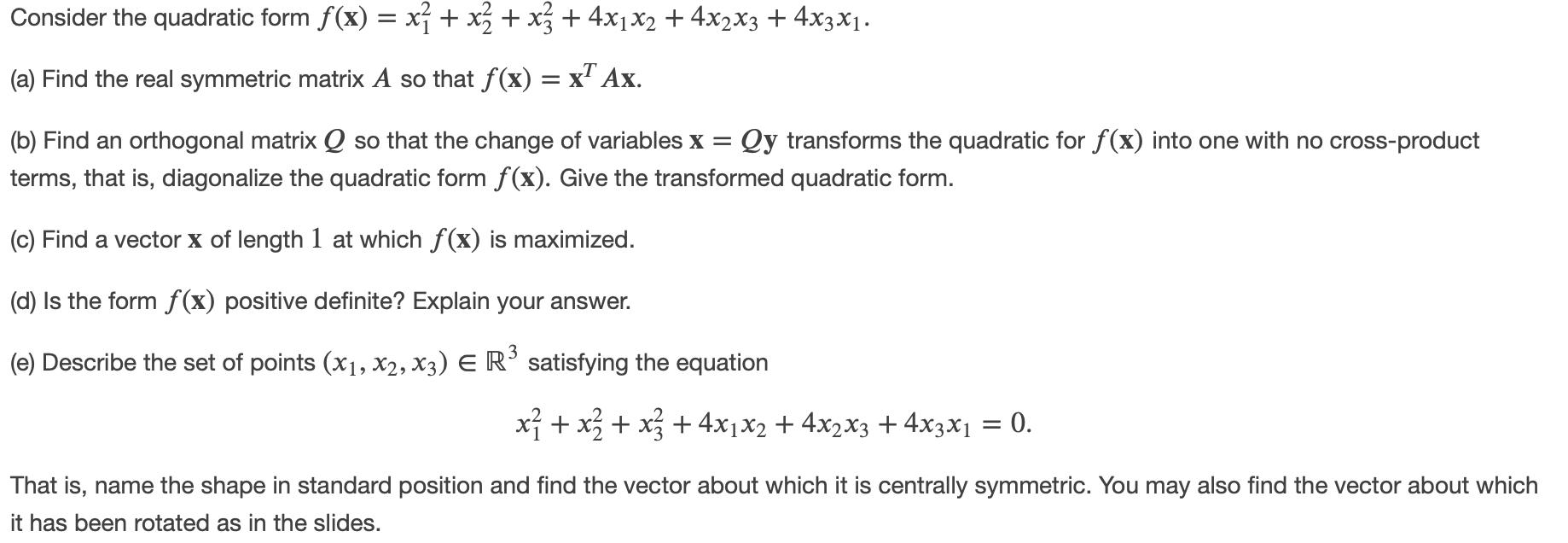
Consider the quadratic form f(x) = x} + x2 + xz + 4x1x2 + 4x2x3 + 4x3x1. (a) Find the real symmetric matrix A so that f(x) =

Consider the quadratic form f(x) = x + x3 + x3 + 4x1X2 + 4x2x3 + 4x3x1. (a) Find the real symmetric matrix A so that f(x) = x' Ax. (b) Find an orthogonal matrix Q so that the change of variables x = Qy transforms the quadratic for f(x) into one with no cross-product terms, that is, diagonalize the quadratic form f(x). Give the transformed quadratic form. (c) Find a vector x of length 1 at which f(x) is maximized. (d) Is the form f(x) positive definite? Explain your answer. (e) Describe the set of points (x1, X2, X3) E R satisfying the equation x + x3 + x3 + 4x1x2 + 4x2x3 + 4x3X1 = 0. That is, name the shape in standard position and find the vector about which it is centrally symmetric. You may also find the vector about which it has been rotated as in the slides.

Step by Step Solution

★★★★★

3.34 Rating (160 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

Given that O2 22 2 42x 4 432 3 2 2 ... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

Leggere, an Internet book retailer, is interested in better understanding the purchase decisions of its customers. For a set of 2,000 customer transactions, it has categorized the individual book...

In each case, find a symmetric matrix A such that q = XTBX takes the form q = XTAX. (a) (b) (c) (d) 2 101 011 103 212 145

In each case, find a symmetric matrix A such that (v, w) = vTAw. (a) (b) (c) (d) +5vzw = vy 2 + 2vy w, + 2vyw

In Figure, a block slides down an incline. As it moves from point A to point-B, which are 5.0 m apart, force F acts on the block, with magnitude 2.0 N and directed down the incline. The magnitude of...

Following the lead design an optimum 2- bit quantizer for a signal whose samples follow a triangular PDF, (a) Find the four quantization levels, { y1 ,y2 ,y3 ,y4}, and the three boundary points, {...

Use Newtons method with initial approximation x1 = 1 to find x2, the second approximation to the root of the equation x3 + x + 3 = 0. Explain how the method works by first graphing the function and...

(Limited Information Maximum Likelihood Estimation) Consider a bivariate distribution for x and y that is a function of two parameters, and . The joint density is f (x, y | , ). We consider maximum...

In this problem, you are to show that the generalized form of Ampres law (Equation 32-4) and the Biot Savart law give the same result in a situation in which they both can be used. Figure shows two...

Consider the following problem. Minimize Z = 2x1 + 3x2 + 2x3, Subject to And x1 ¥ 0, x2 ¥ 0, x3 ¥ 0. Let x4 and x6 be the surplus variables for the first and second constraints,...

Suppose X (t) is a Weiner process with diffusion parameter = 1 as described in Section 8.5. (a) Write the joint PDF of X1 = X (t1) and X2 = X (t2) for t2

In this exercise we review some basics of attitudes toward risk when tastes are state-independent and, in part B, we also verify some of the numbers that appear in the graphs of part A of the chapter...

The demand curve for a product is given by Q x = 1,000 2Px + .02Pz, where Pz = $400. a. What is the own price elasticity of demand when Px = $154? Is demand elastic or inelastic at this price? What...

One common way to test for authenticity of data is to analyze the frequencies of digits. When people are weighed and their weights are rounded to the nearest pound, we expect the last digits 0, 1, 2,...

Final Exam 1 2 of 1 7 This test: 1 0 0 point ( s ) possible n list This question: 6 point ( s ) poss Integrate f ( x , y , z ) = x y z x 2 y 2 z 2 over the path f r ( t ) = 3 t i 3 t j 2 t k , 0 6...

In the Olympiad of 708B.C, some athletes competing in the standing long jump used handheld weights called halters to lengthen their jumps (Figure). The weights were swung up in front just before...

Jumping up before the elevator hits after the cable snaps and the safety system fails, an elevator cab free-falls from a height of 36 m. During the collision at the bottom of the elevator shaft, a 90...

In Figure ?a? both batteries have emf ? = 1.20 V and the external resistance R is a variable resistor. Figure ?b? gives the electric potentials 7 between the terminals of each battery as functions of...

Shown is the graph of the population function P(t) for yeast cells in a laboratory culture. Use the method of Example 1 to graph the derivative P'(t). What does the graph of P' tell us about the...

Knowing that at the instant shown rod AB has a constant angular velocity of 15 rad/s counterclockwise, determine (a) The angular acceleration of rod DE, (b) The acceleration of point D. 4.5.- -5m la...

Solve ECO2-06 20259:Introductory Financial Accounting I nal Exam Review Quiz 6 of 36 An error in the reported inventory will cause errors in all of the following EXCEPT the: list K O A. Statement of...

Write the quadratic function f (x) = ax2 + bx + c in standard form to verify that the vertex occurs at ( -b/2a, f(-b/2a)).

Mixture distribution suppose that the joint distribution of the two random variables x and y is a. Find the maximum likelihood estimators of and and their asymptotic joint distribution. b. Find the...

Consider the following function: f (x) = 48x 60x2 + x3. (a) Use the first and second derivatives to find the local maxima and local minima of f (x). (b) Use the first and second derivatives to show...

A certain random variable has a probability density function of the form f x (x) = ce 2xu(x). Find the following: (a) The constant c, (b) Pr (X >2), (c) Pr (X 2).

Repeat Exercise 3.13 using the PDF. A certain random variable has a probability density function of the form f x (x) = ce 2xu(x). Find the following: (a) The constant c, (b) Pr (X >2), (c) Pr (X

Consider the QHIC data in Figure 13.21 (page 556). When we performed a regression analysis of these data by using the simple linear regression model, plots of the model's residuals versus x (home...