The annual benefit for the following project is potentially: $80,000 with the probability of 65%, $65,000 with the probability of 20%, or $100,000. It is estimated that a life of 6 years is twice as likely compared to a life of 9 years for this project. Assume, the initial/first cost of this project is $500,000. The firm uses an interest rate of 6% APR to finance their projects. Assume, the probability distributions for annual benefit and life of the project are unrelated or statistically independent. Calculate the probability distribution for present worth of the project. Find Expected value of the present worth and present worth of expected value. Find PWs standard deviation.

Note: Please do on Excel step by step and write down formulae used. As an example picture attached.

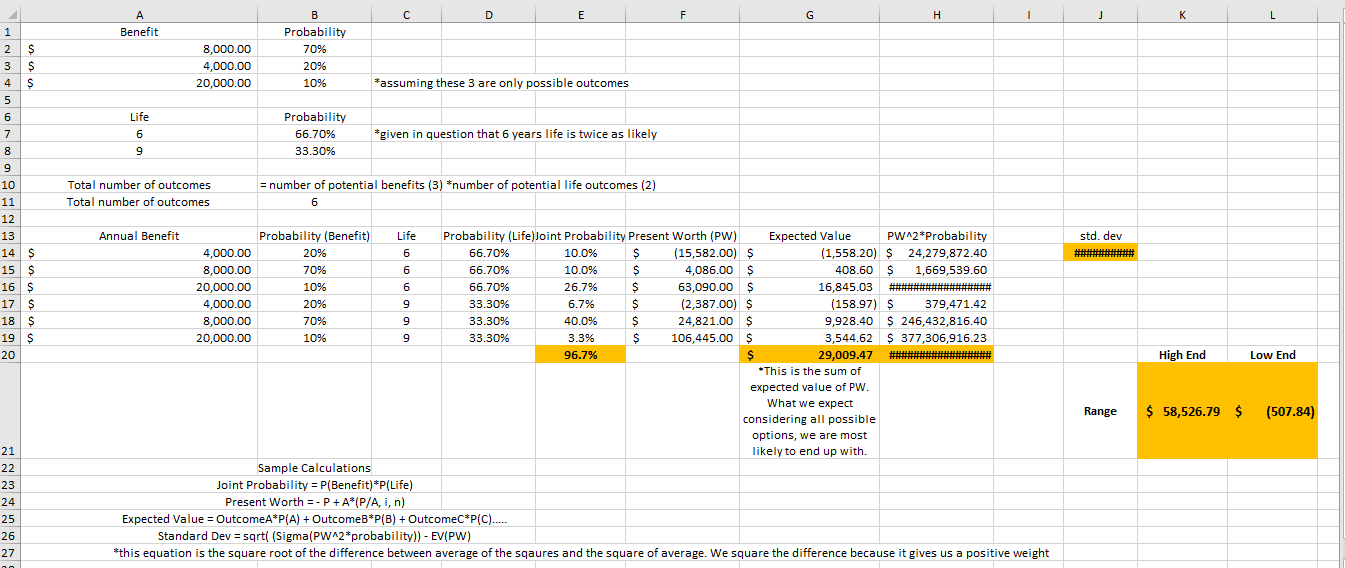One thing should be clear there is mistake in above example so expert will solved subject Problem without any mistake.

D E F G H J K L Benefit 8,000.00 4,000.00 20,000.00 B Probability 70% 20% 10% *assuming these 3 are only possible outcomes Life Probability 66.70% 33.30% 6 9 9 *given in question that 6 years life is twice as likely 1 2 $ 3 $ 4 $ 5 6 7 8 9 9 10 11 12 13 14 S 15 $ 16 $ 17 $ 18 $ 19 $ 20 Total number of outcomes Total number of outcomes = number of potential benefits (3) *number of potential life outcomes (2) 6 std. dev High End Low End Annual Benefit Probability (Benefit) Life Probability (Life)Joint Probability Present Worth (PW) Expected Value PW^2*Probability 4,000.00 20% 6 66.70% 10.0% $ (15,582.00) S (1,558.20) $ 24,279,872.40 8,000.00 70% 6 66.70% 10.0% $ 4,086.00 $ 408.60 $ 1.669.539.60 20,000.00 10% 6 66.70% 26.7% $ 63,090.00 $ 16,845.03 4,000.00 20% 9 33.30% 6.7% $ (2,387.00) $ (158.97) $ 379,471.42 8,000.00 70% 9 33.30% 40.0% $ 24,821.00 $ 9,928.40 $ 246,432,816.40 20,000.00 10% 9 33.30% 3.3% $ 106,445.00 $ 3,544.62 $ 377,306,916.23 96.7% $ 29,009.47 ##***************######## This is the sum of expected value of PW. What we expect considering all possible options, we are most likely to end up with. Sample Calculations Joint Probability = P(Benefit)*P(Life) Present Worth = -P+A (P/A, I, n) Expected Value = OutcomeA*P(A) + OutcomeB*P(B) + OutcomeC*P(C)..... Standard Dev = sqrt((Sigma(PW^2*probability)) - EV(PW) *this equation is the square root of the difference between average of the sqaures and the square of average. We square the difference because it gives us a positive weight Range $ 58,526.79 $ (507.84) 21 22 23 24 25 26 27 D E F G H J K L Benefit 8,000.00 4,000.00 20,000.00 B Probability 70% 20% 10% *assuming these 3 are only possible outcomes Life Probability 66.70% 33.30% 6 9 9 *given in question that 6 years life is twice as likely 1 2 $ 3 $ 4 $ 5 6 7 8 9 9 10 11 12 13 14 S 15 $ 16 $ 17 $ 18 $ 19 $ 20 Total number of outcomes Total number of outcomes = number of potential benefits (3) *number of potential life outcomes (2) 6 std. dev High End Low End Annual Benefit Probability (Benefit) Life Probability (Life)Joint Probability Present Worth (PW) Expected Value PW^2*Probability 4,000.00 20% 6 66.70% 10.0% $ (15,582.00) S (1,558.20) $ 24,279,872.40 8,000.00 70% 6 66.70% 10.0% $ 4,086.00 $ 408.60 $ 1.669.539.60 20,000.00 10% 6 66.70% 26.7% $ 63,090.00 $ 16,845.03 4,000.00 20% 9 33.30% 6.7% $ (2,387.00) $ (158.97) $ 379,471.42 8,000.00 70% 9 33.30% 40.0% $ 24,821.00 $ 9,928.40 $ 246,432,816.40 20,000.00 10% 9 33.30% 3.3% $ 106,445.00 $ 3,544.62 $ 377,306,916.23 96.7% $ 29,009.47 ##***************######## This is the sum of expected value of PW. What we expect considering all possible options, we are most likely to end up with. Sample Calculations Joint Probability = P(Benefit)*P(Life) Present Worth = -P+A (P/A, I, n) Expected Value = OutcomeA*P(A) + OutcomeB*P(B) + OutcomeC*P(C)..... Standard Dev = sqrt((Sigma(PW^2*probability)) - EV(PW) *this equation is the square root of the difference between average of the sqaures and the square of average. We square the difference because it gives us a positive weight Range $ 58,526.79 $ (507.84) 21 22 23 24 25 26 27