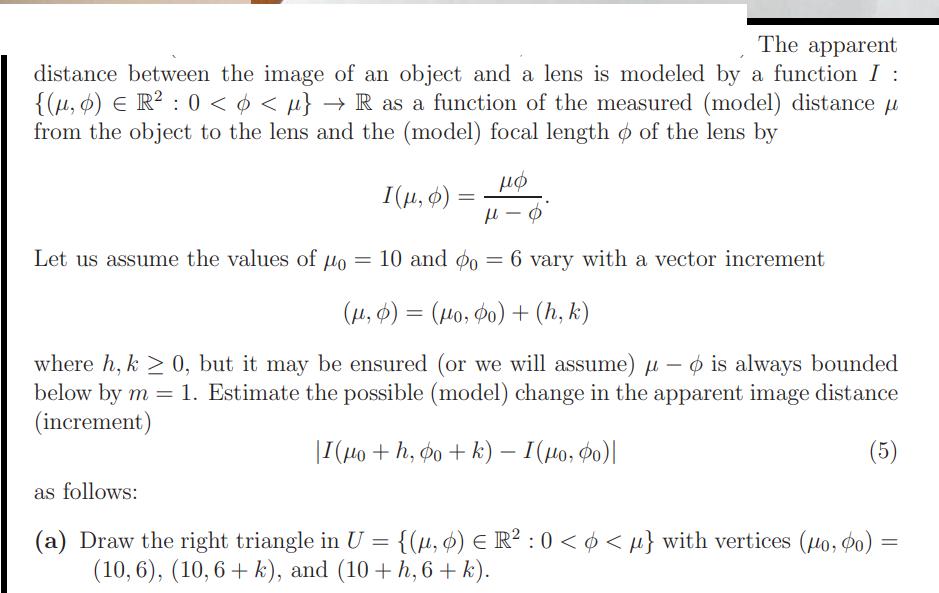
The apparent distance between the image of an object and a lens is modeled by a function I: {(1, 0) R : 0 < < } R as a function of the measured (model) distance from the object to the lens and the (model) focal length of the lens by Let us assume the values of po = I (, 0) = 10 and do l = 6 vary with a vector increment (,0) = (0,00) + (h, k) where h, k 0, but it may be ensured (or we will assume) -o is always bounded below by m = 1. Estimate the possible (model) change in the apparent image distance (increment) I(o+h, do + k) I (o, Po)| (5) as follows: (a) Draw the right triangle in U = {(u, o) R : 0 < < } with vertices (o, do) = } 0) (10, 6), (10,6+k), and (10+h, 6+k). (b) Use the triangle inequality to show the increment in (5) is bounded above by the sum of the increments and I(Mo+h, do + k) - I(Mo, o + k)| \I(po, o+k) I(po, o)]. (c) Express the increment in (7) in the form f(k) - f(0)| for an appropriate choice of fe C(0, k) nC[0, k], and then use the mean value theorem applied to f to get an estimate of the form \I(o,o+k) I(zo,o)\