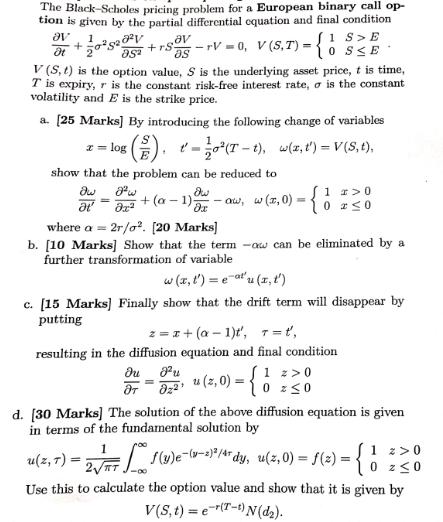
The Black-Scholes pricing problem for a European binary call op- tion is given by the partial differential equation and final condition 252 V av 1 t +20 J1 S>E +rS -V-0, V(S.T) = 0 SE sav as 85 v (S,t) is the option value, 5 is the underlying asset price, t is time, T is expiry, r is the constant risk-free interest rate, o is the constant volatility and E is the strike price. a. [25 Marks] By introducing the following change of variables x = log (2). - 1/0 (T-1), w(x, t') = V(S, t), show that the problem can be reduced to dw aw 02 = +(a-1); t x -aw, w (20) = { 1 230 0 0 where a = - 2r/o. [20 Marks] b. [10 Marks] Show that the term -ow can be eliminated by a further transformation of variable w (x, t')=eatu (x, t') c. [15 Marks] Finally show that the drift term will disappear by putting z = x + (a-1)t', 7=, resulting in the diffusion equation and final condition Ju z>0 2022, (2,0)=1230 d. [30 Marks] The solution of the above diffusion equation is given in terms of the fundamental solution by poo 1 u(z, 7) = = 2/ TT 0 1 (v)e- (2-2)/4 dy, u(2,0) = (z) = { ={} 1 z>0 0 z0 Use this to calculate the option value and show that it is given by V(s, t)= e(T-t) N(dz).