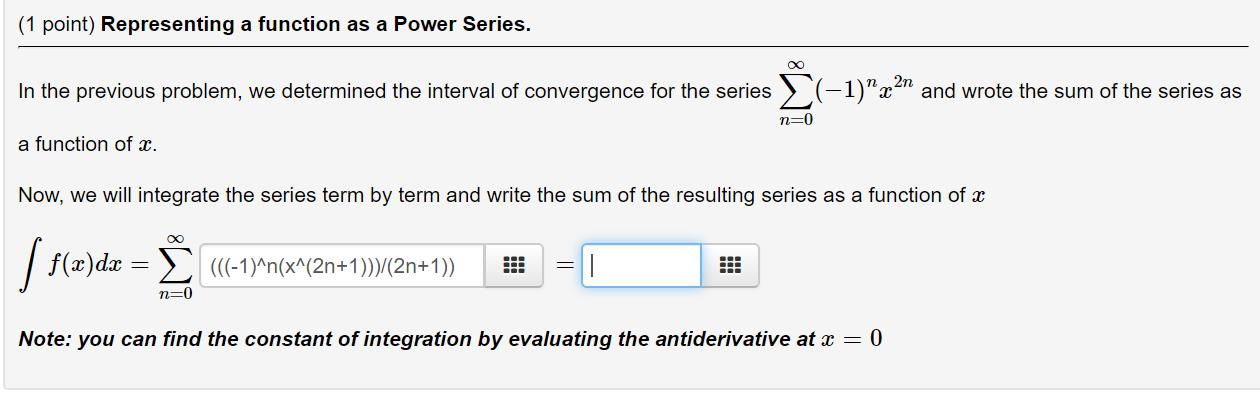
(1 point) Representing a function as a Power Series. 2n In the previous problem, we determined the interval of convergence for the series (-1)"x and wrote the sum of the series as a function of x. Now, we will integrate the series term by term and write the sum of the resulting series as a function of [ f(x)dx = (((-1)^n(x^(2n+1))/(2n+1))} n=0 = n=0 T Note: you can find the constant of integration by evaluating the antiderivative at x = 0