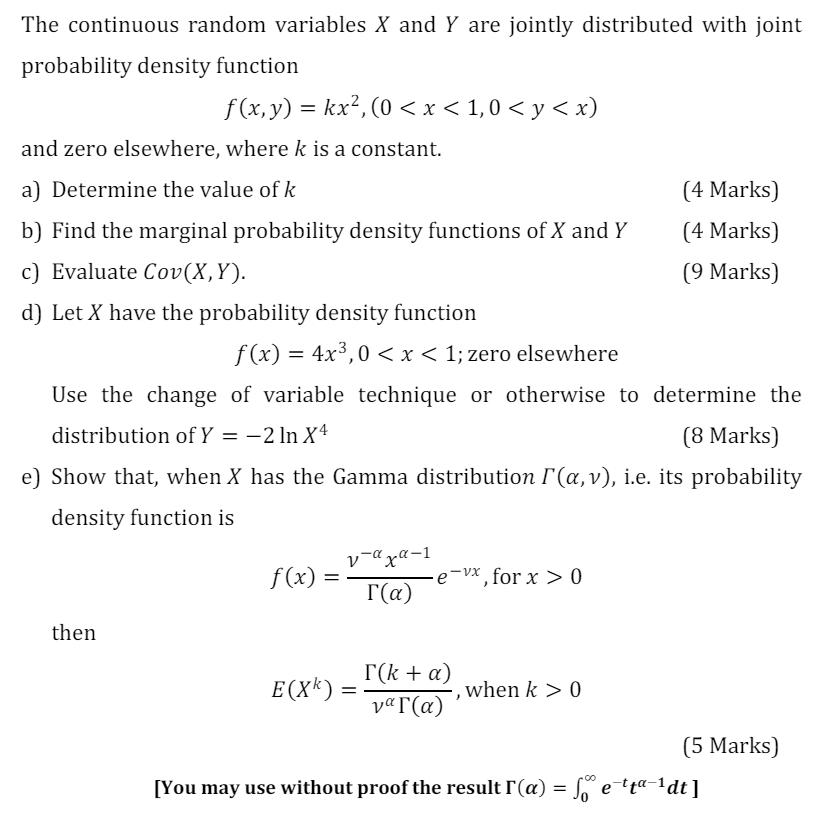
The continuous random variables X and Y are jointly distributed with joint probability density function f(x, y) = kx, (0 < x < 1, 0 < y < x) and zero elsewhere, where k is a constant. a) Determine the value of k (4 Marks) b) Find the marginal probability density functions of X and Y (4 Marks) c) Evaluate Cov(X, Y). (9 Marks) d) Let X have the probability density function f(x) = 4x,0 < x < 1; zero elsewhere Use the change of variable technique or otherwise to determine the distribution of Y = -2 In X4 (8 Marks) e) Show that, when X has the Gamma distribution (a, v), i.e. its probability density function is f(x) = -1 r(a) e-vx, for x>0 then (k + ) E(X) = when k > 0 () (5 Marks) [You may use without proof the result (a) = fo etta-dt]