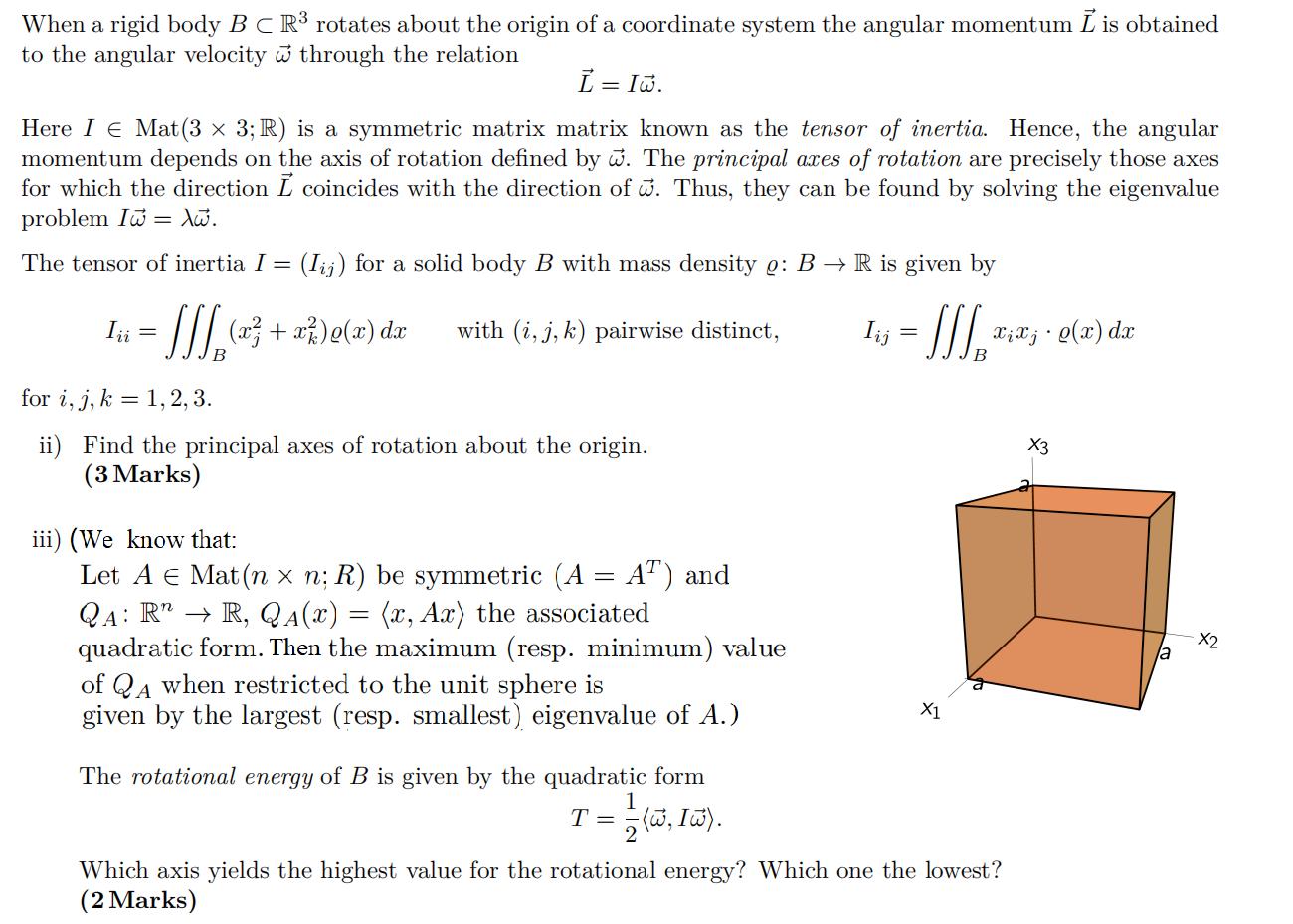
When a rigid body BC R rotates about the origin of a coordinate system the angular momentum I is obtained to the angular velocity through the relation L = Iw. Here I Mat (3 x 3; R) is a symmetric matrix matrix known as the tensor of inertia. Hence, the angular momentum depends on the axis of rotation defined by w. The principal axes of rotation are precisely those axes for which the direction I coincides with the direction of . Thus, they can be found by solving the eigenvalue problem I Xw. The tensor of inertia I = (Iij) for a solid body B with mass density o: BR is given by with (i, j, k) pairwise distinct, Iii = [[[_(x + x )o(x) dx B for i, j, k = 1, 2, 3. ii) Find the principal axes of rotation about the origin. (3 Marks) iii) (We know that: Let A Mat (n x n; R) be symmetric (A = AT) and QA: RR, QA(x) = (x, Ax) the associated quadratic form. Then the maximum (resp. minimum) value of QA when restricted to the unit sphere is given by the largest (resp. smallest) eigenvalue of A.) Which axis yields the highest value for (2 Marks) Iij = I x ; x ; - Q(x) dx X1 The rotational energy of B is given by the quadratic form T = 1/(w, Iw). rotational energy? Which one the lowest? X3 la X2