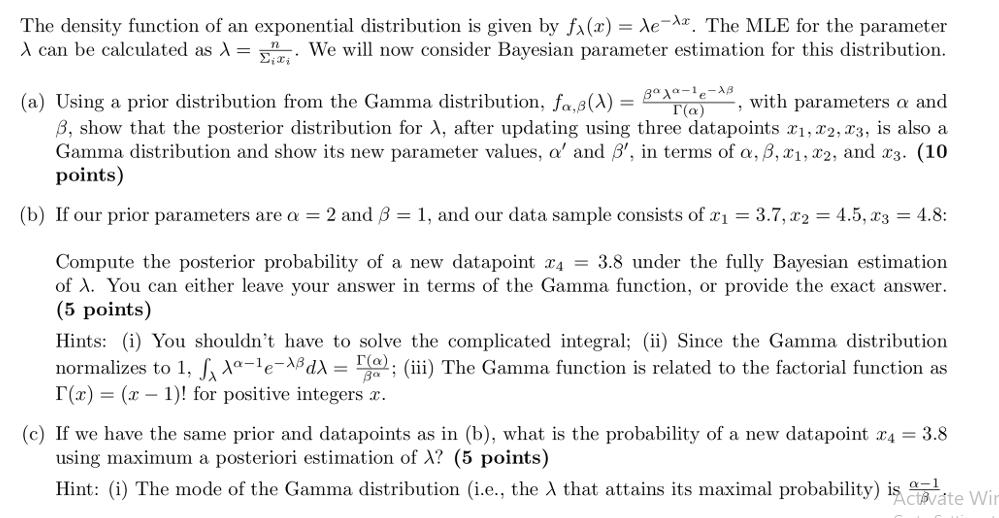
The density function of an exponential distribution is given by fx(x) = ex. The MLE for the parameter A can be calculated as = We will now consider Bayesian parameter estimation for this distribution. = Baxa-e-AB T(a) (a) Using a prior distribution from the Gamma distribution, fa,B(A) with parameters a and , show that the posterior distribution for A, after updating using three datapoints x1, x2, x3, is also a Gamma distribution and show its new parameter values, a' and B', in terms of a, b, x1, x2, and x3. (10 points) (b) If our prior parameters are a = 2 and 3 = 1, and our data sample consists of x1 = 3.7, x2 = 4.5, x3 = 4.8: Compute the posterior probability of a new datapoint x4 = 3.8 under the fully Bayesian estimation of A. You can either leave your answer in terms of the Gamma function, or provide the exact answer. (5 points) Hints: (i) You shouldn't have to solve the complicated integral; (ii) Since the Gamma distribution normalizes to 1, A-e d = (a); (iii) The Gamma function is related to the factorial function as T(x) (x 1)! for positive integers x. (c) If we have the same prior and datapoints as in (b), what is the probability of a new datapoint x4 = 3.8 using maximum a posteriori estimation of X? (5 points) Hint: (i) The mode of the Gamma distribution (i.e., the A that attains its maximal probability) is a "Activate Wi