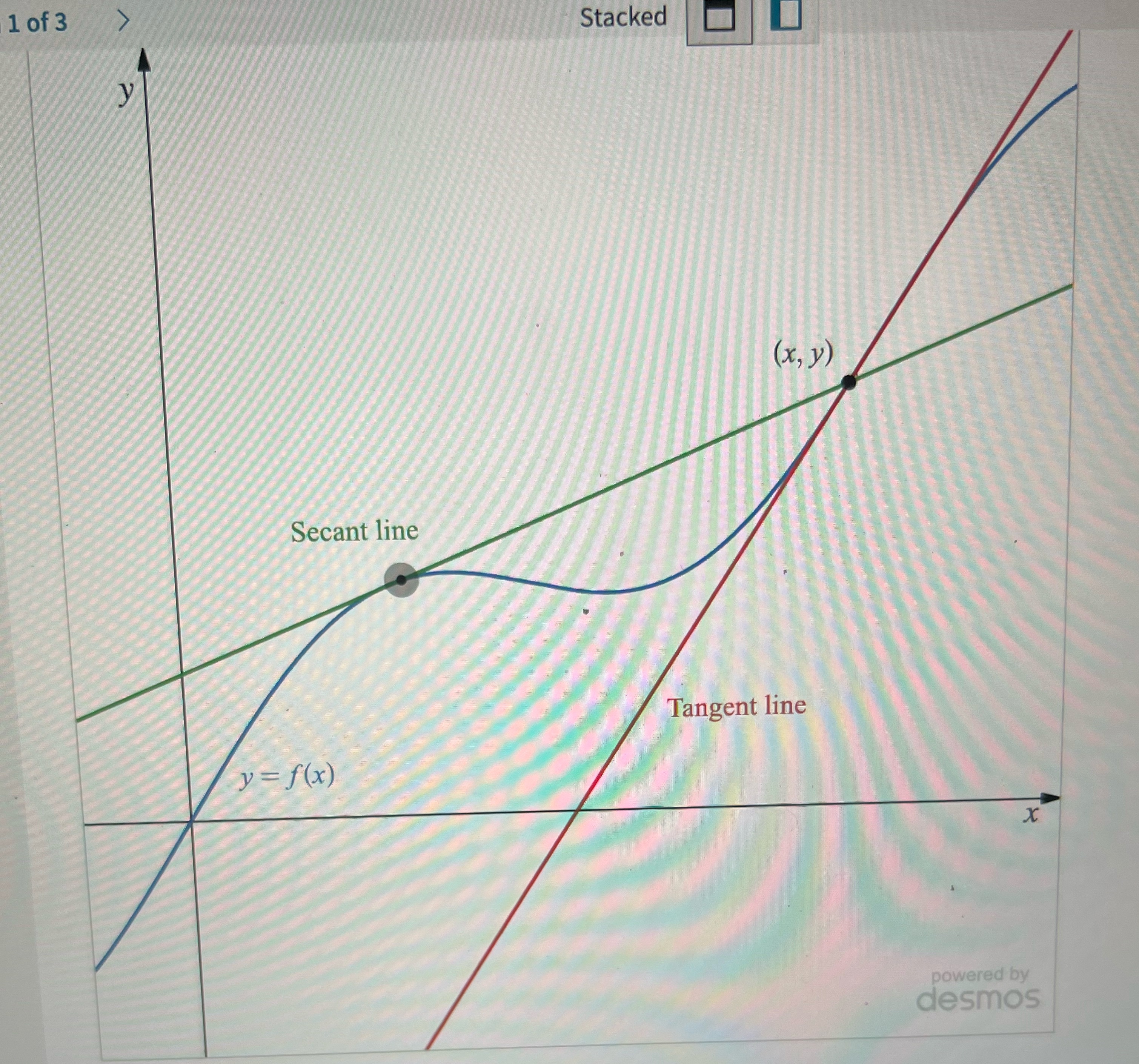
The dynamic figure shows the graphs of y = f(x) the line tangent to at coordinates (x, y) , and the secant line through (x, y) and a second point on the graph of f Drag the movable point along the graph of to explore the relationship between the secant line and the tangent line as the movable point approaches the coordinates (x, y)As the movable point approaches the coordinates (x, y) how do the slopes of the secant and tangent lines compare? Macmillan Learning The slope of the secant line decreases , approaching the slope of the tangent line. The slope of the secant line decreases, becoming less similar to the slope of the tangent line. The slope of the secant line increases, approaching the slope of the tangent line. The slope of the secant line increases, becoming less similar to the slope of the tangent line.