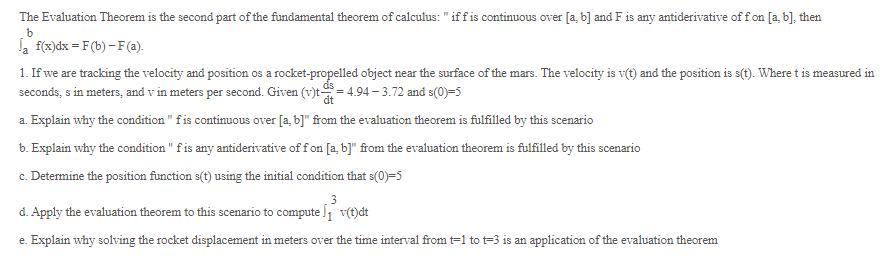
The Evaluation Theorem is the second part of the fundamental theorem of calculus: "iff is continuous over [a, b] and F is any antiderivative off on [a, b], then b Ja f(x) dx = F(b)-F (a). 1. If we are tracking the velocity and position os a rocket-propelled object near the surface of the mars. The velocity is v(t) and the position is s(t). Where t is measured in seconds, sin meters, and vin meters per second. Given (v)t=4.94-3.72 and s(0)=5 dt a. Explain why the condition " fis continuous over [a, b]" from the evaluation theorem is fulfilled by this scenario b. Explain why the condition " f is any antiderivative of fon [a, b]" from the evaluation theorem is fulfilled by this scenario c. Determine the position function s(t) using the initial condition that s(0)=5 3 d. Apply the evaluation theorem to this scenario to compute v(t)dt e. Explain why solving the rocket displacement in meters over the time interval from t=1 to t-3 is an application of the evaluation theorem