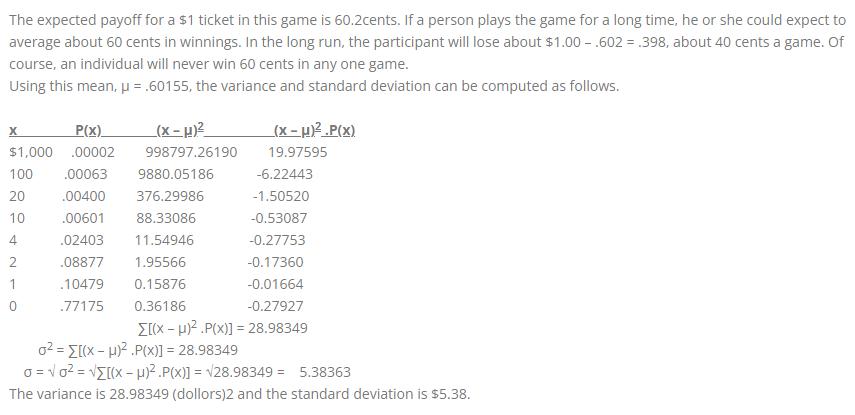
The expected payoff for a $1 ticket in this game is 60.2cents. If a person plays the game for a long time, he or she could expect to average about 60 cents in winnings. In the long run, the participant will lose about $1.00 - .602 = .398, about 40 cents a game. Of course, an individual will never win 60 cents in any one game. Using this mean, u = .60155, the variance and standard deviation can be computed as follows. P(x) (x - H)? _(x - H2.P(x) $1,000 .00002 998797.26190 19.97595 100 .00063 9880.05186 -6.22443 20 .00400 376.29986 -1.50520 10 .00601 88.33086 -0.53087 4. .02403 11.54946 -0.27753 .08877 1.95566 -0.17360 1 .10479 0.15876 -0.01664 .77175 0.36186 -0.27927 E[(X - H)2 .P(x)] = 28.98349 o2 = E[(x - H)? .P(X)] = 28.98349 O = v o2 = VE[(x - ?.P(X)] = v28.98349 = 5.38363 The variance is 28.98349 (dollors)2 and the standard deviation is $5.38.