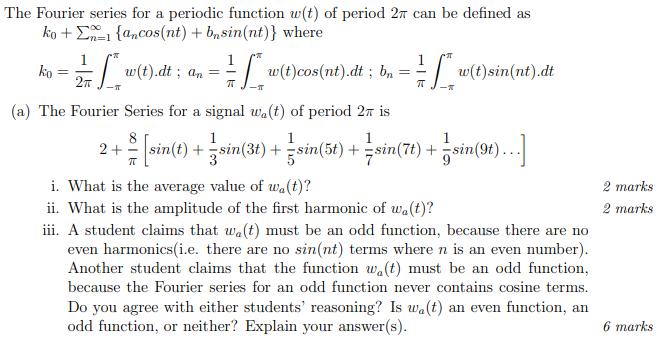
The Fourier series for a periodic function w(t) of period 27 can be defined as ko + E {ancos(nt) + bnsin(nt)} where n=1 1 ko | w(t).dt ; an w(t)cos(nt).dt ; b,n w(t)sin(nt).dt %3D -IT (a) The Fourier Series for a signal wa(t) of period 27 is 8 - sin(t) +sin(3t) +sin(5t) +sin(7t) +sin(9t). 2+ 1 1 1 1 i. What is the average value of wa(t)? ii. What is the amplitude of the first harmonic of wa(t)? iii. A student claims that wa(t) must be an odd function, because there are no even harmonics(i.e. there are no sin(nt) terms where n is an even number). Another student claims that the function w.(t) must be an odd function, 2 marks 2 marks because the Fourier series for an odd function never contains cosine terms. Do you agree with either students' reasoning? Is wa(t) an even function, an odd function, or neither? Explain your answer(s). 6 marks